Arithmetic - Mixture, Alligation, Removal & Replacement - Previous Year CAT/MBA Questions

Answer: Option D

Explanation :

Let 10 units of mixture is mixed with 30 units of sugar syrup.

Amount of sugar syrup in 10 units of mixture = 5 units
Amount of lemon juice in 10 units of mixture = 5 units

∴ Total amount of lemon juice in the final mixture = 5 units, and
Total amount of sugar syrup in the final mixture = 5 + 30 = 35 units

Ratio of lemon juice and sugar syrup in final mixture = 5 : 35 = 1 : 7.

Hence, option (d).

Answer: Option A

Explanation :

Let container A initially have 100 liters of sugar while container B have 100 liters of milk.

​​​​​​​

Now the second container has sugar and milk in the ratio of 1 : 2. 
When half i.e., 75 liters of it is transferred, 25 liters of sugar and 50 liters of milk will be transferred.

​​​​​​​

Now the first container has sugar and milk in the ratio of 3 : 2. 
When half i.e., 62.5 liters of it is transferred, 37.5 liters of sugar and 25 liters of milk will be transferred.

​​​​​​​

∴ Ratio of sugar and milk in 2nd container = 62.5 : 75 = 625 : 750 = 25 : 30 = 5 : 6

Hence, option (a).

Answer: Option D

Explanation :

When two containers contain equal amount of milk and water respectively and then equal amounts are transferred from 1^st to 2^nd and then from 2^nd to 1^st.

The amount of milk in 1^st becomes equal to amount of water in 2nd while 
amount of water in 1^st becomes equal to amount of milk in 2^nd.

Hence, option (d).

Answer: 200

Explanation :

Let x cc is removed from first bottle and replaced with solution from second bottle.

Using alligation rule

⇒ (800-x)/x = 4/12 = 1/3

⇒ 2400 – 3x = x

⇒ x = 600 cc.

∴ Solution left in second bottle = 800 – 600 = 200 cc.

Hence, 200.

Answer: 45

Explanation :

Initially the container is full of milk. Let the capacity of container be ‘V’ liters.

When 9 liters are drawn, fraction of quantity drawn = $\frac{9}{V}$.

Hence, fraction of milk drawn will also be $\frac{9}{V}$

∴ Fraction of milk remaining will be $\left(1-\frac{9}{V}\right)$

Only water is added.

⇒ Quantity of milk remaining after first replacement = $V\left(1-\frac{9}{V}\right)$

Similarly, after second replacement quantity of milk remaining = $V{\left(1-\frac{9}{V}\right)}^2$

This is $\frac{16}{25}$ ^th  of the total volume.

∴ $V{\left(1-\frac{9}{V}\right)}^2$ = $\frac{16}{25}$V

⇒ 1 – $\frac{9}{V}$ = $\frac{4}{5}$

⇒ V = 45 liters.

Hence, 45. 

Answer: Option C

Explanation :

Let the weight of initial alloy of silver and copper be x kg and it has p% silver.

This alloy is mixed with 3 kg pure silver.

Using alligation:

⇒ $\frac{a}{3}$ = $\frac{100-90}{90-p}$ = $\frac{10}{90-p}$   …(1)

This alloy is not mixed with another alloy of 2kg containing 90% silver.

Using alligation:

⇒ $\frac{a}{2}$ = $\frac{90-84}{84-p}$ = $\frac{6}{84-p}$   …(2)

From (1) and (2), we get

$\frac{30}{90-p}$ = $\frac{12}{84-p}$

⇒ 2520 – 30p = 1080 – 12p

⇒ 18p = 1440

⇒ p = 80%

∴ a = $\frac{30}{90-p}$ = $\frac{30}{90-80}$ = 3 kg.

Hence, option (c).

Answer: 8

Explanation :

40 litres solution had dye and water in the ratio 2 : 3

∴ dye = 16 l and water = 24 l.

After adding x liters of water, now proportion is 2 : 5

⇒ $\frac{16}{24+x}=\frac{2}{5}$

⇒ x = 16 liters

Dye = 16 l and water = 40 l now in the solution.

Now, 1/4^th is removed from the solution, hence dye left = ¾ × 16 = 12 l and water left = ¾ × 40 = 30 l

Now, After adding y liters of dye, proportion becomes 2 : 3.

⇒ $\frac{12+y}{30}=\frac{2}{3}$

⇒ y = 8 liters

Hence, 8.

Answer: Option B

Explanation :

Let 1 liter of A, B and C weight 5kg, 2kg and 6 kg respectively.

Now, let’s suppose 3, 4 and 7 liters of A, B and C are mixed.

∴ Total weight of 14 liters of this solution = 3 × 5 + 4 × 2 + 7 × 6 = 65 kgs.

⇒ 65 kgs of the solution contains 7 × 6 = 42 kgs of C

∴ 130 kgs of the solution contains 2 × 42 = 84 kgs of C

Hence, option (b).

Answer: Option A

Explanation :

Given, A and B are mixed in the ratio 1 : 3

Let 10 liters of A and 30 liters of B is mixed initially i.e., total 40 liters solution.

Now volume is double by adding 40 liters of A.

∴ In the solution A = 10 + 40 = 50 liters and B = 30 liters

Concentration of alcohol in this 80-liter solution = 72%

Let the alcohol concentration in B = b%

∴ 80 × 72% = 50 × 60% + 30 × b%

⇒ 576 = 300 + 30b

⇒ b = 92%

Hence, option (a). 

Answer: Option C

Explanation :

Density of liquid 1 = 1000 g/L.

Density of liquid 2 = 800 g/L.

Half litre of the mixture weighs 480 gm, so 1 L of the mixture weighs 960 gm.

So, density of the mixture = 960 g/L.  

Using the alligation cross;

$\frac{Liquid 1}{Liquid 2}=\frac{(960-800)}{(1000-960)}$ = $\frac{4}{1}$.

Percentage of liquid 1 in the mixture = (4/5) × 100 = 80%.

Hence, option (c). 

Answer: Option D

Explanation :

Vessels A, B and C contains salt solution of strengths 10%, 22% and 32% respectively

It is also given that the amount of salt solution = 500 ml

So, Vessels A, B and C contains salt of 50 grams, 110 grams and 160 grams respectively

100 ml of Solution is transferred from A to B:

A would have 400 ml, B would have 600 ml of solution

Amount salt from A which is transferred to B = (Initial salt amount)/5 = 10 grams

So, Total salt in B = 110 + 10 = 120 grams (After first transfer)

Total salt in A = 40 grams (After first transfer)

Now, 100 ml from Vessel B is transferred to Vessel C

So similarly, 1/6th of salt would transfer from B to C

Total Salt in B = 120 - 20 = 100 grams (After second transfer)

Total Salt in C = 160 + 20 = 180 grams (After second transfer)

Now, 100 ml from Vessel C is transferred to Vessel A

So similarly, 1/6^th of salt would transfer from C to A

Total Salt in C = 160 - 30 = 130 grams (After third transfer)

Total Salt in A = 40 + 30 = 70 grams (After third transfer)

So, Vessel A contains 70 grams Salt in 500 ml solution

Strength of Salt Solution in Vessel A = 14%

Hence, option (d).

Answer: Option D

Explanation :

Let cost of tea A and B be a and b respectively.

If 3 kg of tea A is mixed with 2 kg of tea B, (3a + 2b) × 1.1 = 40× 5 = 200

If 2 kg of tea A is mixed with 3 kg of tea B, (2a + 3b) × 1.05 = 40× 5 = 200

Therefore,

(3a + 2b) × 1.1 = (2a + 3b) × 1.05

∴ 3.3a + 2.2b = 2.1a + 3.15b

∴ a/b = 19/24

Hence, option (d).

Answer: Option A

Explanation :

Let the 10 litres of mixture has ‘Y’ litres of A and (10 – Y) litres of B. Let cost of paint B be Rs. X and that of A be Rs. (X + 8).

We know that, Y ≥ (10 – Y) ⇒ Y ≥ 5

The trader makes 10% profit by selling this mixture at Rs. 264.

∴ Cost price of the mixture = $\frac{264}{1.1}$ = Rs. 240

∴ (X + 8) × Y + (10 – Y) × X = 240

∴ 10X + 8Y = 240

∴ X = 24 – 0.8Y

For maximum value of X, we need to consider minimum value of Y.

∴ X = 24 – (0.8 × 5) = Rs. 20

Hence, option (a).

Answer: Option C

Explanation :

Originally, the volume of alcohol = 4 times the volume of water. Therefore, original percentage of alcohol = 80% and original volume of water = 20%.

When 10% of the mixture is removed and replaced with water, the percentage of alcohol remained in the mixture

= $80\%\times {\left(\frac{9}{10}\right)}^2$ = 64.8%

Therefore the percentage of water in the mixture = (100 - 64.8)% = 35.2%.

Hence, option (c).

Answer: Option A

Explanation :

Suppose the concentrations of the solutions A, B and C are ‘a’, ‘b’ and ‘c’ respectively.

If the three solutions are mixed in the ratio 1 : 2 : 3, the resultant solution has 20% concentration. Therefore, we have

$\frac{a+2b+3c}{1+2+3}=20$ or a + 2b + 3c = 120   ...(1)

If the three solutions are mixed in the ratio 3 : 2 : 1, the resultant solution has 30% concentration. Therefore, we have

$\frac{3a+2b+c}{3+2+1}=30$ or 3a + 2b + c = 180   ...(2)

Multiplying equation (1) with 3 and (2) with 2 and equating both, we get

3a + 6b + 9c = 6a + 4b + 2c

⇒ 2b + 7c = 3a

Now, concentration of solution D = $\frac{2b+7c}{9}$ = $\frac{3a}{9}$ = $\frac{a}{3}$

Therefore the ratio of D's concentration to A's concentration $=\frac{a/3}{a}=\frac{1}{3}$

Hence, option (a).

Answer: Option D

Explanation :

Suppose the mixture from drum 1 = 300x litres and the mixture from drum 2 = 400x litres. Therefore, we have the following

​​​​​​​

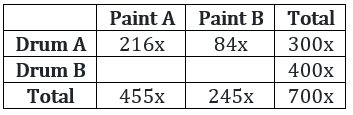
Therefore the volume of Paint A in Drum B = 455x – 216x = 239x and the volume of Paint B in Drum B = 245x − 84x = 161x.

Therefore the required ratio is 239 : 161.

Hence, option (d).

Answer: Option B

Explanation :

A 20% solution is mixed with x% solution in the ratio 1 : 3

Let 10 liters of 20% solution is mixed with 30 liters of x% solution.

Now, the total volume of the mixture = 40 liters.

This solution is mixed with 20% solution having equal volume.

∴ A total of 50 liters of 20% solutio is mixed with 30 liters of x% solution.

⇒ Final concentration = 31.25% = $\frac{20\%\times 50+x\times 30}{80}$

⇒ 2500 = 1000 + 30x
⇒ x = 50%

Hence, option (b).

Answer: Option A

Explanation :

Let the average score of the boys in the midsemester examination be b.
Average score of the girls = b + 5
Average of the class = $\frac{20\mathrm{b}+30(\mathrm{b}+5)}{50}$ = b + 3

New average of girls = (b + 5) - 3 = b + 2
Let new average of the boys = x

Average score of the entire class increased by 2 and is hence (b + 3) + 2 = b + 5

∴ $\frac{20\mathrm{x}+30(\mathrm{b}+2)}{50}$ = b + 5

⇒ 20x + 30b + 60 = 50b + 250
⇒ 20x = 20b + 190
⇒ x = b + 9.5

Increase in the average of boys is 9.5

Hence, option (a).

Answer: Option B

Explanation :

Bottle 1 contains 7/9 of milk
Bottle 1 contains 9/13 of milk

The final mixture contains 3/4 of milk

Now using the allegation rule, we get the ratio of quanities in bottles as:

⇒ $\frac{\mathrm{Quantity} \mathrm{in} \mathrm{bottle} 1}{\mathrm{Quantity} \mathrm{in} \mathrm{bottle} 2}$ = $\frac{{\displaystyle \frac{3}{4}}-{\displaystyle \frac{9}{13}}}{{\displaystyle \frac{7}{9}}-{\displaystyle \frac{3}{4}}}=\frac{{\displaystyle \frac{3}{52}}}{{\displaystyle \frac{1}{36}}}$

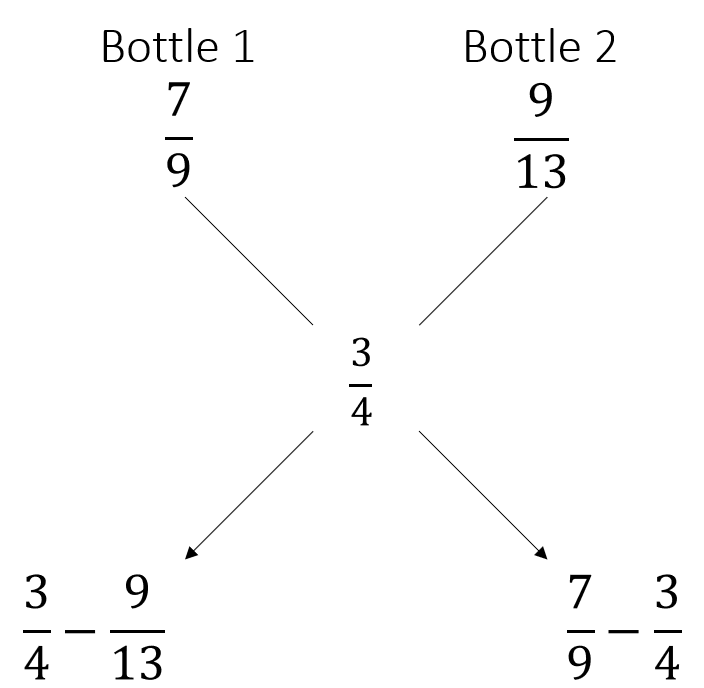
⇒ $\frac{\mathrm{Quantity} \mathrm{in} \mathrm{bottle} 1}{\mathrm{Quantity} \mathrm{in} \mathrm{bottle} 2}$ = $\frac{3}{52}+\frac{1}{36}$ = $\frac{108}{52}$ = $\frac{27}{13}$ = 27 : 13

Hence, option (b).

Answer: Option C

Explanation :

Proportion of water in three mixtures with A, B and C is $\frac{1}{3}$, $\frac{1}{4}$ and $\frac{1}{5}$ respectively.
[Sum of these proportions is 4, 5 and 6 and LCM(4, 5, 6) = 60]

Now as three mixture with A, B and C are mixed in the ratio 4 : 3 : 2.
Let the amount of three mixtures mixed be 4 × 60, 3 × 60 and 2 × 60 = 240, 180 and 120 liters respectively.

Quantity of water in liquid A, B and C is $\frac{1}{3}$ × 240 + $\frac{1}{4}$ × 180 + $\frac{1}{5}$ × 120 = 80 + 45 + 24 = 149

Quantity of liquid A in resultant mixture = $\frac{2}{3}$ × 240 = 160

Quantity of liquid B in resultant mixture = $\frac{3}{4}$ × 180 = 135

Quantity of liquid C in resultant mixture = $\frac{4}{5}$ × 120 = 96

Now let is examine the options individually

(a) The resultant mixture has 149 liters water and 160 of liquid B.
Hence, option (a) in incorrect.

(b) The resultant mixture has 135 liters of liquid B and 96 liters of liquid C.
Hence, option (b) is incorrect.

(c) Now quantity of water in resultant mixture 149 liters and quantity of liquid B is 135 liters.
Hence, option (c) is correct.

(d) Quantity of liquid A in resultant, mixture is 160 liters and quantity of water = 149 liters.
Hence, option (d) is incorrect.

Hence, option (c).

Answer: Option A

Explanation :

​​​​​​​

The quantity of milk and water is as shown in the table.

∴ Current proportion of water and milk is 40 : 60 = 2 : 3

Hence, option (a).

Answer: Option C

Explanation :

Total weight of fresh grapes = 20 kg

Weight of grape mass =

In dried grapes, water is 20%.

∴ Grape mass is 80%.

∴ Total weight of dried grapes =

Hence, option (c).

Answer: Option C

Explanation :

If the mixture is to be made 100 times as sweet as glucose, its sweetness should be 74. The ratio in which saccharin and sucrose be mixed to get the above level of sweetness is given by the following alligation table.

In other words, it means to achieve the given level of sweetness, you need to add 601 g of sucrose to 73 g of saccharin. Hence to 1 g of saccharin, the amount of sucrose to be added is $\frac{601}{73}$ = 8.23.g.

Hence, option (c).

Answer: Option A

Explanation :

$\frac{\left[\right(0.74)+(1.000)2+(1.7\left)3\right]}{6}$ = 1.31.

Hence, option (a).

Answer: Option C

Explanation :

Let the capacity of each cup be 100 ml. So 300 ml of alcohol is taken out from the first container and poured into the second one. So the first vessel will have 200 ml of alcohol and the second one will have 500 ml of water and 300 ml of alcohol. So the ratio of water to alcohol in the second vessel is 5 : 3.
Hence, proportion of alcohol in B = 3 : 8
Now if 300 ml of mixture is removed from the second container, it will have $\left(300\times \frac{5}{8}\right)$ = 187.5 ml of water and $\left(300\times \frac{3}{8}\right)$ = 112.5 ml of alcohol. Now if this mixture is poured in the second vessel, that vessel would have (200 + 112.5) = 312.5 ml of alcohol and 187.5 ml of water. Hence, ratio of alcohol to water in this container = 312.5 : 187.5 = 5 : 3
Hence, proportion of water = A = 3 : 8
Hence, we find that A = B
Note: This result will be independent of the capacity of the cup.