Geometry - Mensuration - Previous Year CAT/MBA Questions
The best way to prepare for Geometry - Mensuration is by going through the previous year Geometry - Mensuration CAT questions. Here we bring you all previous year Geometry - Mensuration CAT questions along with detailed solutions.
Click here for previous year questions of other topics.
It would be best if you clear your concepts before you practice previous year Geometry - Mensuration CAT questions.
The surface area of a closed rectangular box, which is inscribed in a sphere, is 846 sq. cm., and the sum of the lengths of all its edges is 144 cm. The volume, in cubic cm, of the sphere is
- (a)
1125π
- (b)
1125π√2
- (c)
750π√2
- (d)
750π
Answer: Option B
Text Explanation :
Workspace:
If the area of a regular hexagon is equal to the area of an equilateral triangle of side 12 cm, then the length in cm of each side of the hexagon is
- (a)
4√6
- (b)
√6
- (c)
6√6
- (d)
2√6
Answer: Option D
Text Explanation :
Area of the equilateral triangle = √3/4 × (12)2
Let the length of each side of the regular hexagon be ‘a’.
Area of a regular hexagon with side ‘a’ = 6 × √3/4 × (a)2
Now, 6 × √3/4 × (a)2 = √3/4 × (12)2
⇒ a2 = 24
⇒ a = 2√6
Hence, option (d).
Workspace:
On a rectangular metal sheet of area 135 sq in, a circle is painted such that the circle touches two opposite sides. If the area of the sheet left unpainted is two-thirds of the painted area then the perimeter of the rectangle in inches is
- (a)
- (b)
- (c)
- (d)
Answer: Option C
Text Explanation :
Area of the sheet left unpainted is two-thirds of painted area i.e., area of the circles will be three-fifth of the total area.
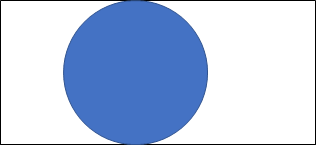
∴ Area of the circle = 3/5 × 135 = 54 sq. in.
Let the radius of the circle be r and l be the length of the rectangle. Width of the rectangle will be 2r.
⇒ πr2 = 81
⇒ r =
Area of rectangle = 135 = 2r × l
⇒ l = = =
∴ Perimeter of the rectangle = 2(l + r) = =
Hence, option (c).
Workspace:
A solid right circular cone of height 27 cm is cut into two pieces along a plane parallel to its base at a height of 18 cm from the base. If the difference in volume of the two pieces is 225 cc, the volume, in cc, of the original cone is
- (a)
256
- (b)
232
- (c)
264
- (d)
243
Answer: Option D
Text Explanation :
When a cone of height ‘H’ is cut at a distance of ‘h’ from the top
⇒ =

∴ = =
⇒ If volume of the smaller cone = x, the volume of original cone is 27x, and volume of the frustum = 26x
⇒ 26x – x = 225
⇒ x = 9
∴ Volume of the original cone = 27x = 27 × 9 = 243.
Hence, option (d).
Workspace:
If the rectangular faces of a brick have their diagonals in the ratio 3 : 2√3 : √15, then the ratio of the length of the shortest edge of the brick to that of its longest edge is?
- (a)
√3 : 2
- (b)
2 : √5
- (c)
1 : √3
- (d)
√2 : √3
Answer: Option C
Text Explanation :
Ratio of the three diagonals is 3 : 2√3 : √15
Let the legths of the three diagonals be 3k, (2√3)k and (√15)k.
And, the brick have length, breadth, height as x, y and z respectively.
∴ x2 + y2 = (3k)2 = 9k2 ...(1)
y2 + z2 = [(2√3)k]2 = 12k2 ...(2)
z2 + x2 = [(√15)k]2 = 15k2 ...(3)
Adding (1), (2) and (3), we get;
x2 + y2 + z2 = 18k2 ...(4)
Using (4) along with any of (1), (2) and (3), we get;
x = k√6 , y = k√3 and z = 3k,
Required ratio = (k√3)/3k = 1/√3.
Hence, option (c).
Workspace:
The base of a regular pyramid is a square and each of the other four sides is an equilateral triangle, length of each side being 20 cm. The vertical height of the pyramid, in cm, is
- (a)
10√2
- (b)
8√3
- (c)
12
- (d)
5√5
Answer: Option A
Text Explanation :

In the above image, the side length of the square is equal to the side length of the equilateral triangle.
So, AB = BC = OC = 20 cm.
∆AOM is a right angled triangle with ∠AMO = 90°, AO = 20 cm. OM is the height of the pyramid.
AC is the diagonal of the square base, so AM = AC/2 = (20√2)/2 = 10√2.
So using pythagoras theorem; OM2 = AO2 − AM2 = 202 − (10√2)2 = 400 − 200 = 200.
∴ OM = 10√2.
Hence, option (b)
Workspace:
A man makes complete use of 405 cc of iron, 783 cc of aluminium, and 351 cc of copper to make a number of solid right circular cylinders of each type of metal. These cylinders have the same volume and each of these has radius 3 cm. If the total number of cylinders is to be kept at a minimum, then the total surface area of all these cylinders, in sq cm, is
- (a)
1044(4 + π)
- (b)
8464π
- (c)
928π
- (d)
1026(1 + π)
Answer: Option D
Text Explanation :
As the total number of cylinders to be kept at a minimum, the volume of each cylinder must be maximum possible.
HCF(405, 783, 351) = 27 ⇒ Volume of each cylinder = 27 cc.
∴ πr2h = 27 (where r and h represent the radius and height of the cylinder)
Given that r = 3, so πh = 3.
Number of cylinders of;
Iron = 405/27 = 15.
Aluminium = 783/27 = 29.
Copper = 351/27 = 13.
∴ Total number of cylinders = 15 + 29 + 13 = 57.
Total surface area of all the cylinders = 57 × [2πr2 + 2πrh]
Put r = 3 and πh = 3 to get;
Required surface area = 1026(1 + π) sq. cm.
Hence, option (d).
Workspace:
A right circular cone, of height 12 ft, stands on its base which has diameter 8 ft. The tip of the cone is cut off with a plane which is parallel to the base and 9 ft from the base. With π = 22/7, the volume, in cubic ft, of the remaining part of the cone is
Answer: 198
Text Explanation :

∆ABE ~ ∆ACD
⇒ =
AB = 3 ft, AC = 12 ft and CD = 4 ft ⇒ BE = 1 ft
The required volumn of the cone = - = = = 198 cubic ft.
Hence, 198.
Workspace:
From a rectangle ABCD of area 768 sqcm, a semicircular part with diameter AB and area 72π sqcm is removed. The perimeter of the leftover portion, in cm, is:
- (a)
88 + 12π
- (b)
82 + 24π
- (c)
80 + 16π
- (d)
86 + 8π
Answer: Option A
Text Explanation :
The area of the semicircle = 72π sq. cm. If ‘r’ is the radius of the semi-circular portion removed,
We get
Therefore r = 12 cm.
Therefore the diameter of the semi-circle = AB = 24 cm.
Area of rectangle ABCD = 768 sq. cm.
Therefore the length of side AC

The perimeter of the leftover portion
= Perimeter of the semicircle + l(AD) + l(DC) + l(CB)
= 12π + 32 + 24 + 32 = 88 + 12π
Hence, option (a).
Workspace:
A solid metallic cube is melted to form five solid cubes whose volumes are in the ratio 1 : 1 : 8 : 27 : 27. The percentage by which the sum of the surface areas of these five cubes exceeds the surface area of the original cube is nearest to:
- (a)
10
- (b)
50
- (c)
60
- (d)
20
Answer: Option B
Text Explanation :
The volumes of the 5 smaller cubes and the original big one are in the ratio 1 : 1 : 8 : 27 : 27 : 64.
Therefore, the sides are in the ratio 1 : 1 : 2 : 3 : 3 : 4 while the areas are in the ratio 1 : 1 : 4 : 9 : 9 : 16.
The sum of the areas of the 5 smaller cubes is 24 parts while that of the big cube is 16 parts.
The sum is 50% greater.
Hence, option (b).
Workspace:
A ball of diameter 4 cm is kept on top of a hollow cylinder standing vertically. The height of the cylinder is 3 cm, while its volume is 9π cm3. Then the vertical distance, in cm, of the topmost point of the ball from the base of the cylinder is:
Answer: 6
Text Explanation :
The height of the cylinder (h) = 3
The volume = 9π
⇒ πr2h = 9π
⇒ r = √3
The radius of the ball (R) = 2
The height of O, the centre of the ball, above the line representing the top of the cylinder is say a.

Hence, 22 = a2 + (√3)2
∴ a = 1
∴ The height of the topmost point of the ball from the base of the cylinder is = height of cylinder + height of center of ball from the top of cylinder + radius of the ball = 3 + 1 + 2 = 6.
Hence, 6.
Workspace:
The base of a vertical pillar with uniform cross section is a trapezium whose parallel sides are of lengths 10 cm and 20 cm while the other two sides are equal in length. The perpendicular distance between the parallel sides of the trapezium is 12 cm. If the height of the pillar is 20 cm, then the total area, in sq cm, of all six surfaces of the pillar is
- (a)
1300
- (b)
1340
- (c)
1480
- (d)
1520
Answer: Option C
Text Explanation :
Let us draw the base of the vertical pillar as shown below

In the above figure EF = AB = 10 cm
Also, as AD = BC, by rule of symmetry
DE = FC
Now DC = DE + EF + FC
Let DE = FC = x
20 = x + 10 + x
⇒ 2x + 10 = 20
⇒ x = 5
Now in ∆AED, AE = 12 cm and DE = 5 cm
∴ AD =
Also given AD = BC
∴ BC = 13 units
Now, total surface area of vertical pilar with base ABCD = Area of Rectangle with side AB and side (Height) 20 cm + Area of Rectangle side AD and side (Height) 20 cm + Area of Rectangle with side BC and side (Height) 20 cm + Area of Rectangle with side DC and side (Height) 20 cm + 2 × Area of Trapezium ABCD
⇒ 10 × 20 + 13 × 20 + 13 × 20 + 20 × 20 + 2 × × (20 + 10) × 12
⇒ 200 + 260 + 260 + 400 + 360
⇒ 1480 sq.cm.
Hence, option (c).
Workspace:
If three sides of a rectangular park have a total length 400 ft, then the area of the park is maximum when the length (in ft) of its longer side is
Answer: 200
Text Explanation :
Let ‘x’ and ‘y’ be the dimensions of the rectangle
Let us suppose 2x + y = 400 … (1)
Area = xy.
For xy to be maximum, 2xy should also be maximum.
Now the product 2xy will be maximum when 2x = y. [AM ≥ GM]
So y + y = 400 [From (1)]
⇒ 2y = 400 or y = 200
Substituting value of y in (1) we get,
2x = 200 or x = 100
In a rectangle, length is greater than breadth, so we take y as the length.
Hence area of the park is maximum when length is 200 ft.
Hence, 200.
Workspace:
Consider a right circular cone of base radius 4 cm and height 10 cm. A cylinder is to be placed inside the cone with one of the flat surface resting on the base of the cone. Find the largest possible total surface area (in sq. cm) of the cylinder.
- (a)
- (b)
- (c)
- (d)
- (e)
Answer: Option A
Text Explanation :
As shown in the figure, ABC is the cross section of a cone of height 10 cm and radius of base 4 cm.
PQRS is the cross section of a cylinder which needs to be fitted inside the cone such that one of the flat faces of the cylinder (represented by RS) coincides with the base of the cone (represented by BC).

∵ ∆ABD and ∆PBR are similar triangles.
∴
∴ h = 10 - 2.5 × r
Total surface area of the cylinder = S = 2πr × (h + r) = 2πr × (10 - 2.5 × r + r) cm2
⇒ S = πr(20 - 3r) cm2 ...(1)
Now consider two terms 3r and (20 - 3r)
Now, ≥ [AM ≥ GM]
⇒ 10 ≥
⇒ 3r(20 - 3r) ≤ 100
∴ r(20 - 3r) ≤ 100/3
From (1), we have
⇒ S = πr(20 - 3r) ≤ π × 100/3
Hence, option (a).
Workspace:
Each question is followed by two statements A and B. Answer each question using the following instructions.
Mark (1) if the question can be answered by using statement A alone but not by using statement B alone.
Mark (2) if the question can be answered by using statement B alone but not by using statement A alone.
Mark (3) if the question can be answered by using both the statements together but not by using either of the statements alone.
Mark (4) if the question cannot be answered on the basis of the two statements.
ABC Corporation is required to maintain at least 400 Kilolitres of water at all times in its factory, in order to meet safety and regulatory requirements. ABC is considering the suitability of a spherical tank with uniform wall thickness for the purpose. The outer diameter of the tank is 10 meters. Is the tank capacity adequate to meet ABC’s requirements?
A. The inner diameter of the tank is at least 8 meters.
B. The tank weighs 30,000 kg when empty, and is made of a material with density of 3 gm/cc.
- (a)
1
- (b)
2
- (c)
3
- (d)
4
- (e)
5
Answer: Option B
Text Explanation :
Let the inner radius be r meter. Capacity of tank = (1 m3 = 1 kilolitre)
From statement A, since r ≥ 4m
∴ Capacity of tank > 256 m3
Since the capacity needed is more than 256 m3 statement A is insufficient.
From statement B,
Volume of the material of tank = mass/density = 30000kg/(3 gm/cc) = 10,000,000 cm3 = 10 m3
Hence the inner volume of tank = Outer volume – Volume of material of tank
Therefore, we can say that the tank capacity is adequate.
Hence, option (b).
Workspace:
The length, breadth and height of a room are in the ratio 3 : 2 : 1. If the breadth and height are halved while the length is doubled, then the total area of the four walls of the room will
- (a)
remain the same.
- (b)
decrease by 13.64%.
- (c)
decrease by 15%.
- (d)
decrease by 18.75%.
- (e)
decrease by 30%.
Answer: Option E
Text Explanation :
Let the original length, breadth and height of the room be 3x, 2x and x respectively.
∴ The new length, breadth and height are 6x, x and x/2 respectively.
Area of four walls = (2 × length × height) + (2 × breadth × height)
Original area of four walls = 6x2 + 4x2 = 10x2
New area of four walls = 6x2 + x2 = 7x2
∴ Area of wall decreases by [(10x2 − 7x2)/10x2] × 100 = 30%
Hence, option (e).
Workspace:
Answer the next 2 questions based on the information given below:
A punching machine is used to punch a circular hole of diameter two units from a square sheet of aluminium of width 2 units, as shown below. The hole is punched such that the circular hole touches one corner P of the square sheet and the diameter of the hole originating at P is in line with a diagonal of the square.
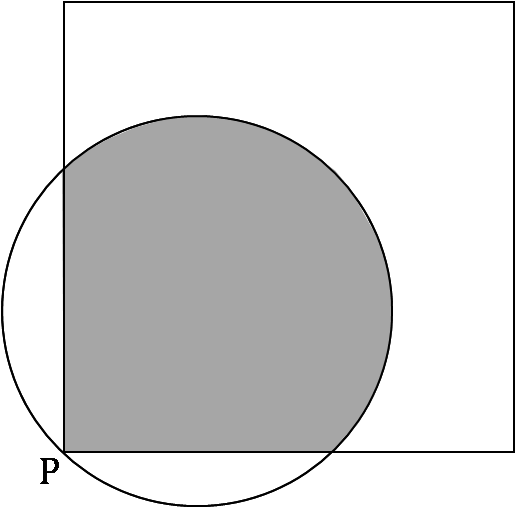
The proportion of the sheet area that remains after punching is:
- (a)
- (b)
- (c)
- (d)
- (e)
Answer: Option B
Text Explanation :

Let PQRS be the square sheet and let the hole have centre O.
As P lies on the circumference of the circle and as m ∠APC = 90°, AC is a diameter.
∵ BP is a diameter, m ∠PAB = m ∠BCP = 90°.
∵ BP = AC, ABCP is a square.
∴ m ∠POC = 90° and OP = OC = 1 unit
The area of part of the circle falling outside the square sheet
= 2 × (Area of sector OPC – Area of ∆ OPC)
Area of part of hole on sheet = Area of hole − Area of part of the circle falling outside the square sheet
Part of square remaining after punching = Area of square − Area of part of hole on sheet
∴ Proportion of sheet area that remains after punching
Hence, option (b).
Workspace:
Find the area of the part of the circle (round punch) falling outside the square sheet.
- (a)
- (b)
- (c)
- (d)
- (e)
Answer: Option D
Text Explanation :
We have calculated this value while solving the previous question.
The area of part of the circle falling outside the square sheet
= 2 × (Area of sector OPC – Area of ∆OPC)
Hence, option (d).
Workspace:
A jogging park has two identical circular tracks touching each other, and a rectangular track enclosing the two circles. The edges of the rectangles are tangential to the circles. Two friends, A and B, start jogging simultaneously from the point where one of the circular tracks touches the smaller side of the rectangular track. A jogs along the rectangular track, while B jogs along the two circular tracks in a figure of eight. Approximately, how much faster than A does B have to run, so that they take the same time to return to their starting point?
- (a)
3.88%
- (b)
4.22%
- (c)
4.44%
- (d)
4.72%
Answer: Option D
Text Explanation :
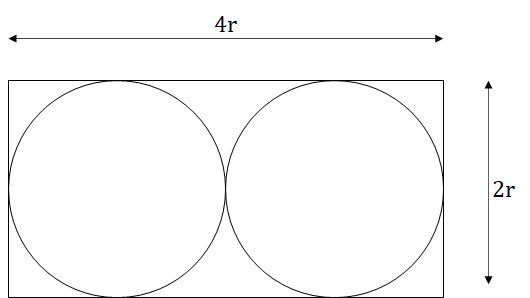
Let r be the radius of the circular tracks.
Length and breadth of the rectangular track are 4r and 2r respectively.
Length (perimeter) of the rectangular track = 12r
Length of the two circular tracks (figure of eight) = 4πr
If A and B have to reach their starting points at the same time,
(where a and b are the speeds of A and B respectively)
∴
⇒
∴ (b - a) × = 0.047 × 100 = 4.7%
Hence, option (d).
Workspace:
Rectangular tiles each of size 70 cm by 30 cm must be laid horizontally on a rectangular floor of size 110 cm by 130 cm, such that the tiles do not overlap. A tile can be placed in any orientation so long as its edges are parallel to the edges of the floor. No tile should overshoot any edge of the floor. The maximum number of tiles that can be accommodated on the floor is
- (a)
4
- (b)
5
- (c)
6
- (d)
7
Answer: Option C
Text Explanation :

This problem can be solved by trying different ways of placing the tiles on the floor. The maximum number of tiles that can be accommodated is 6, as shown in the figure.
Hence, option (c).
Workspace:
A rectangular floor is fully covered with square tiles of identical size. The tiles on the edges are white and the tiles in the interior are red. The number of white tiles is the same as the number of red tiles. A possible value of the number of tiles along one edge of the floor is
- (a)
10
- (b)
12
- (c)
14
- (d)
16
Answer: Option B
Text Explanation :
Let each square tile have side = 1 unit
Let the length of the rectangular floor be m units and the breadth be n units.
Number of red tiles = (m – 2)(n – 2)
Number of white tiles = mn − (m – 2)(n – 2)
Now, (m – 2)(n – 2) = mn − (m – 2)(n – 2)
⇒ 2(mn – 2m – 2n + 4) − mn = 0
⇒ mn – 4m – 4n + 8 = 0
⇒ n(m – 4) = 4m − 8
⇒ n = 4(m – 2)/(m – 4)
Now, consider the options.
Option (a): If m = 10, n = 32/6, which is not possible as n is an integer
Option (b): If m = 12, n = 40/8 = 5, which is possible
Option (c): If m = 14, n = 48/10, which is not possible as n is an integer
Option (d): If m = 16, n = 56/12, which is not possible as n is an integer
Hence, option (b).
Workspace:
A rectangular sheet of paper, when halved by folding it at the mid-point of its longer side, results in a rectangle, whose longer and shorter sides are in the same proportion as the longer and shorter sides of the original rectangle. If the shorter side of the original rectangle is 2, what is the area of the smaller rectangle?
- (a)
4√2
- (b)
2√2
- (c)
√2
- (d)
None of the above
Answer: Option B
Text Explanation :
Let the length and breadth of the rectangle be x and y respectively.
x > y
By conditions,
∴
∴ = 4 (∵ y = 2)
∴ x2 = 8
∴ x = 2√2
∴ Area of the smaller rectangle = 2√2 sq.units.
Hence, option (b).
Workspace:
If the lengths of diagonals DF, AG and CE of the cube shown in the adjoining figure are equal to the three sides of a triangle, then the radius of the circle circumscribing that triangle will be _______.

- (a)
equal to the side of the cube
- (b)
times the side of the cube
- (c)
times the side of the cube
- (d)
impossible to find from the given information
Answer: Option A
Text Explanation :
Let the side of the cube be a units.
DF, AG and CE are body diagonals each of length a√3 units.
∴ Circumradius of the equilateral triangle = = a units
∴ Circumradius = side of cube
Hence, option (a).
Workspace:
Let A and B be two solid spheres such that the surface area of B is 300% higher than the surface area of A. The volume of A is found to be k% lower than the volume of B. The value of k must be
- (a)
85.5
- (b)
92.5
- (c)
90.5
- (d)
87.5
Answer: Option D
Text Explanation :
Ratio of areas of two spheres = s1 : s2 = 4 : 1
∴ Ratio of their radii = r1 : r2 = 2 : 1
∴ Ratio of their volumes = v1 : v2 = 8 : 1
Volume of A is 12.5% (1/8th) of the volume of B.
But, volume of A is k% less than B.
∴ k = (100 − 12.5) = 87.5%
Hence, option (d).
Workspace:
There are 8436 steel balls, each with a radius of 1 centimetre, stacked in a pile, with 1 ball on top, 3 balls in the second layer, 6 in the third layer, 10 in the fourth, and so on. The number of horizontal layers in the pile is
- (a)
34
- (b)
38
- (c)
36
- (d)
32
Answer: Option C
Text Explanation :
The first layer has 1 ball.
Second layer has 1 + 2 = 3 balls
Third layer has 1 + 2 + 3 = 6 balls
∴ The nth layer of the stack would have balls
∴ Total balls in all the layers = = 8436
∴ = 8436
Only n = 36 satisfies the above equation.
Hence, option (c).
Workspace:
Feedback
Help us build a Free and Comprehensive Preparation portal for various competitive exams by providing us your valuable feedback about Apti4All and how it can be improved.
 Solution
Solution Discuss
Discuss Report
Report