Concept: Time, Speed and Distance
CONTENTS
The basic concept of Time, Speed & Distance is used in solving problems based on Relative motion, circular motion, motion in a straight line, trains etc. This chapter is among the most important chapters from the point of view of any MBA Entrance Test. Students are advised to understand the concepts of this chapter clearly especially “Concept of Relativity”.
The rate at which a body covers a distance is known as its speed. Mathematically, the above can be represented with the help of the formula :
Speed =
In other words, speed is the distance covered per unit time.
The basic units to represent speed are km/hr, m/s, etc.
1 km/hr = m/s
1 m/s = km/hr
Example: A plane covers the distance of Mumbai to Bhopal in 1 hr and 30 minutes. Calculate the speed of the plane if the distance between Bhopal and Mumbai is 800 kms.
Solution:
Speed =
⇒ Speed = = 533 km/h
Example: Express 27 km/h into m/s.
Solution:
27 km/h = 27 × m/s = 7.5 m/s
Example: Express 12 m/s into km/h.
Solution:
12 m/s = 12 × kmph = 43.2 km/h
Example: A man travels to his office 50 kms in two hours. He does it partly in a chartered bus which travels at the rate of 40 km/h and partly on foot at the rate of 10 km/h. Find how much distance does the man travel on foot?
Solution:
Let x be the distance travelled by bus so the distance travelled on foot = 50
– x
⇒ + = 2
⇒ x = 40 kms
We have, Distance = Speed × Time
Using the concept of proportionality that we learnt in Variation chapter, we can get some important results.
- When speed is constant, distance travelled is directly proportional to the time travelled.
i.e., D ∝ T
⇒ =
- When time travelled is constant, distance travelled is directly proportional to the speed.
i.e., D ∝ S
⇒ =
- When distance travelled is constant, speed travelled is inversely proportional to the time travelled.
i.e., S ∝ 1/T
⇒ =
So, if S1, S2, S3 and T1, T2, T3 are the speeds and times for a given body to cover a given distance then it can be inferred that: S1 : S2 : S3 = : :
Example: Rashmi walks to her bus stop at 5 km/h and reaches there late by 10 minutes. On the next day she increases her speed to 6 km/h and reaches the bus stop 10 minutes early. How far is the bus stop?
Solution:
Let x be the distance of the bus stop
The difference in time taken for both speeds = 10 + 10 = 20 minutes = 20/60 hours
⇒ – =
⇒ x = 10 km/h
Alternate Method:
Ratio of speeds = 5 : 6, hence the ratio of time taken = 6 : 5
So, we can assume that the actual times taken are 6x and 5x respectively.
⇒ 6x – 5x = 20 ⇒ x = 20 minutesHence, time taken at 6 kmph in minutes = 5 × 20 = 100 minutes or 5/3 hours
The distance = 6 × 5/3 = 10 kms
If a particular object/person travels at different speeds during different time-intervals, then average speed can be calculated by dividing the total distance travelled by the total time it takes to travel that distance.
Example: Amar travels at a speed of 10 kmph for 2 hours and then increases his speed to 20 kmph for next 1 hour. What is his average speed for the entire distance.
Solution:
Total distance travelled by Amar = 10 × 2 + 20 × 1 = 40 kms.
Total time taken to cover this distance = 2 + 1 = 3 hours.
∴ Average speed = 40/3 kmph.
Here, average of the two speeds = (10 + 20)/2 = 15 kmph.
Note: Average speed is not necessarily equal to average of speeds.
Example: A boy covers some distance at the rate of 15 km/h on a bike, and he returns back at a speed of 12 km/h. If the time taken for the travel is not known, find the average speed at which the boy travels?
Solution:
Let the distance covered by the boy each way b “x”
Average speed = D/T
D = Total distance = 2x,T = Total Time = + (Time = )
Average speed = + = 13 kmph.Average speed when time travelled is same
When a person travels at 2 or more than two different speeds for same amount of time, average speed is simple average of individual speeds.
For e.g. if Galib travels at speeds of a, b and c kmph for same amount of time, let us calculate his average speed.
Let the time travelled at each of the three speeds = t hours.
Total time travelled = t + t + t = 3t
Total distance travelled = at + bt + ct
Average speed = = =
This is the only case where average speed = average of speeds.
Average speed when distance travelled is same
When a person travels at 2 or more than two different speeds for same amount of distance, average speed is same as harmonic mean of individual speeds.
For e.g. if Galib travels at speeds of a, b and c kmph for same amount of distance, let us calculate his average speed.
Let the distance travelled at each of the three speeds = d kms.
Total distance travelled = d + d + d = 3d
Total time travelled = + +
Average speed = = =
Note: When a person travels at two different speeds i.e., x and y, for same amount of distance, the average speed is also given by the formula
Example: Deepak covers a total distance of 300 kms at three different speeds of 30, 60 and 80 kmph. Find his average speed for the entire duration.
Solution:
Deepak covers 100 kms at each of the three different speeds.
Total time taken = + +
∴ Average speed =
= = 48 kmph.
Example: If Karan travels 30 kms at 30 kmph, at what speed should he travel the next 30 kms such that his average speed for the entire journey is 48 kmph.
Solution:
Total distance travelled by Karan = 30 + 30 = 60 kms
Let speed for second part of the journey be ‘s’ kmph.
∴ Total time taken = +
⇒ Average speed = 48 =
⇒ 4 = 5/(1+30/s)
⇒ 4 + = 5
⇒ s = 120 kmph
Example: Suresh travels by a hovercraft from Vashi to Gateway of India. Every day he catches the Hovercraft at 9 a.m. One day, however, he is delayed by five minutes because of a railway crossing which he has to cross to reach Vashi from his home. He normally takes 20 minutes to reach Vashi by his car from Panvel just 30 kms from Vashi. If the railway crossing is 21 kms from Panvel, find at what speed should. Suresh travel in order to reach on time?
Solution:
The average speed of Suresh S = 20/30 = 2/3 km/h
Suresh must have travelled from Panvel to the railway crossing at the normal speed. The time required by Suresh
to travel the remaining distance from Railway crossing to Vashi is = 2/3 × 9 = 6 minutes, out of these 6
minutes required by Suresh, 5 minutes go waste due to the delay at the crossing. This results in Suresh’s
being required to travel the remaining distance of 9 kms in one hour. Hence, required speed = 9/1 = 9 km/h
Example: If two persons start at the same time from two points A, B, towards each other and after crossing each other they take X and Y hours in reaching B and A respectively then what is the ratio of the speed of one with respect to the other?
Solution:
let the man starting from A be P and the man starting from B be Q then let their
speeds be 'p' and 'q' respectively.
Let C be point where they meet after t hours.
Distance AC is covered by P in t hours and by Q in Y hours.
⇒ AC = p × t = q × Y
⇒ = ...(1)
Similary, Distance BC is covered by P in X hours and by Q in t hours.
⇒ BC = p × X = q × t
⇒ = ...(2)
Multiplying (1) and (2), we get
⇒ =
∴ =
Relative speed is the rate of change of distance between two moving objects.
Let us take an example to understand the concept of relative speed.
Suppose two trains are running on parallel tracks in the same direction at 50 kmph. For a person sitting in one of these train, it would seem that the other train is not moving at all i.e. it is standing still. In other words, with respect to this person the speed of the other train is zero. Although the second is actually moving at 50kmph, but for the person sitting in the first train (which is also moving at 50kmph) it looks stationary.
Let us take one more example. Now suppose you and your friends house are at two ends of a street 500 meters apart. Both of you start from your respective houses to meet each other at speeds of 5 m/s and 10 m/s.
In 1 second, you would travel 5 meters and your friend will travel 10 meters. hence, the distance between you will reduce by 15 meters. Hence, with respect to you, your friend's speed is 15 meters per second.
Relative speed is the most important fundamental used in solving a variety of questions on time and speed. Whenever we consider two bodies in motion simultaneously we must consider the relative speed of the two bodies in order to compute different problems.
- If two bodies are travelling at speeds of 'a' and 'b' (a > b). Their relative speed when they travel in:
- Opposite direction = (a + b) i.e. sum of the speeds
- Same direction = (a - b) i.e. difference of the speeds
- Relative speed of A w.r.t. B is same as relative speed of B w.r.t. A.
Example: A policeman running at 12 kmph is trying to catch a thief running at 8 kmph. If the thief is currently 16 kms ahead of the policeman, how long will it take to catch the thief
Solution:
Since both of them are running in same direction, relative speed of policeman
w.r.t. thief = 12 - 8 = 4 kmph
∴ time required to catch the thief = 16/4 = 4 hours.
Example: A train travelling at a rate of 25 km/h leaves Bhopal at 9 am and another train travelling in the same direction at a speed of 35 km/h starts at 1 pm. How many kms from Bhopal would both the trains meet and when would they meet?
Solution:
The first train travels from 9 am till 1 pm i.e. for 4 hours at 25 kmph.
Distance travelled by it = 4 × 25 = 100 kms.
Now, the second train starts from Bhopal at 35 kmph.
Relative speed = 35 - 25 = 10 kmph
∴ Time taken for the two trains to meet = D/(a - b) = 100/(35 - 25) = 10 hrs
Distance of meeting point from Bhopal will be same as the distance covered by the train starting from Bhopal.
∴ The trains will meet at a distance of 10 × 35 = 350 kms from Bhopal
Example: A bus starts from Bhopal at 10 am and goes to Indore. Another bus follows at a speed of 65 km/h at 11 a.m. Find at what distance from Bhopal will the second bus catch the first bus? ( Given the speed at which the first bus moves is 50 km/h)
Solution:
To use relative velocity let us first find out
the distance between both the buses when the
second bus starts moving.
In the first one hour the distance travelled by the first bus is 50 kms.
When the second bus starts moving then, since they are both moving in the same direction, the relative speed is given by : b – a
and time required by the second bus is given by : T = D/(b - a)
⇒ T = 50/(65 - 50) = 10/3 hrs
∴ distance travelled by the second bus is = 10/3 × 65 = 216.45 kms
In such questions, there will be two people who start from two different points towards each other. Each person goes to the starting point of the other person, turns back and goes to his own starting point. This cycle keeps going on. The questions would be related to their meeting points.
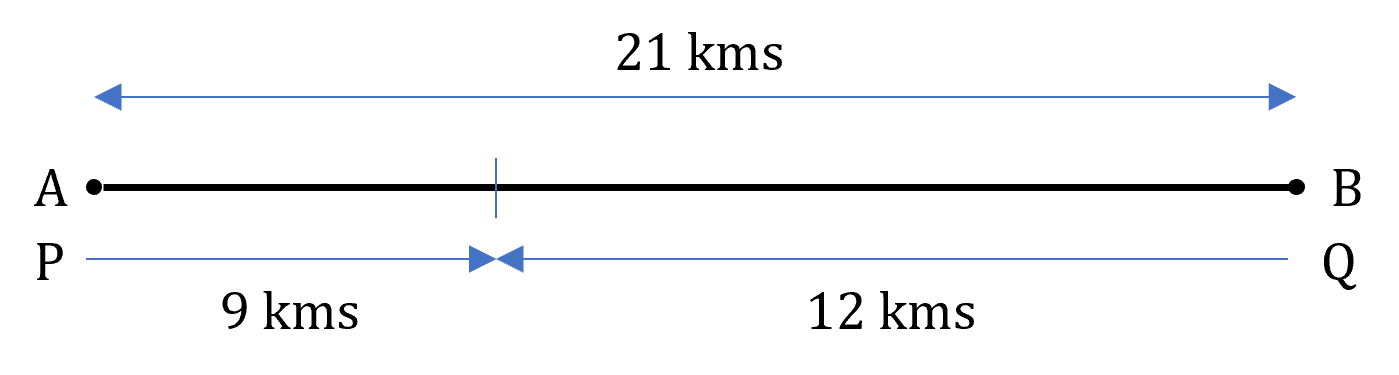
Example: Two men P and Q start from two different points A and B and move in opposite directions at speeds of 3 km/h and 4 km/h respectively. They travel to B and A respectively and again return to their respective positions. Find when and at what point from A would they both meet for the first and the second time? (Given AB = 21 kms)
Solution:
First meeting point:
Initial distance between P and Q is 21
kms and their relative speed = (3 + 4) = 7 kmph
∴ Time taken to meet for the first time = = 3 hours.
∴ Distance travelled by P in 3 hours = 3 × 3 = 9 kms
∴ The first meeting point is at a distance of 9 kms from A.
Second meetig point:
Let the second meeting point be at a distance of x kms from A.
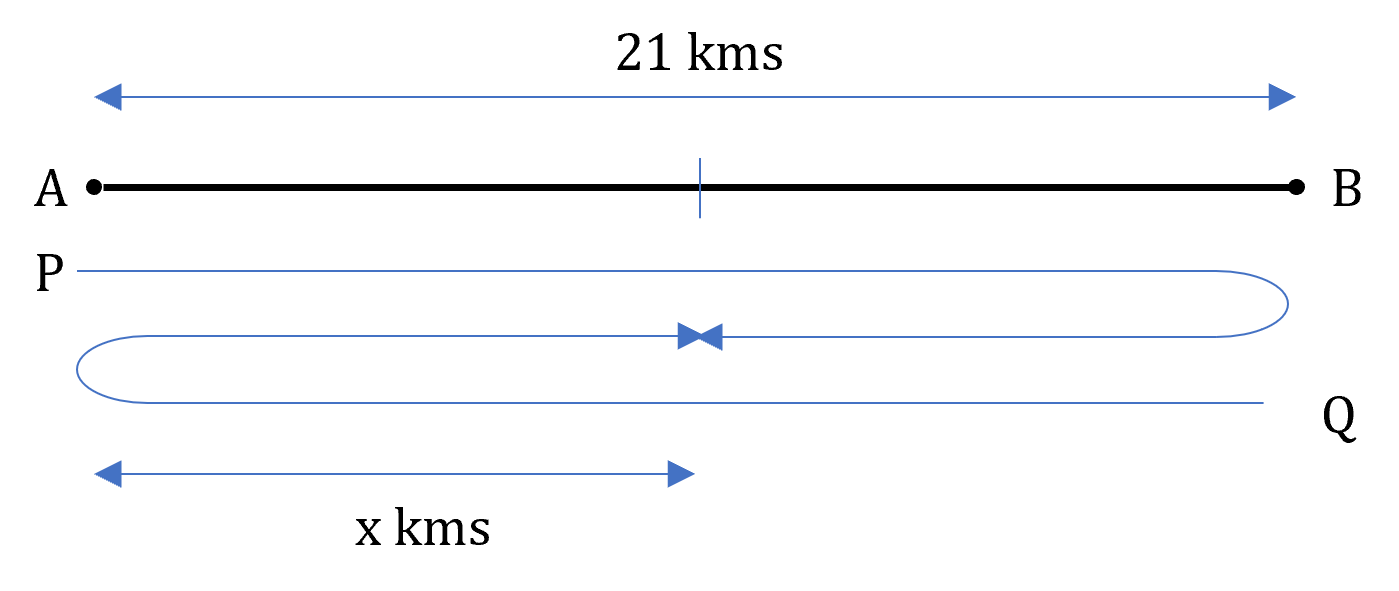
P covers a distance of (21 + 21 - x) kms to reach the second meeting point whereas Q covers a distance of (21 + x) kms to reach the second meeting point.
Both of them cover this distance in the same amount of time.
Ratio of speeds = Ratio of distances travelled.
⇒ =
⇒ 63 + 3x = 168 - 4x
⇒ 7x = 105
⇒ x = 15
∴ The second meeting point is at a distance of 15 kms from A.
- Two people P and Q start from points A and B respectively towards each other. They keep running back and forth between these two points.
If the ratio of speeds of faster and slower person is less than 2
Time taken to meet for nth time = (2n - 1)
where, D is the distance between the two end points and a and b are their speeds.
Example: Two men P and Q start from two different points A and B and move in opposite directions at speeds of 3 km/h and 7 km/h respectively. They travel to B and A respectively and again return to their respective positions. Find the time taken by them to meet for the first and the second time? (Given AB = 40 kms)
Solution:
First meeting point:
Initial distance between P and Q is 40
kms and
their relative speed = (3 + 7) = 10 kmph
∴ Time taken to meet for the first time = = 4 hours.

Second meetig point:
Let the second meeting point be at a distance of x kms from A.

Since Q is much faster than P, Q will meet P for second time even before P reaches the opposite end point.
P covers a distance of x kms to reach the second meeting point whereas Q covers a distance of (40 + x) kms to reach the second meeting point.
Both of them cover this distance in the same amount of time.
Ratio of speeds = Ratio of distances travelled.
⇒ =
⇒ 120 + 3x = 7x
⇒ 4x = 120
⇒ x = 30
∴ Time taken by P to reach second meeting point is = = 10 hours.
Example: Two men A and B start from two different points X and Y respectively towards other end. After meeting each other A takes 45 mins to reach the other end while B takes 20 mins to reach the other end. Find the time taken by them to meet from the start and the ratio of speeds.
Solution:
Let the time taken to meet from start is t mins.
Let speeds of A and B be 'a' and 'b' meters/min.
Distance XD is covered by A in t mins and by B in 45 mins ∴ a × t = b × 45 ...(1)
Distance DY is covered by A in 20 mins and by B in t mins ∴ a × 20 = b × t ...(2)
(1) × (2)
⇒ a × t × a × 20 = b × 45 × b × t
⇒
⇒
⇒
(1) ÷ (2)
⇒
⇒ t2 = 900
⇒ t = 30
If two men A and B start from two different points X and Y respectively towards other end and after meeting each other A takes ta mins to reach the other end while B takes tb mins to reach the other end.
Let the time taken to meet from start is t mins.
Let speeds of A and B be 'a' and 'b' meters/min.
Distance XD is covered by A in t mins and by B in tb mins ∴ a × t = b × tb ...(1)
Distance DY is covered by A in ta mins and by B in t mins ∴ a × ta = b × t ...(2)
(1) × (2)
⇒ a × t × a × ta = b × tb × b × t
⇒
(1) ÷ (2)
⇒
⇒ t2 = ta × tb
⇒ t =
∴ Time to meet = and
Ratio of Speeds
These questions deal with moving objects (especially trains) with certain length.
- The distance traveled by a train to cross a pole or person is equal to the length of the train.
- The distance traveled by train when it crosses a platform is equal to the sum of the length of the train and length of the platform.
- The distance traveled by train when it crosses the driver of another train is equal to only its own length
Example: Two trains 80 m and 120 m in length respectively are running at 45 km/h and 55 km/h. Find the time they will take to cross each other if it is known that they are travelling in opposite direction?
Solution:
The total length to be covered by both the trains together = 120 + 80 = 200 m
Since, the trains are travelling in opposite directions the relative speed will be the addition of the two given speeds = 45 + 55 = 100 km/h = 100 × 5/18 m/s
∴ Time required will be = = = 7.2 sec.
Example: Two trains 80 m and 120 m in length respectively are running at 45 km/h and 55 km/h. Find the time taken by faster train to overtake the slower train?
Solution:
The total length covered by both the trains together = 120 + 80 = 200 m
Since, the trains are travelling in same directions the relative speed will be the difference of the two given speeds = 55 - 45 = 10 km/h = 10 × 5/18 m/s
∴ Time required will be = = = 72 sec.
Example: A train 100 m long takes 5 seconds to cross a man walking at the rate of 5 m/s. Find the speed of the train if the man and the train are travelling in the opposite direction?
Solution:
Let the speed of the train be ‘V’ m/s
Relative speed of train and man = V + 5
The time required by the train to cross the man = 5 sec =
Hence, V = 15 m/s.
Example: Two trains of length 100 m and 200 m are travelling in opposite directions at respective speeds of 30 and 24 kmph. How long will it take for the slower train to cross the driver of the faster train?
Solution:
Relative speed of the two trains = 24 + 30 = 54 kmph = 54 × 5/18 m/s = 15
m/s
Here the slower train has to cross the driver of faster train hence we will not be considering the length of the faster train.
∴ Required time = 200/15 = 40/3 seconds.
Example: A train crosses a bridge in 25 seconds when running at 72 km/h and crosses another bridge twice as long in 20 seconds when running at the rate of 162 km/h. Find the length of the train and the length of the bridge?
Solution:
72 km/h = 20 m/s and 162 km/h = 45 m/s
Let the length of the train and the smaller bridge be T & B respectively.
= 25 and = 20
Thus, on solving the above equations, we get T and B as 100 m and 400 m respectively.
Example: A train 120 m long and travelling at 90 km/h overtakes another train running at 72 km/h and passes the second train completely in 50 seconds. Find the length of the second train?
Solution:
Let the length of the second train be L then.
Relative speed of the two trains = 90 - 72 = 18 kmph = 5 m/s
⇒ (120 + L)/5 = 50
∴ L = 130 m
Example: Two trains, one half as long as the other, cross each other in 20 seconds and the faster one can overtake the slower one in 50 seconds. Find the speed of each of the trains if the longer train is 100 m long?
Solution:
The length of longer train = 100 m hence, that of shorter train = 50 m.
Let 'a' and 'b' be the speeds of the two trains.
∴ 150/(a + b) = 20 and 150/(a - b)=50.
⇒ a + b = 75 and a – b = 3
On solving the above equations, we get a and b as 39 and 36 m/s respectively.
These problems revolve around the movement of bodies in still and moving fluids. If a boy swims in still water : e.g. a pond or a swimming pool then the speed with which he moves is the speed of that boy in still water. Now imagine the same boy swimming in the river, then he can either swim with the flow of water or against the flow of water. This movement of the boy in the river with the flow of water is called downstream movement whereas the same movement of water against the flow of water is called as an upstream movement. If the speed of the boy is known to be “b” and that of the flow of water is known to be “r” then
Upstream speed (SU) = b - r and Downstream speed (SD) = b + r
- Speed of boat in still water =
- Speed of river =
Example: A man can row upstream at the rate of 16 km/h and downstream at the rate of 20 km/h. Find the speed of the man in still water and also the speed of the flow of water.
Solution:
SU = b - r = 16 and SD = x + y = 20.
On solving the equations we get x = 18 km/h and y = 2 km/h
Example: A man can row 6 kms in still water and it takes him thrice as long as to go up the stream as it takes to go down the stream. Find the rate of the stream.
Solution:
Let the distance to be covered up and
down the stream be D then he the time required by him to go down
the stream and up the stream respectively, are
TD =
and TU =
Given, TU = 3 × D
⇒ = 3 ×
On solving the given equation we get y = 3
Example: A man rows 30 kms upstream and 44 kms downstream in 10 hours on some day and the next day he rows 40 kms upstream and 55 kms downstream in 13 hours. Find the speed of the man in still water?
Solution:
Let the speed of man be ‘b’ km/h and that of the flow of water be
‘r’ then the sum of the upstream and the downstream times must be equal to
the total time required by the man to travel both the distances.
+ = 10 and + = 13
On solving the above two equations we get “d” = 11 km/h and “u” = 5 km/hNow, d = b + r and u = b - r
On solving the above two equations we get b = 8 kmph and r = 3 kmph
Example: Amar can swim up to a certain distance with the current in 6 hours and return the same distance in 9 hours. If the river flows at the rate of 3 km/h then find the speed of Ramesh in still water?
Solution:
Let “D” be the distance that Amar swims with the current so he would
have
covered the same distance against the current also. Also let the speed with which Amar can swim be
“x” then we have
the following equations.
⇒ = 6 and = 9
Thus, x = 15 km/h
- A beats B by x meters implies that in a race of L meters, when A reaches the finishing line, B is still x meters behind him.
- A beats B by t seconds implies that B reaches the finish line t seconds after A reached the finish line.
- A beats B by x meters of t seconds implies that B covers x meters in t seconds.
- A gives B a start of x meters implies that B is starting the race x meters ahead of the starting point while A starts from the starting point.
- A gives B a start of t seconds implies that A will start running t seconds after B starts.
Example: In a 900 meter race, A beats B by 90 meters or 10 seconds. Find:
(a) Speed of B.
(b) Speed of A.
(c) Time taken by A to complete the race.
Solution:
(a) A beats B by 90 meters. It implies that when A finishes the
race B is still 90 meters behind him.
Now, A beats B by 10 seconds implies that B reaches the finish line 10 seconds after A does.
Hence, B covers 90 meters in 10 seconds.
∴ SB = 90/10 = 9 meters/sec.
(b) Now, by the time A covers 900 meters (i.e. reaches finish line), B covers (900 - 90 = ) 810 meters.
∴ = =
⇒ Speed of A = 10 m/s
(c) Time taken by A to complete the race = 900/10 = 90 seconds.
Example: In a km race A gives B a head start of 100 meters and still beats him by 100 meters or 5 seconds. Find the speed of A.
Solution:
A beats B by 100 meters or 5 seconds implies that B covers the final 100 meters in
5 seconds.
∴ SB = 100/5 = 20 meters/sec.
A starts running when B is 100 meters ahead. Hence, by the time A covers 1000 meters (i.e. reaches finish line), B covers (1000 - 100 - 100 = ) 800 meters.
∴ = =
⇒ Speed of A = 25 m/s
Example: In a km race A beats B by 200 meters and B beats C by 100 meters. Find by how much does A beat C by?
Solution:
Method 1:
A beats B by 200 meters
∴ = = ...(1)
B beats C by 100 meters
∴ = = ...(2)
Multiplying (1) and (2) we get
⇒ =
Since time taken is same, ratio of speed = ratio of distance covered
∴ =
⇒ Distance covered by C (d) = 720 meters
Hence, A beats C by 1000 - 720 = 280 meters.
Method 2:
A beats B by 200 meters. It means by the time A covers 1000 meters, B covers 800 meters.
B beats C by 100 meters. It means by the time B covers 1000 meters C is 100 meters behind
∴ when B covers 800 meters, C will be 800 × = 80 meters behind B
⇒ When A finishes, B is 200 behind A and C is another 80 meters behind B.
∴ A beats C by 200 + 80 = 280 meters.
2 people running in opposite direction
Suppose two people are running along a circular track of length 'L' meters at speeds of 'a' and 'b' m/s respectively in opposite direction.
The distance between the two people is L which they are covering at relative speed of (a + b). Hence, time taken to meet for the first time anywhere on the circle = secs
Note: When they meet for the first time the situation becomes same as it was in the starting. Hence, they will again meet each other after another secs.
∴ Time to meet for nth time anywhere on the circle = n × secs
To calculate the time taken for them to meet at the starting points, let us consider each other them individually
Time taken by A to reach the starting point = secs
Time taken by B to reach the starting point = secs
∴ Time taken by B to reach the starting point = LCM
Note: To calculate the time taken to meet at the starting point, the direction in which A or B is running does not matter.
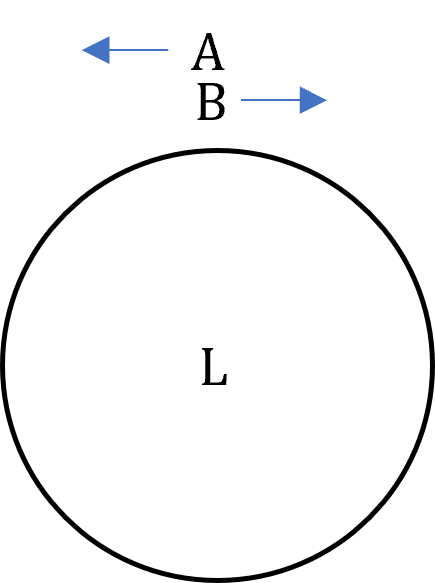
2 people running in same direction
Suppose two people are running along a circular track of length 'L' meters at speeds of 'a' and 'b' m/s respectively in same direction.
The faster one will have to cover one round more than the slower person to catch him again. Hence, the faster one will travel L meters relative to slower person at relative speed of (a - b). Hence, time taken to meet for the first time anywhere on the circle = secs
Note: When they meet for the first time the situation becomes same as it was in the starting. Hence, they will again meet each other after another secs.
∴ Time to meet for nth time anywhere on the circle = n × secs
∴ Time taken by B to reach the starting point = LCM

Example: Two people A and B are running around a 3000 meters circular track at speeds of 5 m/s and 3 m/s in opposite directions. Find the time taken for them to meet
(a) anywhere on the circle
(b) at the starting point
Solution:
(a) Time to meet anywhere on the circle = = 375 secs
(b) Time to meet at the starting point = LCM = 3000 secs
Example: Two people A and B are running around a 3000 meters circular track at speeds of 5 m/s and 3 m/s in same directions. Find the time taken for them to meet
(a) anywhere on the circle
(b) at the starting point
Solution:
(a) Time to meet anywhere on the circle = = 1500 secs
(b) Time to meet at the starting point = LCM = 3000 secs
3 people running in same direction
Suppose 3 people (A, B and C) are running around a circular track of length 'L' meters at speeds of 'a , 'b' and 'c' m/s in same direction.
A and B will meet every secs
A and C will meet every secs
∴ A will meet both B and C in every LCM secs

3 people running in different directions
Suppose 3 people are running around a circular track of length 'L' meters at speeds of 'a , 'b' and 'c' m/s such that A and B are running in same direction while C is running in different direction.
A and B will meet every secs
A and C will meet every secs
∴ A will meet both B and C in every LCM secs

Time taken to meet at the starting point = LCM
- when running in opposite direction
- anywhere on the circle =
- at the starting point = LCM
- when running in same direction
- anywhere on the circle =
- at the starting point = LCM
- when all 3 are running in same direction
- anywhere on the circle = LCM
- at the starting point = LCM
- when two (A and B) are running in a direction and third (C) is running in opposite
- anywhere on the circle = LCM
- at the starting point = LCM
- when running in opposite direction = a + b
- when running in same direction = |a - b|
Example: Two people A and B are running around a circular track at speeds of 30 m/s and 20 m/s. Find the number of distinct meeting poinst if
(a) they are running in opposite direction
(b) they are running in same direction
Solution:
Simplified ratio of speeds = 30 : 20 = 3 : 2
of distinct meeting points when
(a) they are running in opposite direction = 3 + 2 = 5
(b) they are running in same direction = 3 - 2 = 1
The concept of escalators is quite similar to Boats and Streams. While water can flow only from a higher altitude to a lower altitude, an escalator can be a moving up escalator or a moving down escalator.
In such questions a man is generally moving on an escalator which is going up or down. Since escalators are involved, distance is measure in steps and speed is measured in steps/second
Man's speed on still escalator is 's' steps/sec and escalator's speed is 'e' steps/second, then:
- If the man is going up on a moving up escalator, his effective speed = (s + e)
- If the man is going down on a moving up escalator, his effective speed = (s - e)
- If the man is going up on a moving down escalator, his effective speed = (s - e)
- If the man is going down on a moving down escalator, his effective speed = (s + e)
Example: A man can go up a moving down escalator in 60 seconds. If escalator is moving at 2 steps/sec and speed of the person is 3 steps/sec on stationery escalator, find the time required by the man to go up when the escalator is not moving.
Solution:
Let the number of steps be 'N'.
∴ Effective speed of man while going up = (3 - 2) = 1 step/sec
∴ = 60
⇒ N = 60 steps
Now, time taken by the man to go up when the escalator is not moving = 60/3 = 20 seconds
In the above example we can do some futher analysis. We calculate that the escalator has 60 steps.
The person takes 60 seconds to come down on a moving up escalator. The distance travelled by him = 3 × 60 = 180 setps
Now think, how it is possible to take 180 steps on an escalator which has 60 steps.
to figure that out let us calculate the distance travelled by the escalator, which = 2 × 60 = 120 steps
Hence, what is happening is the man takes 180 steps upwards, whereas the escalator brings him down by 120 steps. Effectively, the man travels (180 - 120 =) 60 steps upwards.
Example: When Akash walks down, he takes 1 minute on an escalator that is moving down but takes 40 seconds when he runs down. He takes 20 steps when he is walking whereas he takes 30 steps when he is running. Calculate the total number of steps in the escalator?
Solution:
Let the number of steps be 'N', speeds of Akash while walking and running
be 'w' and 'r' respectively and speed of escalator be 'e' steps/sec.
Case 1: When Akash walks down
effective speed of Akash = w + e
⇒ N = (w + e) × 60
⇒ N = w × 60 + e × 60
⇒ N = 20 + e × 60 [Akash himself takes 20 steps while walkind, w × 60 = 20]
⇒ N = 20 + 60e ...(1)
Case 2: When Akash runs down
effective speed of Akash = r + e
⇒ N = (r + e) × 40
⇒ N = r × 40 + e × 40
⇒ N = 30 + e × 40 [Akash himself takes 20 steps while walking, r × 40 = 30]
⇒ N = 30 + 40e ...(2)
Solving (1) and (2) we get
e = 0.5 steps/sec and N = 50 steps.