Concept: Simple & Compound Interest
CONTENTS
This chapter is an application of percentage. Before going through this topic, students are advised to revise the concepts of percentages.
INTEREST
Interest is the money paid by borrower to the lender for the use of money lent.
The sum borrowed is called the principal.
The sum of principal and interest is called amount or amount due.
The interest is usually paid yearly, half yearly or quarterly which is called time or period.
The interest that is calculated for every 100 rupees usually for a year is called Rate-percent per annum.
Interest is of two kinds, Simple and Compound. When interest is calculated on the original principal (i.e. the amount borrowed initially) for any length of time, it is called Simple Interest. Whereas when interest is calculated on the outstanding principal, it is called Compound Interest.
Suppose Rs. 100 is borrowed at 10% per annum, then
Interst for first year = 10% of Rs. 100 = Rs. 10
Amount due at the end of 1st year = 100 + 10 = Rs. 110
Now for 2nd year the principal will remain the same i.e. Rs. 100
∴ Interest for second year = 10% of Rs. 100 = Rs. 10
Amount due at the end of 2nd year = Amount due at the beginning + interest for current year.
Amount due at the end of 2nd year = 110 + 10 = Rs. 120
Similarly, we can make the following tab
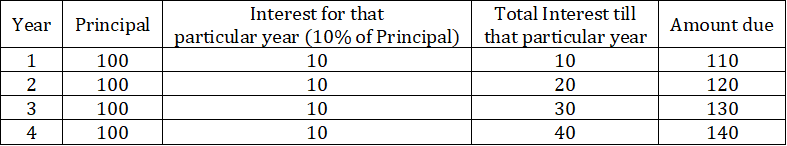
Important point to note here is that Principal used to calculate interest every year is Rs. 100 (i.e. the amount that was borrowed).
Since Principal every year is same, interest every year is same.
If,
P = Principal
r = rate of interest per annum
T = Time
An = Amount due after n years
TIn = Total Interest accumulated after n years
then,
Interest for any particular year will be same as interest for the first year.
∴ Iyear 1 = P × =
Since, interest each year is same, we can calculate interest for T years by simply multiplying interest for first
year with T.
∴ Total InterestT years = P × × T =
Amount due at the end of T years can be calculated by adding total accumulated interest with the starting
principal.
∴ Amount Dueafte T years = P + = P
Example:A sum of Rs 4,500 is lent for 6 years at 8% p.a. The S.I. and amount respectively are
(a) Rs. 2160, Rs. 6660
(b) Rs. 1775, Rs. 6275
(c) Rs. 1600, Rs. 6100
l(d) Rs. 1120, Rs. 5620
Solution:
Interest = = 2160
Amount = P + I = 4500 + 2160 = 6660
Example:In what time, a sum of money will triple itself at the rate of 10% p.a., interest calculated as S.I.
(a) 12 years
(b) 17 years
(c) 16 years
(d) 20 years
(e) None of these
Solution:
Let principal = P. Then, amount = 3P and interest = 2P.
⇒ Amount due = P
⇒ 3P = P
⇒ 3 = 1 +
⇒ T = 20 years
Alternately,
1 year’s interest = 10% of P = 0.1P.
It would take for interest to become 2P = 2P/0.1P = 20 years.
Example:At what rate per cent, the simple interest on Rs. 2375 will be Rs. 475 in 5 years?
(a) 8%
(b) 6.5%
(c) 7%
(d) 3.5%
(e) 4%
Solution:
Simple Interest =
475 = , gives R = 4%.
Example:A sum of Rs 3600 is lent out in two parts in such a way that the interest on one part at 7 % for 4 years is
equal to that on another part at 2% for 10 yrs. Find the sum lent out at 7%.
(a) Rs 1500
(b) Rs 2100
(c) Rs 2000
(d) None of these
Solution:
Let P be the sum lent out at 7% and (3600 - P) be the sum lent out at 2%.
So, P × 7 × 4 = (3600 - P) × 2 × 10.
⇒ 7P = 18000 - 5P
⇒ P = Rs. 1500
Example:The simple interest on a sum of money is 1/4th of the principal and the number of years is equal to the rate
% p.a. Then the rate % p.a. is
(a) 7.5
(b) 6
(c) 5
(d) None of these
Solution:
=
⇒ R2 = 25
⇒ R = 5%
Example:A sum becomes 6 times in 6 years at S.I. The same sum will become 26 times in how many years?
(a) 26 years
(b) 48 years
(c) 30
years
(d) 57 years
(e) None of these
Solution:
Let the principal be Rs. P. Now, amount due is 6P in 6 years
⇒ 6P = P
∴ r = 250/3%
Now, amount due will be 26P in let’s say t years
⇒ 26P = P
Substituting r = 250/3%
⇒ 26P = P
∴ t = 30 years.
The same question can also be solved in the following manner:
Example:A sum becomes 6 times in 6 years at S.I. The same sum will become 26 times in how many years?
(a) 26 years
(b) 48 years
(c) 30
years
(d) 57 years
(e) None of these
Solution:
Let the principal be Rs. P.
Interest accumulate in 6 years = 5P
∴ 5P interest in accumulated in 6 years
Hence, 25P interest will be accumulated in 6 × 5 = 30 years.
Hence, P will become 26P in 30 years.
This method can be applied here because in case of SI interest every year is same.
Example:A sum triples itself in nine years at SI. In how many years will the sum become nine times of itself at the same rate of interest?
Solution:
Let the principal be Rs. P. Interest accumulate in 9 years = 2P
∴ 2P interest in accumulated in 9 years
8P interest will be accumulated in 9 × 4 = 36 years.
Hence, P will become 9P in 36 years.
Example:What sum of money will yield Rs. 60 as simple interest at 6% p.a. in 5 years?
Solution:
Total Interest = PRT/100
⇒ 60 =
⇒ Required sum = Rs. = Rs. 200
Example:At what rate of interest p.a. will a sum triple itself in 10 years?
Solution:
AT = P
⇒ 3P = P
⇒ 3 = 1 +
∴ r = 20%
Example:An amount of money lent against simple interest at 4% p.a. amounts to Rs. 381 after 6 years. Find the sum
Solution:
381 = P = =
∴ Required sum = Rs. 300
Example:A person had Rs. 2000 a part of which he lent at 5% p.a. and the rest at 4% p.a. The total interest he received annually was Rs. 96. How much did he lend at 4%?
Solution:
Let the part lent at 4% be Rs. x
∴ the part lent at 5% is Rs. (2000 – x)
Now, 96 = +
⇒ 96 =
⇒ 9600 = 10,000 - x
⇒ x = Rs. 400
Example:A certain sum of money at simple interest amounts to Rs. 379.50 in 3 years and to Rs. 453.75 in 7 years, the rate being the same in the two cases. Find the rate of interest p.a.
Solution:
Amount due after 3 years = Rs. 379.5 = P + ...(1)
and after 4 years = Rs. 453.75
⇒ Interest for 4.5 years = 435.75 - 379.50 = 74.25
∴ Interest for 3 year = 74.25 × = 49.5 ...(2)
Solving (1) and (2) we get P = Rs. 330
Using (2) we get 49.5 =
⇒ r = 5%
Example:A man invests an amount of Rs. 15,860 in the names of his three sons A, B and C in such a way that they get the same amount after 2, 3 and 4 years respectively. If the rate of simple interest is 5% then find the ratio in which the amount was invested for A, B and C?
Solution:
Let the three amounts be x, y and z respectively.
According to the question:
x = y = z
⇒ 110x = 115y = 120z
⇒ 22x = 23y = 24z
Dividing all the terms by LCM of 22, 23 and 24, we get
⇒ = =
∴ x : y : z = 552 : 528 : 506 = 276 : 264 : 253
Example:The simple interest on a certain sum of money at 4% p.a. for 4 years is Rs. 80 more than the interest on the sum for 3 years at 5% p.a. Find the sum.
Solution:
Let the sum be Rs. x, then at 4% rate for 4 years the simple interest = = Rs.
At 5% rate for 3 years the simple interest = = Rs.
Now, We have, - = 80
⇒ 16x - 15x = 8000
∴ x = Rs. 8,000
Example:The difference between the interest received from two different banks on Rs. 500 for 2 years is Rs. 2.5. Find the difference between their rates.
Solution:
I1 = 500 × 2% × r1 = 10r1
I2 = 500 × 2% × r2 = 10r2
I1 - I2 = 10r1 - 10r2 = 2.5
⇒ r1 - r2 = 0.25%
Example:What is the amount of Rs. 4,000 at 4% simple interest from Jan 10, 1984 to April 11, 1985?
Solution:
Let us calculate the number of days from Jan 10, 1984 to April 11, 1985
Number of days from January 10, 1984 to December 31, 1984 = 366 – 10 = 356 days
(Since 1984 is a leap year)
For 1 Jan, 1985 till 11 April, 1985 = 31 (Jan) + 28 (Feb) + 31 (March) + 11 (April) = 101 days
∴ Total no. of days = 356 + 101 = 457
Simple interest = Principal × Rate% × Time
⇒ Simple Interest = (Assuming 365 days in a year)
∴ S.I. = 14,624/73
∴ Amount = Principal + Simple Interest = 4000 + 200.33 = Rs. 4,200.33
Example:Three equal principals amount to Rs. 3,720 after 3, 4 and 5 years at simple interest 6% p.a., Find the principal.
Solution:
Let each principal be Rs. P
Formula : Amount Due =
Amount after 3 years = P = P ×
Amount after 4 years = P = P ×
Amount after 5 years = P = P ×
∴ Total amount due = P × = P ×
⇒ 3720 = P ×
⇒ P = Rs. 1,000
∴ Total Principal = Rs. 3000
When the simple interest (not paid as soon as it falls due) is added to the principal so that the amount due becomes the principal for the next period, is called compound interest (abbreviated as C.I.)
Suppose Rs. 100 is borrowed at 10% per annum, then
For the first year the calculation is same as that for simple interest.
Interst for first year = 10% of Rs. 100 = Rs. 10
Amount due at the end of 1st year = 100 × = Rs. 110
Now for 2nd year the principal will not be Rs. 100 but the amount due the previous year i.e. Rs. 110.
Interst for second year = 10% of Rs. 110 = Rs. 11
Amount due at the end of 2nd year = 110 × = Rs. 121
Similarly, we can make the following tab
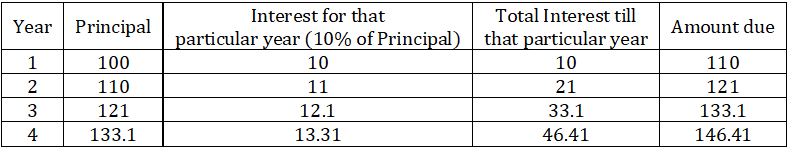
Important point to note here is that Principal used to calculate interest every year increases by 10% (i.e. the rate of interest) because of which interest each year is also increasing by 10%.
If
P = Principal
r = rate of interest per annum
T = Time
An = Amount due after n years
TIn = Total Interest accumulated after n years
then,
Interest for first year, In = P ×
Amount due after T years (AT) = P
TIT = AT – P = P- P
Example:What will be the C.I. on Rs. 2,000 for 3 yrs at 20% p.a.?
(a) Rs. 1331
(b) Rs. 1456
(c) Rs. 1900
(d) Rs. 1855
(e) None of these
Solution:
Amount due after 3 years = 2000 = Rs. 3456
CI = Amount due - Principal = 3456 - 2000 = Rs. 1456
Example:If C.I. for a certain sum for 2 years at 5% p.a. be Rs. 2050, what is the principal?
(a) Rs. 20,000
(b) Rs. 32,000
(c) Rs. 22,000
(d) Rs. 26,400
(e) None of these
Solution:
CI = 2050 = P - P
⇒ 2050 = P[(1.05)2 - 1]
⇒ 2050 = 1.1025P - P = 0.1025P
⇒ P = Rs. 20,000
Example:At what rate percent compounded yearly will Rs. 80,000 amount to Rs. 88,200 in 2 years?
Solution:
We have 80,000 = 88,200
⇒ = =
⇒ 1 + =
∴ r = 5%
Example:What sum will become Rs. 6,690 after three years and Rs. 10,035 after six years on compound interest?
Solution:
We know amount increases by r% every year.
6690 = 10,035
⇒ = = ...(1)
Now dividing the second equation by the first equation, we get :
Now, 6690 is the amount due after three years,
∴ 6690 = P
⇒ 6690 = P ×
∴ P = Rs. 4,460
Example:If a sum placed at compound interest doubles itself in 4 years. In how many years will it be eight
times?
(a) 10
(b) 13
(c) 12
(d) 11
Solution:
Amount due after T years = P
Amount doubles in 4 years,
⇒ 2P = P
∴ 2 = …(1)
Now, amount has to be 8 times in let’s say t years.
⇒ 8P = P
⇒ 8 =
⇒ 23 =
⇒ =
⇒ =
∴ t = 12 years
Note: There is not need to find the value of r separately in this question.
Here, 2 = means the amount doubles every 4 years.
This question can also be solved in the following manner:
Example:If a sum placed at compound interest doubles itself in 4 years. In how many years will it be eight
times?
(a) 10
(b) 13
(c) 12
(d) 11
Solution:
Let the sum be Rs 100
Here, 2 = means the amount doubles every 4 years.
∴ in 4 yrs Rs 100 becomes Rs 200, and in next four years Rs 200 becomes Rs 400, and again in next four years Rs 400 becomes Rs 800.
So clearly, in 12 years, the sum becomes 8 times.
Example:Rs 4800 becomes Rs. 6000 in 4 years at a certain rate of compound interest. What will be the sum after 12 years?
Solution:
We have 4800 = 6000
⇒ = 6000/4800 = 5/4
Hence, the amount becomes 5/4 times in 4 years.
In 12 (= 4 + 4 + 4) years it will become 5/4 × 5/4 × 5/4 = 125/64 times
∴ Amount due = 125/64 × 4800 = Rs. 9375
Example:If the rate of interest is 20% per annum compounded annually in what time approximately money doubles
itself?
(a) 3 years
(b) 4 years
(c) 5
years
(d) 6 years
Solution:
Let us assume that we invest Re 1
So, after 1 year, it will be Rs 1.2
After two years, it will be Rs 1.44 = (1.2)2
After three years, it will be Rs 1.728 = (1.2)3
After four years, it will be Rs 2.0736 = (1.2)4
So clearly, after 4 years, the money almost doubles itself
Interest for a particular year can be obtained by calculating r% of principal for that. Also, Principal for a particular year is same as the Amount due for the previous year.
Pn> = An-1 = P
Interest for nth year = Pn × r% = P × =
Also, if you notice the table above, interest every year increases by r%.
∴ Interest for nth year can also be obtained by increasing first year’s interest by r% (n - 1) times.
Hence, Interest for nth year = Interest for first year × =
Example:If CI for 5th year is Rs. 10,000 on a certain sum at the rate of 10% p.a. compounded annually, then what will be the compound interest for 9th year?
Solution:
We know in case of CI, interest for any particular year increases by r% every year. Since there are 4 compounding
periods between 9th and 5th year.
⇒ CI for 9th year = CI for 5th year × = 10,000 = 14,641
Example:Divide Rs 21866 into two parts such that the amount of one in 3 years is same as
the amount of second in 5 years. The rate of compound interest being 5% per annum.
(a) 11466, 10400
(b) 14466, 7400
(c)
14166, 7700
(d) None of these
Solution:
Let the amount lent for 5 years be x, hence amount lent for 3 years is (21866 - x)
∴ (21866 - x) = x
⇒ 21866 - x = x
⇒ 21866 - x = 1.1025x
⇒ x = Rs. 10,400
∴ Amount lent for 5 years is Rs. 10,400 and amount lent for 3 years = Rs. 11,466
Example:Find compound interest on Rs. 100000 at 20% p.a. for 2 years 3 months compounded annually.
Solution:
Amount due after 2 year = 100000 = 144000
Amount due after another 3 months = 144000 × = 144000 × = 151200
∴ Compound Interest = 1,51,200 – 1,00,000 = Rs. 51,200.
Example:Find the C.I. on Rs. 1200 for 1 years @ 12% p.a.
Solution:
A = 1200 = 1424.64
∴ C.I. = 1424.64 – 1200 = Rs. 224.04
Example:What is the compound interest on Rs. 2000 for two years at 5% interest p.a. for the first year and at 10% interest p.a. for the 2nd year?
Solution:
Compound Interest = 2000 - 2000
= 2000 × × - 2000 = Rs. 310
Example:What sum of money at compound interest will amount to Rs. 2249.52 in 3 years, if the rate of interest is 3% for the first year, 4% for the second year, and 5% for the third year?
Solution:
The general formula for such question is :A = P……
Where A = Amount, P = Principal and r1, r2, r3 are the rate of interest for different years.
In the above case: 2249.52 = P
⇒ 2249.52 = P × 1.03 × 1.04 × 1.05
⇒ P = = Rs. 2000
Now let’s consider Rs. 100 is lent to two people at 10% per annum on SI basis to one and CI basis to another.
Here, we will be calculating interest for a particular year for both SI and CI.
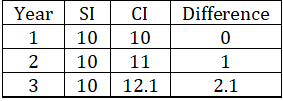
Interest for first year is same for both.
Similarly, if Rs. P is lent to two people at r% per annum on SI basis to one and CI basis to another.
x = Interest for first year =
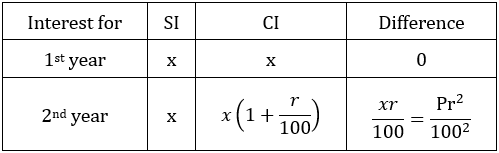
Hence, when Rs. P is lent at r% per annum on SI basis to one and CI basis to another, then
Difference in interest for first year = 0
Difference in interest for second year = (i.e. r% of first year’s interest) =
Total difference in interest for two years =
Example:The difference between the simple interest and the compound interest is Rs 8 for 2 years at the rate of 20%
per annum. What is the sum?
(a) Rs. 350
(b) Rs. 470
(c) Rs.
200
(d) 190
(e) None of these
Solution:
Difference in total interest for 2 years in case of CI and SI =
⇒ 8 =
∴ P = Rs. 200
Example:If the difference between SI for 2 years and CI for 2 years on the same sum and at the same rate
of interest is 120. The difference between SI for 3 years and CI for 3 years on the same sum and at the same rate of interest is
Rs366. Find the rate of interest?
(a) 10 %
(b) 5 %
(c) 20 %
(d) 12 %
Solution:
We know interest in case of SI is same for every year, while in case of CI increases by r% every year.
If x is the interest for first year, then we can make the following table for interest for each of the three years.
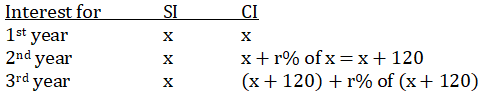
Different between CI and SI for 1st year for same sum at same rate is zero.
Difference between CI and SI for 2nd year = (x + r% of x) - x = 120
⇒ r% of x = 120 ...(1)
Difference between CI and SI for 3rd year = (x + 120 + r% of (x + 120) - x
Also, as per given in the question, difference in CI and SI for 3rd year = 366 – 120 = 246.
⇒ x + 120 + r% of (x + 120) - x = 246
⇒ x + 120 + 120 + r% of 120 - x = 246 [From (1): r% of x = 120]
⇒ r% of 120 = 6
⇒ r = 5%
Example:Find the difference between S.I. and C.I. on Rs. 1200 at the rate of 10% for 3 yrs.
(a) Rs. 18.75
(b) Rs. 37.20
(c) Rs.
23.80
(d) Rs. 28.50
(e) None of these
Solution:
CI for 3 years = 1200 - 1200 = 397.2
SI for 3 years = (1200 × 10 × 3)/100 = 360
∴ CI - SI = 397.2 - 360 = Rs. 37.2
Example: If the CI on a certain sum for 2 years at 3% be Rs. 101.50. what would be the S.I.?
Solution:
Let the prinicipale be P
CI for 2 years = P - P = 101.50
⇒ 101.50 = 1.0609P - P = 0.0609P
⇒ P = Rs. 5000/3
Simple interest = = Rs. 100