LR - Mathematical Reasoning - Previous Year CAT/MBA Questions

Answer: 2

Text Explanation :

There is exactly one prize of type a.

There can be 99 items of type b. Thus, there can be only two types of items.

Answer: 2.

Answer: 6

Text Explanation :

There is exactly one prize of type a.

As we need to find maximum possible different types of prizes, number of prizes of type b has to be minimum possible and hence must be 2, number of items of type c = 4 …and so on.

1(type a) + 2(type b) + 4(type c) + 8(type d) + 16(type e) + 32(type e) = 63

Suppose there is prize of type f then number of items has to be at least 64. But then there are more than 100 items, which is not true. So there cannot be prize of type f.

Answer: 6.

Answer: Option D

Text Explanation :

There is exactly one prize of type a.

[1]. If there are 30 items of type b, then items of type c = 100 – 30 −1 = 69. So this case is possible.

[2]. There are 75 items of type e, then items of type b, c and d = 100 – 75 −1 = 24.

Some of the values of (b, c, d) are (2, 4, 18) or (2, 5, 17) or (3, 6, 15). So this case is possible.

[3]. If there are 60 items of type d, then items of type b and c = 100 – 60 −1 = 39. So this case is possible as we can find many combinations for (b, c).

[4]. If there are 45 items of type c, then items of type a, b and c in all cannot be more than 1 + 22 + 45 = 68. Now items of type d has to be more than 90. But then total number of items exceed 100. So this case is not possible.

Hence, option (d).

Answer: Option A

Text Explanation :

Considering the given options, the maximum number of different types can be 6.

Assume that there are 6 items.

Now number of items of same type as the one in box 45 = 1 + 31 + 43 = 75

So number of remaining items = 25

1 + 2 + 4 + 8 + 16 = 31. If there are 5 types of items, the minimum number of items of 5 types = 31.

31 + 75 >100

So, there cannot be 6 types of items.

Now consider that there are 5 types of items.

Now number of items of same type as the one in box 45 = 1 + 31 + 43 = 75

So number of remaining items = 25

Now, 25 = (1 + 2 + 4 + 17) or (1 + 3 + 6 + 16) ,… etc

So there can be 5 types of different items.

Hence, option (a).

Answer: 1

Text Explanation :

Units place of (F + F) is F ⇒ F = 0 

Units place of (H + H) or (H + H + 1) is also F. This is possible only when H is 5 and there is no carry over.

So, F = 0 and H = 5

∴ (1 + B + A) = (10A + A)
⇒ 1 + B = 10A
⇒ A = 1 and B = 9

As units place of (G + K) = 1, actual value of G + K = 11

So, 1 + F + A = 1 + 1 + 0 = 2 = C

Now, 1 + J = G and G + K = 11

Also, G, J and K ∈ {3, 4, 6, 7, 8}

Therefore, (G, J, K) = (8, 7, 3) or (7, 6, 4) or (4, 3, 7), accordingly D and E can be represented by (4,6) or (3, 8) or (6, 8) in some order.

The letter A represents digit 1.

Answer: 1.

Answer: 9

Text Explanation :

Consider the solutoin to first question of this set.

B represents the digit 9.

Answer: 9.

Answer: 7

Text Explanation :

Consider the solutoin to first question of this set.

The digit 7 cannot be represented by the letter D.

Answer: 7.

Answer: 6

Text Explanation :

Consider the solution to first question of this set.

The digit 6 cannot be represented by the letter G.

Answer: 6.

Answer: Option A

Text Explanation :

70% of 20 = 14

Qualified candidate score 14 or more marks in two or more sections.

Composite scores of few students:

Chetna: 2(19) + 4 + 12 = 54

Ester: 2(12) + 18 + 16 = 58

Falak: 2(15) + 7 + 10 = 47

From (6), Jatin scored 20 marks in DI. Therefore, his Composite score is 2(20) + 16 + 14 = 70

Therefore, Indu’s Composite score = 60

If Indu scored 20 marks in DI, her score in GA = 60 – 2(20) – 8 = 12.

In this case, Indu scored less than 70% in WE and GA. But from (4) Indu was recruited. Therefore, this case is not valid. This means she must have scored 100% marks in GA i.e., 20 marks in GA.

∴ indu's marks (out of 20) in DI = $\frac{60-8-20}{2}$

$=\frac{32}{2}$ = 16

From (5), Danish and Harini also scored 20 marks in GA.

Therefore, his Composite score = 2(8) + 15 + 20 = 51

From (2), Ajay must have scored more than 18 marks. Assuming his score in WE as 19, composite score would be 2(8) + 19 + 16 = 51. This cannot be true as Danish’s composite score was 51. Thus, Ajay’s score in WE = 20 and his composite score 2(8) + 20 + 16 = 52.

Bala, Chetna and Falak scored less than 70% marks in at least two sections and hence were disqualified.

From (4), Geeta had the lowest score. Maximum composite score of Harini = 2(5) + 20 + 20 = 50

Ajay(52), Danish(51), Ester(58), Indu(60) and Jatin(70) are definitely qualified.

So, Ester(58), Indu(60) and Jatin(70) were top three scorers. Geeta must have scored more than 52 marks. Therefore marks scored by her in WE ≥ 53 – 2(14) − 6 i.e., marks scored by her in WE ≥ 19

If she had scored 20 marks in WE, her composite marks would be 2(14) + 20 + 6 = 54

But Chetna’s composite score = 54. So, Geeta’s marks in WE = 19 and her composite score = 53

Thus we have

​​​​​​​

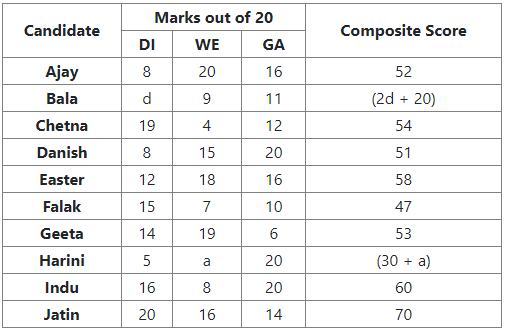
‘a’ denotes marks of Harini in WE while ‘d’ denote marks of Bala in DI.

Jatin’s composite score = 70 and Danish’s composite score = 51

Thus, statement 1 is true.

Indu’s score in DI = 16 and Chetna’s score in DI = 19

Thus, statement 2 is true.

Jatin’s score in GA = 14 and Indu’s score in GA = 20

Thus, statement 3 is false.

Hence, option (a).

Answer: Option B

Text Explanation :

Harini’s composite score = (30 + a) and Falak’s composite score = 47

Thus, statement 1 is may not be true for values of ‘a’ more than or equal to 17.

In DI, if Bala’s score is 19 or 20, his composite score would be 58 (or 60). But, no two candidates had the same composite scores. Therefore, Bala’s score must be less than or equal to 18 and hence his composite score must be less than or equal to 56.

Therefore, Chetna scored more than Bala in DI and Bala’s composite score was less than that of Ester. Thus, statements 2 and 3 are true.

If Bala scores same as Jatin in DI, Bala’s composite score would be 60. This is not valid.

Hence, statement 2 must be false.

Hence, option (b).

Answer: 13

Text Explanation :

2d + 20 < 54 ⇒ d < 17

d ≠ 14, 15, 16, 12, 8 and 5

d can take value 13 or 11 or 9 or 7 or 6 or 4 or 3 or 2 or 1.

For d = 13, the composite score = 46

Thus, Bala could have scored 13 marks in DI.

Therefore, the required answer is 13.

Answer: 13

Answer: 14

Text Explanation :

Marks scored in WE are 20, 19, 18, 16, 15, 9, 8, 7, 4 and ‘a’.

As all scored different marks, ‘a’ can take one of the following values: 17, 14, 13, 12, 11, 10, 6, 5, 3, 2, 1

For a = 17, Harini’s composite score i.e., 47 would be same as Falak’s. Hence, a ≠ 17

For a = 14, Harini’s composite score i.e., 44 which is different from others.

Therefore, the required answer is 14.

Answer: 14

Answer: 7

Text Explanation :

Preference was given to Rs. 500 notes.

The ATM machine can dispense 10 notes of Rs. 500.

(i) 500 × 10 = Rs. 5,000

If the ATM machine dispenses 9 notes of Rs. 500;

(ii) 500 × 9 = 4500, 200 × 2 = 400 and 100 × 1= 100

(Notes of Rs. 500 denomination = 9 and notes of Rs. 200 and Rs. 100 denominations = 2 + 1 = 3, 9 > 3)

(iii) 500 × 9 = 4500, 200 × 1 = 200 and 100 × 3 = 300

(Notes of Rs. 500 denomination = 9 and notes of Rs. 200 and Rs. 100 denominations = 1 + 3 = 4, 9 > 4)

(iv) 500 × 9 = 4500, 100 × 5 = 500

(Notes of Rs. 500 denomination = 9 and notes of Rs. 200 and Rs. 100 denominations = 0 + 5 = 5, 9 > 5) If the ATM machine dispenses 8 notes of Rs. 500;

(v) 500 × 8 = 4000 and 200 × 5 = 1000

(Notes of Rs. 500 denomination = 8 and notes of Rs. 200 and Rs. 100 denominations = 5 + 0 = 5, 8 > 5)

(vi) 500 × 8 = 4000, 200 × 4 = 800 and 100 × 2 = 200

(Notes of Rs. 500 denomination = 8 and notes of Rs. 200 and Rs. 100 denominations = 4 + 2 = 6, 8 > 6)

(vii) 500 × 8 = 4000, 200 × 3 = 600 and 100 × 4 = 400

(Notes of Rs. 500 denomination = 8 and notes of Rs. 200 and Rs. 100 denominations = 3 + 4 = 7, 8 > 7)

If numbers of notes of denomination Rs. 200 are reduced further, number of notes of the customer’s preferred denomination will not exceed the total number of notes of other denominations.

The ATM machine can dispense 7 notes of Rs. 500, for the minimum number of total notes of the remaining two denominations; one can have 7 notes of Rs. 200 and one note of Rs. 100. But even then, number of notes of the customer’s preferred denomination will be less than the total number of notes of other denominations. As number of notes of denominations reduces, number of notes of the denomination Rs. 500 will be less than the total number of notes of other denominations.

Thus, there are 7 different ways in which the ATM can serve a customer who gives 500 rupee notes as her preference.

Therefore, the required answer is 7.

Answer: 7

Answer: 6

Text Explanation :

Preference was given to Rs. 500 notes.

The ATM machine can dispense 10 notes of Rs. 500.

(i) 500 × 10 = Rs. 5,000

If the ATM machine dispenses 9 notes of Rs. 500;

(ii) 500 × 9 = 4500, 200 × 2 = 400 and 100 × 1= 100

(Notes of Rs. 500 denomination = 9 and notes of Rs. 200 and Rs. 100 denominations = 2 + 1 = 3, 9 > 3)

(iii) 500 × 9 = 4500, 200 × 1 = 200 and 100 × 3 = 300

(Notes of Rs. 500 denomination = 9 and notes of Rs. 200 and Rs. 100 denominations = 1 + 3 = 4, 9 > 4)

(iv) 500 × 9 = 4500, 100 × 5 = 500

(Notes of Rs. 500 denomination = 9 and notes of Rs. 200 and Rs. 100 denominations = 0 + 5 = 5, 9 > 5) If the ATM machine dispenses 8 notes of Rs. 500;

(v) 500 × 8 = 4000 and 200 × 5 = 1000

(Notes of Rs. 500 denomination = 8 and notes of Rs. 200 and Rs. 100 denominations = 5 + 0 = 5, 8 > 5)

(vi) 500 × 8 = 4000, 200 × 4 = 800 and 100 × 2 = 200

(Notes of Rs. 500 denomination = 8 and notes of Rs. 200 and Rs. 100 denominations = 4 + 2 = 6, 8 > 6)

(vii) 500 × 8 = 4000, 200 × 3 = 600 and 100 × 4 = 400

(Notes of Rs. 500 denomination = 8 and notes of Rs. 200 and Rs. 100 denominations = 3 + 4 = 7, 8 > 7)

If numbers of notes of denomination Rs. 200 are reduced further, number of notes of the customer’s preferred denomination will not exceed the total number of notes of other denominations.

The ATM machine can dispense 7 notes of Rs. 500, for the minimum number of total notes of the remaining two denominations; one can have 7 notes of Rs. 200 and one note of Rs. 100. But even then, number of notes of the customer’s preferred denomination will be less than the total number of notes of other denominations. As number of notes of denominations reduces, number of notes of the denomination Rs. 500 will be less than the total number of notes of other denominations.

if a customer’s preferred denomination is Rs. 500, the minimum number of notes of Rs. 500 the ATM machine dispenses is 8.

Hence, with fifty 500 rupee notes, the ATM machine can serve 6 customers who could have given 500 rupee notes as their preferences.

Therefore, the required answer is 6.

Answer: 6

Answer: Option B

Text Explanation :

Here we just need to keep in mind that

> the customers are to be served with at most 20 notes per withdrawal.

> The ATM machine has stock of fifty

> 500 rupee notes and a sufficient number of notes of other denominations.

(i) If the ATM machine dispenses one

(ii) 500 rupee note, for the total number of notes less than or equal to 20, notes of 200 rupee denominations has to be maximum.

500 × 1 + 200 × 22 + 100 × 1 = 5000

i.e., the minimum number of notes = 24

(iii) If the ATM machine dispenses two 500 rupee notes, for the total number of notes less than or equal to 20, notes of 200 rupee denominations has to be maximum.

500 × 2 + 200 × 20 = 5000 i.e., the minimum number of notes = 22

(iv) If the ATM machine dispenses three 500 rupee notes, for the total number of notes less than or equal to 20, notes of 200 rupee denominations has to be maximum.

500 × 3 + 200 × 17 + 100 × 1 = 5000

i.e., the minimum number of notes = 21

(v) If the ATM machine dispenses four 500 rupee notes, for the total minimum number of notes, notes of 200 rupee denominations has to be maximum.

500 × 4 + 200 × 15 = 5000

i.e., the minimum number of notes = 19

Therefore, if the ATM dispenses 4 notes of 500 rupees then it can serve a maximum of 12 customers with 50 notes.

Hence, option (b).

Answer: Option C

Text Explanation :

In order to have the total number of notes to be dispensed is the smallest possible, notes of denomination Rs. 500 has to be maximum.

The ATM machine could have dispensed 10 notes of 500 rupee to the customers who gave 500 rupee notes as their preference.

Therefore, the number of 500 rupee notes required to serve these 50 customers = 10 × 50 = 500

The ATM machine could have dispensed 10 notes of 100 rupee and 8 notes of 500 rupee to the customers who gave 100 rupee notes as their preference.

Therefore, the number of 500 rupee notes required to serve these 50 customers = 8 × 50 = 400

Total number of 500 rupee notes required = 500 + 400 = 900

Hence, option (c).

Answer: 1200

Text Explanation :

Suppose the base exchange rates of A, B and C w.r.t L are 100m, 120m and m respectively.

Therefore we have the following:

​​​​​​​​​​​​​​

Given: The outlet received 88000 units of L by selling A. Therefore the number of units of A sold 

$=\frac{88000}{110 m}=\frac{800}{m}$

From point 3, the number of units of L received by selling B = $\frac{9}{5}$ × 88000 = 158400

Therefore the number of units of B sold = $\frac{158400}{132 m}=\frac{1200}{m}$

From points 5 and 6, the number of units of A bought = 800 + $\frac{800}{m}$

Therefore, the number of units of L paid to buy A

= 95m $\left(800+\frac{800}{m}\right)$ = 76000 (1 + m)

From point 2, the number of units of L paid to buy B = 

$\frac{3}{5}$ × 76000 (1 + m) = 45600 (1 + m)

Therefore the number of units of B bought = $\frac{45600(1+m)}{114 m}=400+\frac{400}{m}$

From points 5 and 6,

$\frac{1200}{m}=400+\frac{400}{m}.$ Solving for m, m = 2

Therefore we have the following

​​​​​​​​​​​​​​

If the number of units of C sold = x, from points 5 and 6, the number of units of C bought = x + 3000.

Therefore, we get, 1.9(x+3000)=2.2x or solving for x, x = 19000.

Using the value of m, we get the following table for the number of units of A, B and C bought and sold.

​​​​​​​​​​​​​​

Answer: 1200

Answer: Option C

Text Explanation :

Suppose the base exchange rates of A, B and C w.r.t L are 100m, 120m and m respectively.

Therefore we have the following:

​​​​​​​​​​​​​​

Given: The outlet received 88000 units of L by selling A. Therefore the number of units of A sold 

$=\frac{88000}{110 m}=\frac{800}{m}$

From point 3, the number of units of L received by selling B = $\frac{9}{5}$ × 88000 = 158400

Therefore the number of units of B sold = $\frac{158400}{132 m}=\frac{1200}{m}$

From points 5 and 6, the number of units of A bought = 800 + $\frac{800}{m}$

Therefore, the number of units of L paid to buy A

= 95m $\left(800+\frac{800}{m}\right)$ = 76000 (1 + m)

From point 2, the number of units of L paid to buy B = 

$\frac{3}{5}$ × 76000 (1 + m) = 45600 (1 + m)

Therefore the number of units of B bought = $\frac{45600(1+m)}{114 m}=400+\frac{400}{m}$

From points 5 and 6,

$\frac{1200}{m}=400+\frac{400}{m}.$ Solving for m, m = 2

Therefore we have the following

​​​​​​​​​​​​​​

If the number of units of C sold = x, from points 5 and 6, the number of units of C bought = x + 3000.

Therefore, we get, 1.9(x+3000)=2.2x or solving for x, x = 19000.

Using the value of m, we get the following table for the number of units of A, B and C bought and sold.

​​​​​​​​​​​​​​

The outlet sold 19000 units of currency C on that day.

Hence, option (c).

Answer: 240

Text Explanation :

Suppose the base exchange rates of A, B and C w.r.t L are 100m, 120m and m respectively.

Therefore we have the following:

​​​​​​​​​​​​​​

Given: The outlet received 88000 units of L by selling A. Therefore the number of units of A sold 

$=\frac{88000}{110 m}=\frac{800}{m}$

From point 3, the number of units of L received by selling B = $\frac{9}{5}$ × 88000 = 158400

Therefore the number of units of B sold = $\frac{158400}{132 m}=\frac{1200}{m}$

From points 5 and 6, the number of units of A bought = 800 + $\frac{800}{m}$

Therefore, the number of units of L paid to buy A

= 95m $\left(800+\frac{800}{m}\right)$ = 76000 (1 + m)

From point 2, the number of units of L paid to buy B = 

$\frac{3}{5}$ × 76000 (1 + m) = 45600 (1 + m)

Therefore the number of units of B bought = $\frac{45600(1+m)}{114 m}=400+\frac{400}{m}$

From points 5 and 6,

$\frac{1200}{m}=400+\frac{400}{m}.$ Solving for m, m = 2

Therefore we have the following

​​​​​​​​​​​​​​

If the number of units of C sold = x, from points 5 and 6, the number of units of C bought = x + 3000.

Therefore, we get, 1.9(x+3000)=2.2x or solving for x, x = 19000.

Using the value of m, we get the following table for the number of units of A, B and C bought and sold.

​​​​​​​​​​​​​​

The base exchange rate of B w.r.t. L on that day was 240

Therefore the required answer is 240.

Answer: 240

Answer: Option C

Text Explanation :

Suppose the base exchange rates of A, B and C w.r.t L are 100m, 120m and m respectively.

Therefore we have the following:

​​​​​​​​​​​​​​

Given: The outlet received 88000 units of L by selling A. Therefore the number of units of A sold 

$=\frac{88000}{110 m}=\frac{800}{m}$

From point 3, the number of units of L received by selling B = $\frac{9}{5}$ × 88000 = 158400

Therefore the number of units of B sold = $\frac{158400}{132 m}=\frac{1200}{m}$

From points 5 and 6, the number of units of A bought = 800 + $\frac{800}{m}$

Therefore, the number of units of L paid to buy A

= 95m $\left(800+\frac{800}{m}\right)$ = 76000 (1 + m)

From point 2, the number of units of L paid to buy B = 

$\frac{3}{5}$ × 76000 (1 + m) = 45600 (1 + m)

Therefore the number of units of B bought = $\frac{45600(1+m)}{114 m}=400+\frac{400}{m}$

From points 5 and 6,

$\frac{1200}{m}=400+\frac{400}{m}.$ Solving for m, m = 2

Therefore we have the following

​​​​​​​​​​​​​​

If the number of units of C sold = x, from points 5 and 6, the number of units of C bought = x + 3000.

Therefore, we get, 1.9(x+3000)=2.2x or solving for x, x = 19000.

Using the value of m, we get the following table for the number of units of A, B and C bought and sold.

​​​​​​​​​​​​​​

The buying exchange rate of C w.r.t. L on that day was 1.90

Hence, option (c).

Answer: Option A

Text Explanation :

From points 1 and 2, the number of tickets bought by Young people = 80, the number of tickets bought by the middle aged people = 40 and the number of tickets bought by the old people = 20.

Using points 3 & 4, we get the following

​​​​​​​​​​​​​​

Now all the questions can be answered.

We have, 20 - 2y = x + 2y - 20. Therefore, x + 4y = 40.

Therefore, x = 40 - 4y = 4(10 - y)

or 2x = 8(10 - y). Therefore, x is a multiple of 8. Out of the given options, 32 is a possible answer.

Hence, option (a).

Answer: 3

Text Explanation :

We have, 20 − 2y = 17 − y or y = 3. Therefore the number of old visitors buying Gold tickets = 3.

Therefore the required answer is 3.

Answer: 3

Answer: 0

Text Explanation :

We have, y > 42 − x or x + y > 42. Now, the number of middle-aged visitors buying Gold tickets

= 43 − (x + y). Since x + y > 42, the number of middle-aged visitors buying Gold tickets = 0.

Therefore the required answer is 0.

Answer: 0

Answer: Option B

Text Explanation :

Option 1: The numbers of Middle-aged and Young visitors buying Gold tickets were equal i.e. 43 − x − y = 42 − x or y = 1. No condition precludes the possibility of y having a value of 1.

Option 2: The numbers of Old and Middle-aged visitors buying Economy tickets were equal i.e. y = 17 − y. This is not possible because in that case y will not be a natural number.
 
Option 3: The numbers of Gold and Platinum tickets bought by Young visitors were equal i.e. x = 42 − x i.e. x = 21. This is possible.

Option 4: The numbers of Old and Middle-aged visitors buying Platinum tickets were equal i.e. 20 - 2y = x + 2y - 20 or x + 4y = 40. This is possible.

Hence, option (b).

Answer: Option C

Text Explanation :

As per the data given in the question the gold coins were equally distributed amongst the 3 children. We are also told that the remaining assets (apart from the gold coins) are also equally distributed and that no flat or house is split or divided between 2 or more people. Only the bank deposit of Rs.70 lakhs can be split. Apart from the gold coins, the total value of the assets is 70 + 50 + 30 + 30 + 30 = 210 lakhs

So 210 lakhs split equally would means that each of the 3 daughters would get Rs.70 lakhs. This would mean that the person receiving the Rs.50 lakh house would get Rs.20 lakhs from the bank deposit. Now there are 3 flats of Rs.30 lakhs each and a balance of 50 lakhs in the bank deposit. The only way these assets can be split equally is if one of the 2 remaining people gets one of the flats of Rs.30 lakhs and receives 40 lakhs from the bank deposit. The second person would receive 70-40-20 = 10 Lakhs as bank deposit and 2 flats of 30 lakhs each, the sum of which adds upto 70 lakhs.
As Neeta receives the least amount in bank deposits, she will receive 10 lakhs. Also, as Geeta receives the highest amount in bank deposits she wil receive 40 lakhs. Therefore, Seeta will receive 20 lakhs from the bank deposit.

Hence, option (c).