LR - Venn Diagram - Previous Year CAT/MBA Questions
The best way to prepare for LR - Venn Diagram is by going through the previous year LR - Venn Diagram CAT questions. Here we bring you all previous year LR - Venn Diagram CAT questions along with detailed solutions.
Click here for previous year questions of other topics.
It would be best if you clear your concepts before you practice previous year LR - Venn Diagram CAT questions.
For Venn Diagram questions from Quant section, please visit this page.
Answer the following questions based on the information given below.
The chart below provides complete information about the number of countries visited by Dheeraj, Samantha and Nitesh, in Asia, Europe and the rest of the world (ROW).
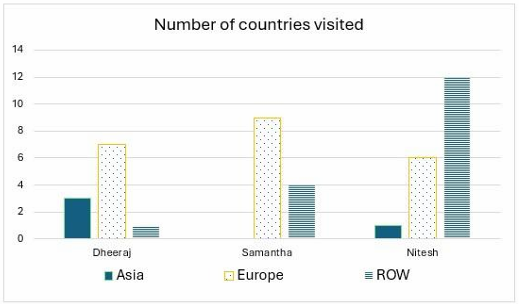
The following additional facts are known about the countries visited by them.
1. 32 countries were visited by at least one of them.
2. USA (in ROW) is the only country that was visited by all three of them.
3. China (in Asia) is the only country that was visited by both Dheeraj and Nitesh, but not by Samantha.
4. France (in Europe) is the only country outside Asia, which was visited by both Dheeraj and Samantha, but not by Nitesh.
5. Half of the countries visited by both Samantha and Nitesh are in Europe.
How many countries in Asia were visited by at least one of Dheeraj, Samantha and Nitesh?
Answer: 3
Text Explanation :
Workspace:
How many countries in Europe were visited only by Nitesh?
Answer: 2
Text Explanation :
Workspace:
How many countries in the ROW were visited by both Nitesh and Samantha?
Answer: 4
Text Explanation :
Workspace:
How many countries in Europe were visited by exactly one of Dheeraj, Samantha and Nitesh?
- (a)
12
- (b)
14
- (c)
5
- (d)
10
Answer: Option A
Text Explanation :
Workspace:
Directions for next 5 queestions.
There are 15 girls and some boys among the graduating students in a class. They are planning a get-together, which can be either a 1-day event, or a 2-day event, or a 3-day event. There are 6 singers in the class, 4 of them are boys. There are 10 dancers in the class, 4 of them are girls. No dancer in the class is a singer.
Some students are not interested in attending the get-together. Those students who are interested in attending a 3-day event are also interested in attending a 2-day event; those who are interested in attending a 2-day event are also interested in attending a 1-day event.
The following facts are also known:
1. All the girls and 80% of the boys are interested in attending a 1-day event. 60% of the boys are interested in attending a 2-day event.
2. Some of the girls are interested in attending a 1-day event, but not a 2-day event; some of the other girls are interested in attending both.
3. 70% of the boys who are interested in attending a 2-day event are neither singers nor dancers. 60% of the girls who are interested in attending a 2-day event are neither singers nor dancers.
4. No girl is interested in attending a 3-day event. All male singers and 2 of the dancers are interested in attending a 3-day event.
5. The number of singers interested in attending a 2-day event is one more than the number of dancers interested in attending a 2-day event.
How many boys are there in the class?
Answer: 50
Text Explanation :
There are boys and girls in the class. Each of the boy or girl is either a dance or singer or none of these but not both.
There are 6 singers in the class, 4 of them are boys.
⇒ There are 4 male singers and 2 female singers
There are 10 dancers in the class, 4 of them are girls. No dancer in the class is a singer
⇒ There are 6 male dances and 4 female dancers

From (4): No girl is interested in attending a 3-day event. All male singers and 2 of the dancers are interested in attending a 3-day event.
Since all 4 male singers are interested in a 3-day event, they all must also be interested in a 2-day and a 1-day event.
Since 2 male dancers are interested in a 3-day event, male dancers interested in a 2-day event must be greater than or equal to 2.

From (1): All the girls and 80% of the boys are interested in attending a 1-day event. 60% of the boys are interested in attending a 2-day event.
From (3): 70% of the boys who are interested in attending a 2-day event are neither singers nor dancers. Hence, 30% of those who are interested in a 2-day event are dancers or singers.

Now, (2 + b) = 30% of 60% of (10 + x)
⇒ 2 + b + 4 = × × (10 + x)
⇒ 6 + b = × (10 + x)
Here b has to be an integer, hence (10 + x) should be completely divisible by 50.
∴ x should be 40 or 90 or 140 and so on.
But since b cannot be greater than 4, x should be 40 and b = 3.
From (5): The number of singers interested in attending a 2-day event is one more than the number of dancers interested in attending a 2-day event.
This is only possible when 2 female singers while no female dance is interested in a 2-day event.

From (3): 60% of the girls who are interested in attending a 2-day event are neither singers nor dancers.
⇒ 0 + 2 = × (0 + 2 + females interested in a 2-day event who are neither singer nor dancer)
females interested in a 2-day event who are neither singer nor dancer = 3

∴ There are 50 boys in the class.
Hence, 50.
Workspace:
Which of the following can be determined from the given information?
I. The number of boys who are interested in attending a 1-day event and are neither dancers nor singers.
II. The number of female dancers who are interested in attending a 1-day event.
- (a)
Only II
- (b)
Neither I nor II
- (c)
Only I
- (d)
Both I and II
Answer: Option A
Text Explanation :
Consider the solution to first question of this set.
I. The number of boys who are interested in attending a 1-day event and are neither dancers nor singers – we don’t this data yet.
II. The number of female dancers who are interested in attending a 1-day event - 5
Hence, option (a).
Workspace:
What fraction of the class are interested in attending a 2-day event?
- (a)
2/3
- (b)
7/10
- (c)
7/13
- (d)
9/13
Answer: Option C
Text Explanation :
Consider the solution to first question of this set.
Total students in the class = 15 + 50 = 65
Total students interested in a 2-day event = 5 + 30 = 35
∴ Required fraction = 35/65 = 7/13
Hence, option (c).
Workspace:
What BEST can be concluded about the number of male dancers who are interested in attending a 1-day event?
- (a)
5 or 6
- (b)
5
- (c)
6
- (d)
4 or 6
Answer: Option A
Text Explanation :
Consider the solution to first question of this set.
Number of male dancers who are interested in attending a 1-day event has to be more than or equal to number of male dancers who are interested in attending a 2-day event i.e., 5 or 6.
Hence, option (a).
Workspace:
How many female dancers are interested in attending a 2-day event?
- (a)
0
- (b)
1
- (c)
2
- (d)
Cannot be determined
Answer: Option A
Text Explanation :
Consider the solution to first question of this set.
Female dancers are interested in attending a 2-day event = 0.
Hence, option (a).
Workspace:
Answer the next 5 questions based on the information given below:
A speciality supermarket sells 320 products. Each of these products was either a cosmetic product or a nutrition product. Each of these products was also either a foreign product or a domestic product. Each of these products had at least one of the two approvals – FDA or EU.
The following facts are also known:
1. There were equal numbers of domestic and foreign products.
2. Half of the domestic products were FDA approved cosmetic products.
3. None of the foreign products had both the approvals, while 60 domestic products had both the approvals.
4. There were 140 nutrition products, half of them were foreign products.
5. There were 200 FDA approved products. 70 of them were foreign products and 120 of them were cosmetic products.
How many foreign products were FDA approved cosmetic products?
Answer: 40
Text Explanation :
There were equal numbers of domestic and foreign products.
∴ There will be 160 foreign as well as domestic products.

Half of the domestic products were FDA approved cosmetic products.
∴ 80 products are domestic, FDA approved and cosmetic products.

None of the foreign products had both the approvals while 60 domestic products had both the approvals.

There were 140 nutrition products, half of them were foreign products.
∴ Number of cosmetic products = 320 – 140 = 180

There were 200 FDA approved products, 70 of them were foreign products
FDA approved products = FDA foreign + FDA domestic
⇒ 200 = 70 + (60 + only FDA domestic products)
⇒ only FDA domestic products = 70

and 120 of them were cosmetic products.
FDA approved cometic products = FDA approved foreign cosmetic + FDA approved domestic cosmetic
⇒ 120 = (0 + only FDA approved cosmetic foreign products) + 80
⇒ only FDA approved cosmetic foreign products = 40
Since there are total 70 foreign FDA approved products out of which 40 are only FDA approved cosmetic foreign products, hence approved nutrition foreign products = 70 – 40 = 30.
⇒ only EU approved nutrition foreign products = 70 – 30 = 40

Total foreign products = 160
⇒ only EU approved cosmetic foreign products = 160 – 40 – 30 – 40 = 50

Total Cosmetic products is 180 = cosmetic foreign + cosmetic domestic
⇒ 180 = (50 + 0 + 40) + (only EU approved domestic cosmetic products + 80)
⇒ only EU approved domestic cosmetic products = 10

Total domestic products = 160 = (10 + only EU nutrition domestic products) + 60 + 70
⇒ only EU nutrition domestic products = 20

With the given information we can tabulate this much.
∴ Number of foreign FDA approved cosmetic products = 0 + 40 = 40
Hence, 40.
Workspace:
How many cosmetic products did not have FDA approval?
- (a)
60
- (b)
10
- (c)
50
- (d)
Cannot be determined
Answer: Option A
Text Explanation :
Consider the solution to first question of this set.
Number of cosmetic products that did not have FDA approval = 50 + 10 = 60
Hence, option (a).
Workspace:
Which among the following options best represents the number of domestic cosmetic products that had both the approvals?
- (a)
At least 20 and at most 70
- (b)
At least 10 and at most 60
- (c)
At least 20 and at most 50
- (d)
At least 10 and at most 80
Answer: Option B
Text Explanation :
Consider the solution to first question of this set.
Domestic products which have both approvals = 60.
∴ Domestic cosmetic products with both approvals cannot be more than 60.
Maximum only FDA approved cosmetic domestic products can be 70, hence minimum cosmetic domestic products with both approvals can be 10.
Hence, option (b).
Workspace:
If 70 cosmetic products did not have EU approval, then how many nutrition products had both the approvals?
- (a)
30
- (b)
50
- (c)
10
- (d)
20
Answer: Option C
Text Explanation :
Consider the solution to first question of this set.
We have:

If 70 cosmetic products did not have EU approval, then number of nutrition products with both approvals = only FDA approved cosmetic (foreign + domestic) products
⇒ 70 = 40 + only FDA approved cosmetic domestic products
⇒ only FDA approved cosmetic domestic products = 30
We can fill the remaining table as follows.

Number of nutrition products with both approvals = 0 + 10 = 10
Hence, option (c).
Workspace:
If 50 nutrition products did not have EU approval, then how many domestic cosmetic products did not have EU approval?
Answer: 50
Text Explanation :
Consider the solution to first question of this set.
We have:

50 nutrition products did not have EU approval = 30 + only FDA domestic nutrition products
⇒ only FDA domestic nutrition products = 20
We can fill the table as follows:
We have:

∴ Number of domestic cosmetic products without EU approval = 50
Hence, 50.
Workspace:
Answer the next 4 questions based on the information given below.
1000 patients currently suffering from a disease were selected to study the effectiveness of treatment of four types of medicines — A, B, C and D. These patients were first randomly assigned into two groups of equal size, called treatment group and control group. The patients in the control group were not treated with any of these medicines; instead they were given a dummy medicine, called placebo, containing only sugar and starch. The following information is known about the patients in the treatment group.
- A total of 250 patients were treated with type A medicine and a total of 210 patients were treated with type C medicine.
- 25 patients were treated with type A medicine only. 20 patients were treated with type C medicine only. 10 patients were treated with type D medicine only.
- 35 patients were treated with type A and type D medicines only. 20 patients were treated with type A and type B medicines only. 30 patients were treated with type A and type C medicines only. 20 patients were treated with type C and type D medicines only.
- 100 patients were treated with exactly three types of medicines.
- 40 patients were treated with medicines of types A, B and C, but not with medicines of type D. 20 patients were treated with medicines of types A, C and D, but not with medicines of type B.
- 50 patients were given all the four types of medicines. 75 patients were treated with exactly one type of medicine.
How many patients were treated with medicine type B?
Answer: 340
Text Explanation :
We can draw the following Venn diagram according to the information given in the question.

75 patients were treated with exactly one type of medicine.
∴ 25 + a + 20 + 10 = 75
⇒ a = 20
250 patients were treated with type A medicine
∴ 25 + 30 + 20 + 35 + 20 + 40 + 50 + e = 250.
⇒ e = 30
100 patients were treated with exactly three types of medicines.
∴ 20 + 40 + c + e = 100
⇒ c = 10
210 patients were treated with type C medicine
∴ 30 + 40 + b + 20 + 20 + 50 + c + 20 = 210
⇒ b = 20
Since patients were equally in treatment group and control group hence, there were total 500 patients who were in the treatment group and 500 in control group (who were not given any medicine).
Total patient in treatment group = 500 = 25 + 30 + 20 + 35 + 20 + 40 + 50 + e + a + b + c + d + 20 + 20 + 10.
⇒ d = 150
The completed Venn diagram:
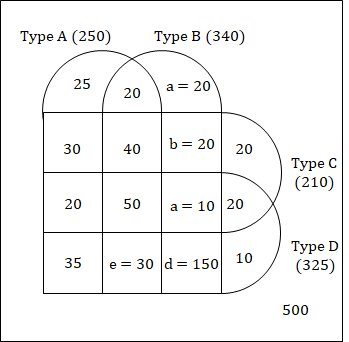
∴ 340 patients were treated with Type B medicine.
Hence, 340.
Workspace:
The number of patients who were treated with medicine types B, C and D, but not type A was:
Answer: 10
Text Explanation :
Consider the solution to the first question of this set.
The number of patients who were treated with medicine types B, C and D, but not type A is a = 10.
Hence, 10.
Workspace:
How many patients were treated with medicine types B and D only?
Answer: 150
Text Explanation :
Consider the solution to the first question of this set.
The number of patients who were treated with medicine types B and D only = d = 150.
Hence, 150.
Workspace:
The number of patients who were treated with medicine type D was:
Answer: 325
Text Explanation :
Consider the solution to the first question of this set.
The number of patients who were treated with medicine type D was 325.
Hence, 325.
Workspace:
Answer the next 4 questions based on the information given below.
Ten musicians (A, B, C, D, E, F, G, H, I and J) are experts in at least one of the following three percussion instruments: tabla, mridangam, and ghatam. Among them, three are experts in tabla but not in mridangam or ghatam, another three are experts in mridangam but not in tabla or ghatam, and one is an expert in ghatam but not in tabla or mridangam. Further, two are experts in tabla and mridangam but not in ghatam, and one is an expert in tabla and ghatam but not in mridangam.
The following facts are known about these ten musicians.
- Both A and B are experts in mridangam, but only one of them is also an expert in tabla.
- D is an expert in both tabla and ghatam.
- Both F and G are experts in tabla, but only one of them is also an expert in mridangam.
- Neither I nor J is an expert in tabla.
- Neither H nor I is an expert in mridangam, but only one of them is an expert in ghatam.
Who among the following is DEFINITELY an expert in tabla but not in either mridangam or ghatam?
- (a)
F
- (b)
A
- (c)
H
- (d)
C
Answer: Option C
Text Explanation :
We can draw the following Venn diagram based on the information given in the main paragraph.

D is an expert in both tabla and ghatam.
Since I is neither an expert in tabla nor in mridangam hence, he is an expert in ghatam
∴ D and I are the only two experts in ghatam.
Now, J is not an expert in tabla hence, he can only be an expert in mridangam only.
Also, H is not an expert in mridangam hence, he can only be an expert in tabla only.
Both A and B are experts in mridangam, but only one of them is also an expert in tabla.
Both F and G are experts in tabla, but only one of them is also an expert in mridangam.
There are two experts in tabla and mridangam only one of them is either A or B and the other is either F or G.
∴ We can make the following Venn diagram

∴ H is an expert in tabla only.
Hence, option (c).
Workspace:
Who among the following is DEFINITELY an expert in mridangam but not in either tabla or ghatam?
- (a)
E
- (b)
B
- (c)
G
- (d)
J
Answer: Option D
Text Explanation :
Consider the solution to the first question of this set.
J is an expert in mridangam only.
Hence, option (d).
Workspace:
Which of the following pairs CANNOT have any musician who is an expert in both tabla and mridangam but not in ghatam?
- (a)
C and E
- (b)
C and F
- (c)
A and B
- (d)
F and G
Answer: Option A
Text Explanation :
Consider the solution to the first question of this set.
Neither C nor E can be an expert in both tabla and mridangam.
Hence, option (a).
Workspace:
If C is an expert in mridangam and F is not, then which are the three musicians who are experts in tabla but not in either mridangam or ghatam?
- (a)
C, E and G
- (b)
E, F and H
- (c)
C, G and H
- (d)
E, G and H
Answer: Option B
Text Explanation :
Consider the solution to the first question of this set.
If C is an expert in mridangam and F is not, we can make the following Venn diagram.
We can make the following Venn diagram

∴ E, F and H are experts in tabla but neither mridangam or ghatam.
Hence, option (b).
Workspace:
Answer the next 4 questions based on the information given.
A survey of 600 schools in India was conducted to gather information about their online teaching learning processes (OTLP). The following four facilities were studied.
F1: Own software for OTLP
F2: Trained teachers for OTLP
F3: Training materials for OTLP
F4: All students having Laptops
The following observations were summarized from the survey.
- 80 schools did not have any of the four facilities – F1, F2, F3, F4.
- 40 schools had all four facilities.
- The number of schools with only F1, only F2, only F3, and only F4 was 25, 30, 26 and 20 respectively.
- The number of schools with exactly three of the facilities was the same irrespective of which three were considered.
- 313 schools had F2.
- 26 schools had only F2 and F3 (but neither F1 nor F4).
- Among the schools having F4, 24 had only F3, and 45 had only F2.
- 162 schools had both F1 and F2.
- The number of schools having F1 was the same as the number of schools having F4.
What was the total number of schools having exactly three of the four facilities?
- (a)
64
- (b)
80
- (c)
50
- (d)
200
Answer: Option D
Text Explanation :
Given, the number of schools with exactly three of the facilities was the same irrespective of which three were considered.
Let us assume this number to be ‘a’ for every possible combination of three OTLPs.
The following diagram can be drawn from the given information.
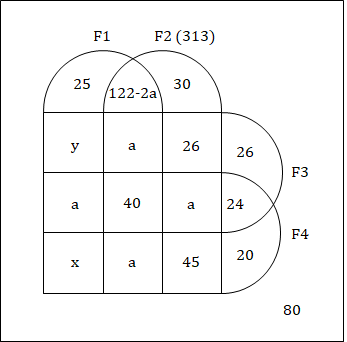
It is also given that 162 schools had F1 and F2
∴ Number of students having only F1 and F2 = 162 – (a + 40 + a) = 122 – 2a.
Total schools having F2 = 313 = 162 + 30 + 26 + a + 45
⇒ a = 50
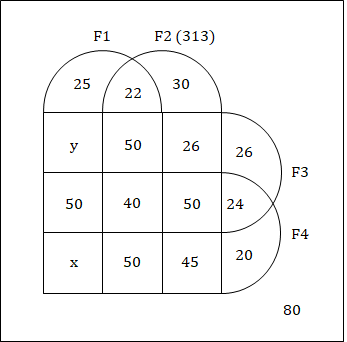
Total number of schools having F1 is equal to total number of schools having F2.
∴ (162 + 25 + y + 50 + x) = (50 + 40 + 50 + 24 + x + 50 + 45 + 20)
⇒ y = 42
Now there are a total of 600 schools
∴ 600 = 25 + 42 + 50 + x + 313 + 26 + 24 + 20 + 80
⇒ x = 20
Therefore, the complete Venn diagram is
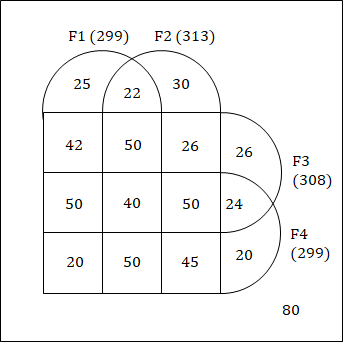
Number of schools having exactly 3 of the 4 facilities = 50 + 50 + 50 + 50 = 200
Hence, option (d).
Workspace:
What was the number of schools having facilities F2 and F4?
- (a)
85
- (b)
45
- (c)
95
- (d)
185
Answer: Option D
Text Explanation :
Consider the solution to the first question of this set.
Number of schools having facilities F2 and F4 = 40 + 50 + 50 + 45 = 185
Hence, option (d).
Workspace:
What was the number of schools having only facilities F1 and F3?
Answer: 42
Text Explanation :
Consider the solution to the first question of this set.
Number of schools having only facilities F1 and F3 = 42
Hence, 42.
Workspace:
Feedback
Help us build a Free and Comprehensive Preparation portal for various competitive exams by providing us your valuable feedback about Apti4All and how it can be improved.
 Solution
Solution Discuss
Discuss Report
Report