LR - Venn Diagram - Previous Year CAT/MBA Questions
The best way to prepare for LR - Venn Diagram is by going through the previous year LR - Venn Diagram CAT questions. Here we bring you all previous year LR - Venn Diagram CAT questions along with detailed solutions.
Click here for previous year questions of other topics.
It would be best if you clear your concepts before you practice previous year LR - Venn Diagram CAT questions.
For Venn Diagram questions from Quant section, please visit this page.
What was the number of schools having only facilities F1 andF4?
Answer: 20
Text Explanation :
Consider the solution to the first question of this set.
Number of schools having only facilities F1 and F4 = 20.
Hence, 20.
Workspace:
Answer the following question based on the information given below.
1600 satellites were sent up by a country for several purposes. The purposes are classified as broadcasting (B), communication (C), surveillance (S), and others (O). A satellite can serve multiple purposes; however a satellite serving either B, or C, or S does not serve O.
The following facts are known about the satellites:
- The numbers of satellites serving B, C, and S (though may be not exclusively) are in the ratio 2 : 1 : 1.
- The number of satellites serving all three of B, C, and S is 100.
- The number of satellites exclusively serving C is the same as the number of satellites exclusively serving S. This number is 30% of the number of satellites exclusively serving B.
- The number of satellites serving O is the same as the number of satellites serving both Cand S but not B.
What best can be said about the number of satellites serving C?
- (a)
Cannot be more than 800
- (b)
Must be at least 100
- (c)
Must be between 450 and 725
- (d)
Must be between 400 and 800
Answer: Option C
Text Explanation :
Let number of satellites exclusively serving B be ‘10x’ and number of satellites serving O be ‘z’.
∴ number of satellites exclusively serving C = number of satellites exclusively serving S = 3a
As the number of satellites serving C = number of satellites serving S, the number of satellites serving C and B but not S = number of satellites exclusively serving S and B but not C = y (assume).

∴ 10x + 2y + 100 + 6x + 2z
= 1600 ⇒ 8x + y + z
= 750 … (i)
From (1), 10x + 2y + 100
= 6x + 2y + 2z + 200
∴ z = 2x – 50 … (ii)
Thus, the minimum value that ‘x’ can takes is 25.
From (i) and (ii),
10x + y = 800 ⇒ y = 800 – 10x
Thus, the maximum value that ‘x’ can takes is 80.
The number of satellites serving
C = 3x + y + z + 100 = 3x + (800 – 10x) + (2x – 50) + 100 = 850 – 5x
As value of ‘x’ is between 25 and 80, value of (850 – 5x) must be between 450 and 725.
Hence, option (c).
Workspace:
What is the minimum possible number of satellites serving B exclusively?
- (a)
100
- (b)
200
- (c)
250
- (d)
500
Answer: Option C
Text Explanation :
The number of satellites serving B exclusively = 10x.
As minimum value of ‘x’ is 25, minimum value of 10x is 250.
Hence, option (c).
Workspace:
If at least 100 of the 1600 satellites were serving O, what can be said about the number of satellites serving S?
- (a)
Exactly 475
- (b)
At most 475
- (c)
At least 475
- (d)
No conclusion is possible based on the given information
Answer: Option B
Text Explanation :
Number of satellites serving S = 3x + y + z + 100 = 3x + (800 – 10x) + (2x – 50) + 100
= 850 – 5x
2x – 50 ≥ 100 ⇒ x ≥ 75 ⇒ (–5x) ≤ (–375)
∴ 850 – 5x ≤ 850 – 375 = 475
Thus, the number of satellites serving S can be at most 475.
Hence, option (b).
Workspace:
If the number of satellites serving at least two among B, C, and S is 1200, which of the following MUST be FALSE?
- (a)
All 1600 satellites serve B or C or S
- (b)
The number of satellites serving B is more than 1000
- (c)
The number of satellites serving C cannot be uniquely determined
- (d)
The number of satellites serving B exclusively is exactly 250
Answer: Option C
Text Explanation :
The number of satellites serving at least two among B, C and S is 1200
i.e., 2y + z + 100 ≥ 1200
⇒ 1600 – 20x + 2x – 50 ≥ 1100
⇒ 450 ≥ 18x
⇒ 25 ≥ x
Earlier we have seen that the minimum value of ‘x’ is 25
∴ x = 25
[1]: z = 2x – 50 = 0 ⇒ All satellites serve B or C or S. Therefore, statement 1 is true.
[2]: The number of satellites serving B = 10x + 2y + 100 = 10(25) + 2(800 – 10x) + 100
= 1450
Therefore, statement 2 is true.
[3]: The number of satellites serving C
= 3x + y + z + 100 = 3x + (800 – 10x) + (2x – 50) + 100 = 850 – 5x = 725
Therefore, statement 3 is false.
[4]: The number of satellites serving B exclusively = 10x = 10 × 25 = 250
Therefore, statement 4 is true.
Hence, option (c).
Workspace:
Answer the following question based on the information given below.
Fun Sports (FS) provides training in three sports – Gilli-danda (G), Kho-Kho (K), and Ludo (L). Currently it has an enrollment of 39 students each of whom is enrolled in at least one of the three sports. The following details are known:
- The number of students enrolled only in L is double the number of students enrolled in all the three sports.
- There are a total of 17 students enrolled in G.
- The number of students enrolled only in G is one less than the number of students enrolled only in L.
- The number of students enrolled only in K is equal to the number of students who are enrolled in both K and L.
- The maximum student enrollment is in L.
- Ten students enrolled in G are also enrolled in at least one more sport.
What is the minimum number of students enrolled in both G and L but not in K?
Answer: 4
Text Explanation :

Since 10 students who play G enrolled in at least one other sport, the number of students who enrolled only in G = 17 - 10 = 7. Therefore x = 7.
Also from statement 1, the number of students = who enrolled in
all the three sports = 4
As 17 students enrolled in G, the number of students who did not enrol in G = 39 - 17 = 22.
Therefore, x + 1 + z + y + z = 22. We know that x = 7 and y = 4. Therefore we have the following: 8 + 4 + 2z = 22 or z = 5.

From statement 5, 6-w>wor w=0 or 1 or 2.
Now first two questions can be answered.
The required answer is 6 - 2 = 4.
Answer: 4
Workspace:
If the numbers of students enrolled in K and L are in the ratio 19:22, then what is the number of students enrolled in L?
- (a)
17
- (b)
19
- (c)
22
- (d)
18
Answer: Option C
Text Explanation :
If the ratio of the numbers of students enrolled in K and L are in the ratio 19:22,
Therefore w = 1.
Therefore total enrollment in L
= 23 − 1 = 22.
Hence, option (c).
Workspace:
Due to academic pressure, students who were enrolled in all three sports were asked to withdraw from one of the three sports. After the withdrawal, the number of students enrolled in G was six less than the number of students enrolled in L, while the number of students enrolled in K went down by one. After the withdrawal, how many students were enrolled in both G and K?
Answer: 2
Text Explanation :
Out of 4 students who are enrolled in all the three, suppose ‘a’ students dropped out of L and ‘b’ students dropped out of K. Therefore the number of students who dropped out of G = 4 - a - b.
Therefore we have the following:

If the number of students enrolled in K reduced by 1 that means out of the 4 students who had enrolled in all the three, one student dropped out of K i.e. b = 1.
Now, if the number of students enrolled in G was 6 less than the number of students enrolled in L, we have the following:
(7 + w + a + 6 − w + b) + 6
= 6 − w + b + 9 − a − b + 8
∴19 + a + b = 23 − w − a
∴2a + b + w = 4
Since b = 1, the only solution for the equation 2a + b + w = 4 is a = 1, b = 1 and w = 1.
Now both the questions can be answered.
The required number of students = w + a = 2.
Answer: 2
Workspace:
Due to academic pressure, students who were enrolled in all three sports were asked to withdraw from one of the three sports. After the withdrawal, the number of students enrolled in G was six less than the number of students enrolled in L, while the number of students enrolled in K went down by one. After the withdrawal, how many students were enrolled in both G and L?
- (a)
7
- (b)
5
- (c)
8
- (d)
6
Answer: Option D
Text Explanation :
The required number of students
= 6 − w + b = 6 − 1 + 1 = 6.
Hence, option (d).
Workspace:
Answer the following question based on the information given below.
Applicants for the doctoral programmes of Ambi Institute of Engineering (AIE) and Bambi Institute of Enginneering (BIE) have to appear for a Common Entrance Test (CET). The test has three sections: Physics (P), Chemistry (C), and Maths (M). Among those appearing for CET, those at or above the 80th percentile in at least two sections, and at or above the 90th percentile overall, are selected for Advanced Entrance Test (AET) conducted by AIE. AET is used by AIE for final selection.
For the 200 candidates who are at or above the 90th percentile overall based on CET, the following are known about their performance in CET:
- No one is below the 80th percentile in all 3 sections.
- 150 are at or above the 80th percentile in exactly two sections.
- The number of candidates at or above the 80th percentile only in P is the same as the number of candidates at or above the 80th percentile only in C. The same is the number of candidates at or above the 80th percentile only in M.
- Number of candidates below 80th percentile in P : Number of candidates below 80th percentile in C : Number of candidates below 80th percentile in M = 4 : 2 : 1.
BIE uses a different process for selection. If any candidates is appearing in the AET by AIE, BIE consider their AET score for final selection provided the candidates is at or above the 80th percentile in P. Any other candidate at or above the 80th percentile in P in CET, but who is not eligible for AET, is required to appear in a separate test to be conducted by BIE for being considered for final selection. Altogether, there are 400 candidates this year who are at or above the 80th percentile in P.
What best can be concluded about the number of candidates sitting for the separate test for BIE who were at or above the 90th percentile overall in CET?
- (a)
3 or 10
- (b)
10
- (c)
5
- (d)
7 or 10
Answer: Option A
Text Explanation :
Using the information given in the question let us represent it in the Venn diagram shown below. The diagram depicts the number of candidates getting 80 percentile and above in at least one or more of the subjects amongst students getting 90 percentile overall.

The number of candidates scoring 80 percentile and above in only Physics, only Chemistry and only Math is the same. Let this be ‘d’
Let ‘a’ – number of candidates scoring 80 percentile and above only in Physics and Math.
Let ‘b’ – number of candidates scoring 80 percentile above only in Physics and Chemistry.
Let ‘c’ – number of candidates scoring 80 percentile and above in Chemistry and Math.
Let ‘e’ – number of candidates scoring 80 percentile and above in all 3 subjects.
a + b + c = 150
Also a + b + c + 3d + e = 200
⇒ 3d + e = 50
Given that (2d + c) : (2d + a) : (2d + b) = 4 : 2 : 1
This implies 6d + a + b + c is a multiple of 7. We already know that a + b + c = 150. So 6d + 150 is a multiple of 7. This implies that 6d + 3 will also be a multiple of 7. So d will be 3, 10, 17. But as 3d + e = 50, it implies that d < 17. So d will be either 3 or 10.
The number of students who scored 90 percentile and above and scored at least 80 percentile in Physics (but not in Chemistry and Math) will be eligible for the BIE entrance test. This is equal to d which is either 3 or 10.
Hence, option (a).
Workspace:
If the number of candidates who are at or above the 90th percentile overall and also at or above the 80th percentile in all three sections in CET is actually a multiple of 5, what is the number of candidates who are at or above the 90th percentile overall and at or above the 80th percentile in both P and M in CET?
Answer: 60
Text Explanation :
Using the information given in the question let us represent it in the Venn diagram shown below. The diagram depicts the number of candidates getting 80 percentile and above in at least one or more of the subjects amongst students getting 90 percentile overall.

The number of candidates scoring 80 percentile and above in exactly each of Physics, chemistry and Math is the same. Let this be ‘d’
Let ‘a’ – number of candidates scoring 80 percentile and above only in Physics and Math.
Let ‘b’ – number of candidates scoring 80 percentile above only in Physics and Chemistry.
Let ‘c’ – number of candidates scoring 80 percentile and above in Chemistry and Math.
Let ‘e’ – number of candidates scoring 80 percentile and above in all 3 subjects.
a + b + c = 150
Also a + b + c + 3d + e = 200
⇒3d + e=50
Given that (2d + c) : (2d + a) : (2d + b) = 4: 2: 1
This implies 6d + a + b + c is a multiple of 7. We already know that a + b + c= 150.
So 6d + 150 is a multiple of 7. This implies that 6d + 3 will also be a multiple of 7. So d will be 3, 10, 17. But as 3d + e = 50, it implies that d < 17. So d will be either 3 or 10.
Now 3d + e = 50
Also, d = 3 or 10
But it is given that e is a multiple of 5, so
e = 20
Now and
Solving the above expression we get the following equations:
c – 2a = 20 … (I)
c – 4b = 60 … (II)
a – 2b = 20 … (III)
Adding (I), (II) and (III) we get
–5b + 3c = 250 … (VI)
Solving (II) and (VI) we get
b = 10 and c = 100
∴ a =150 – 10 – 100 = 40
Now the number of candidates who scored 90 percentile overall and above and who score 80 percentile and above in P and M is a + e = 40 + 20 = 60
Answer : 60
Workspace:
If the number of candidates who are at or above the 90th percentile overall and also at or above the 80th percentile in all three sections in CET is actually a multiple of 5, then how many candidates were shortlisted for the AET for AIE?
Answer: 70
Text Explanation :
Using the information given in the question let us represent it in the Venn diagram shown below. The diagram depicts the number of candidates getting 80 percentile and above in at least one or more of the subjects amongst students getting 90 percentile overall.

The number of candidates scoring 80 percentile and above in exactly each of Physics, chemistry and Math is the same. Let this be ‘d’
Let ‘a’ – number of candidates scoring 80 percentile and above only in Physics and Math.
Let ‘b’ – number of candidates scoring 80 percentile above only in Physics and Chemistry.
Let ‘c’ – number of candidates scoring 80 percentile and above in Chemistry and Math.
Let ‘e’ – number of candidates scoring 80 percentile and above in all 3 subjects.
a +b + c = 150
Also a + b + c + 3d + e = 200
⇒3d + e=50
Given that (2d + c) : (2d + a) : (2d + b)
= 4: 2: 1
This implies 6d + a + b + c is a multiple of 7. We already know that a + b + c= 150.
So 6d + 150 is a multiple of 7. This implies that 6d + 3 will also be a multiple of 7. So d will be 3, 10, 17. But as 3d + e = 50, it implies that d < 17. So d will be either 3 or 10.
Since the number of candidates who are at 90 percentile and above and also at or 80th percentile in all 3 sections is a multiple of 5 (which in the Venn Diagram is ‘e’), the Value of e(as explained in the previous questions) will have to be 20. Now the number of candidates who get 80 percentile in atleast 2 out of P, C and M which in the Venn Diagram will be the sum of a, b, c and e.
We already know a + b + c = 150 and e = 20
∴a + b + c + e = 150 + 20=70
Answer: 170
Workspace:
If the number of candidates who are at or above the 90th percentile overall and also are at or above the 80th percentile in P in CET, is more than 100, how many candidates had to sit for the separate test for BIE?
- (a)
299
- (b)
310
- (c)
321
- (d)
330
Answer: Option A
Text Explanation :
Using the information given in the question let us represent it in the Venn diagram shown below. The diagram depicts the number of candidates getting 80 percentile and above in at least one or more of the subjects amongst students getting 90 percentile overall.

The number of candidates scoring 80 percentile and above in exactly each of Physics, chemistry and Math is the same. Let this be ‘d’
Let ‘a’ – number of candidates scoring 80 percentile and above only in Physics and Math.
Let ‘b’ – number of candidates scoring 80 percentile above only in Physics and Chemistry.
Let ‘c’ – number of candidates scoring 80 percentile and above in Chemistry and Math.
Let ‘e’ – number of candidates scoring 80 percentile and above in all 3 subjects.
a +b + c = 150
Also a + b + c + 3d + e = 200
⇒3d+e=50
Given that (2d + c) : (2d + a) : (2d + b)
= 4: 2: 1
This implies 6d + a + b + c is a multiple of 7. We already know that a + b + c= 150.
So 6d + 150 is a multiple of 7. This implies that 6d + 3 will also be a multiple of 7. So d will be 3, 10, 17. But as 3d + e = 50, it implies that d < 17. So d will be either 3 or 10.
The number of candidates who are at or above 90th percentile overall and also at or above 80th percentile in P (as indicated in the Venn diagram) = a + b + d + e. As indicated in the answers to the previous question a = 3 or 10. Now we have already seen that if d = 10, a = 40, e As indicated in the answers to the previous question a = 3 or 10. Now we have So then a + b + d + e
= 42 + 18 + 3 + 41 = 104, which satisfies the condition given in the question.
Now the number of candidates who appear separately for the BIE test will be those who got 90 percentile and above overall and got 80 percentile and above only in P plus there who got 80 percentile only in P but got less than 90th percentile overall. Candidates who got 80th percentile only in P and got less than 90 in percentile overall = 400 – 104 =296
Number of candidates who get 80 percentile and above only in P and got 90 in percentile and above overall = d = 3
So, the number of candidates who sit for the separate test for BIE = 296 + 3 = 299
Hence, option (a).
Workspace:
Answer the following question based on the information given below.
Help Distress (HD) is an NGO involved in providing assistance to people suffering from natural disasters. Currently, it has 37 volunteers. They are involved in three projects: Tsunami Relief (TR) in Tamil Nadu, FloodRelief (FR) in Maharashtra, and Earthquake Relief (ER) in Gujarat. Each volunteer working with Help Distress has to be involved in at least one relief work project.
- A Maximum number of volunteers are involved in the FR project. Among them, the number of volunteers involved in FR project alone is equal to the volunteers having additional involvement in the ER project.
- The number of volunteers involved in the ER project alone is double the number of volunteers involved in all the three projects.
- 17 volunteers are involved in the TR project.
- The number of volunteers involved in the TR project alone is one less than the number of volunteers involved in ER Project alone.
- Ten volunteers involved in the TR project are also involved in at least one more project.
Based on the information given above, the minimum number of volunteers involved in both FR and TR projects, but not in the ER project is:
- (a)
1
- (b)
3
- (c)
4
- (d)
5
Answer: Option C
Text Explanation :

17 volunteers are involved in the TR project and 10 in TR are also involved in other projects. Thus, 7 volunteers are involved only in TR.
∴ 8 volunteers are involved in ER alone.
∴ 4 volunteers are involved in all the three projects.
Let x people be involved in FR alone.
∴ Number of people involved in FR and ER but not TR = x – 4
Now, a + b + 4 = 10
∴ a + b = 6
Also, 7 + a + b + 4 + x + x – 4 + 8 = 37
∴ 2x = 16 or x = 8
Number of Volunteers involved in FR > Number of Volunteers involved in TR
And Number of Volunteers involved in FR > Number of Volunteers involved in ER
∴ 16 + a > 17 and 16 + a > 16 + b or a > b
∴ a and b can be (6, 0), (5, 1), (4, 2)
The minimum number of volunteers involved in both FR and TR projects, but not in the ER Project = minimum value of a = 4
Hence, option (c).
Workspace:
Which of the following additional information would enable to find the exact number of volunteers involved in various projects?
- (a)
Twenty volunteers are involved in FR.
- (b)
Four volunteers are involved in all the three projects.
- (c)
Twenty three volunteers are involved in exactly one project.
- (d)
No need for any additional information.
Answer: Option A
Text Explanation :
We can obtain the information in options 2 and 3 from the initial data.
Based on the information given in the explanation to the first question, the information in option 1 will give us the value of a, which in turn will give us the value of b. Thus, option 1 would enable us to find the exact number of volunteers involved in various projects.
Hence, option (a).
Workspace:
After some time, the volunteers who were involved in all the three projects were asked to withdraw from one project. As a result, one of the volunteers opted out of the TR project, and one opted out of the ER project, while the remaining ones involved in all the three projects opted out of the FR project. Which of the following statements, then, necessarily follows?
- (a)
The lowest number of volunteers is now in TR project.
- (b)
More volunteers are now in FR project as compared to ER project.
- (c)
More volunteers are now in TR project as compared to ER project.
- (d)
None of the above
Answer: Option B
Text Explanation :

After the volunteers withdraw as mentioned, the number of volunteers working on different projects is as shown.
∴ Number of volunteers working on TR = 7 + 6 + 3 = 16
Number of volunteers working on FR = 14 + a
Number of volunteers working on ER = 15 + b
Considering the possible values of a and b, 14 + a > 15 + b
∴ More volunteers are now in FR than in ER
Hence, option (b).
Workspace:
After the withdrawal of volunteers, as indicated in Question 89, some new volunteers joined the NGO. Each one of them was allotted only one project in a manner such that, the number of volunteers working in one project alone for each of the three projects became identical. At that point, it was also found that the number of volunteers involved in FR and ER projects was the same as the number of volunteers involved in TR and ER projects. Which of the projects now has the highest number of volunteers?
- (a)
ER
- (b)
FR
- (c)
TR
- (d)
Cannot be determined
Answer: Option A
Text Explanation :
Let m volunteers be added to TR project and n be added to each of FR and ER projects.
Then, 7 + m = 8 + n
∴ m = n + 1
Also, b + 2 = 5
∴ b = 3 and a = 3
Number of volunteers working on TR = 7 + n + 1 + 4 + 5 = 17 + n
Number of volunteers working on FR = 17 + n
Number of volunteers working on ER = 18 + n
Thus, ER has the highest number of volunteers.
Hence, option (a).
Workspace:
70 percent of the employees in a multinational corporation have VCD players, 75 percent have microwave ovens, 80 percent have ACs and 85 percent have washing machines. At least what percentage of employees has all four gadgets?
- (a)
15%
- (b)
5%
- (c)
10%
- (d)
Cannot be determined
Answer: Option C
Text Explanation :
70% have VCD Players.
∴ 30% do not have VCD Players.
75% have microwave ovens.
∴ 25% do not have microwave ovens.
80% have ACs.
∴ 20% do not have ACs.
85% have washing machines.
∴ 15% do not have washing machines.
∴ 30 + 25 + 15 + 20 = 90% of employees do not have at least 1 gadget.
∴ Minimum percentage of employees who has all the four gadgets
= 100 – 90 = 10%
Hence, option (c).
Alternatively,
Minimum percentage of employees which possess both VCDs and Microwaves
= 70% + 75% – 100%
= 45%
Minimum percentage of employees which possess both ACs and Washing machines
= 80% + 85% – 100%
= 65%
∴ Minimum percentage of employees which possess all the four gadgets
= 45% + 65% – 100%
= 10%
Hence, option (c).
Workspace:
On her walk through the park, Hansa collected 50 coloured leaves, all either maple or oak. She sorted them by category when she got home, and found the following:
A. The number of red oak leaves with spots is even and positive.
B. The number of red oak leaves without any spot equals the number of red maple leaves without spots.
C. All non-red oak leaves have spots, and there are five times as many of them as there are red spotted oak leaves.
D. There are no spotted maple leaves that are not red.
E. There are exactly 6 red spotted maple leaves.
F. There are exactly 22 maple leaves that are neither spotted nor red.
How many oak leaves did she collect?
- (a)
22
- (b)
17
- (c)
25
- (d)
18
Answer: Option B
Text Explanation :
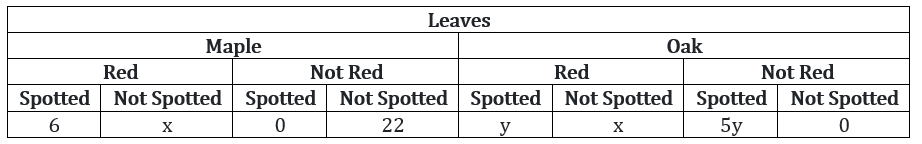
Let the number of red but not spotted oak leaves be ‘x’ and number of red spotted oak leaves be ‘y’.
From the given conditions, we get the following tabulated table.
But, 6 + x + 22 + y + x + 5y = 50
∴ 28 + 2x + 6y = 50
∴ x + 3y = 11
∵ Red spotted oak leaves has to be positive and even.
∴ y = 2
∴ x = 11 − 3 × 2 = 5
∴ Number of Oak leaves = x + y + 5y = 5 + 6 × 2 = 17
Hence, option (b).
Workspace:
Answer the following question based on the information given below.
A and B are two sets (e.g. A = mothers, B = women). The elements that could belong to both the sets (e.g. women who are mothers) is given by the set C = A.B. The elements which could belong to either A or B, or both, is indicated by the set D = A ∪ B. A set that does not contain any elements is known as a null set, represented by ϕ (for example, if none of the women in the set B is a mother, they C = A.B is a null set, or C = ϕ).
Let 'V' signify the set of all vertebrates; 'M' the set of all mammals; 'D' dogs; 'F' fish; 'A' Alsatian and 'P', a dog named Pluto.
Given that X = M.D is such that X = D, which of the following is true?
- (a)
All dogs are mammals.
- (b)
Some dogs are mammals.
- (c)
X = ϕ
- (d)
All mammals are dogs.
Answer: Option A
Text Explanation :
X = M.D implies some dogs are mammals and X = D implies the set of dogs.
This implies all dogs are mammals.
Hence, option (a).
Workspace:
If Y = F. (D.V), is not a null set, it implies that
- (a)
All fish are vertebrates
- (b)
All dogs are vertebrates
- (c)
Some fish are dogs
- (d)
None of the above
Answer: Option C
Text Explanation :
Y = F.(D.V) implies some vertebrates are dogs and some vertebrate dogs are fishes.
∴ Some fishes are dogs.
Hence, option (c).
Workspace:
If Z = (P.D) ∪ M, then
- (a)
The elements of Z consist of Pluto the dog or any other mammal.
- (b)
Z implies any dog or mammal.
- (c)
Z implies Pluto or any dog that is a mammal.
- (d)
Z is a null set.
Answer: Option A
Text Explanation :
Z = (P.D) ∪ M
P.D means a dog called Pluto and P ∪ M means Pluto the dog and the other mammals.
Hence, option (a).
Workspace:
If P.A = ϕ and P ∪ A = D, then which of the following is true?
- (a)
Pluto and Alsatians are dogs.
- (b)
Pluto is an Alsatian.
- (c)
Pluto is not an Alsatian.
- (d)
D is a null set.
Answer: Option C
Text Explanation :
P. A = ϕ means that "Pluto is not an Alsatian".
Hence, option (c).
Workspace:
Feedback
Help us build a Free and Comprehensive Preparation portal for various competitive exams by providing us your valuable feedback about Apti4All and how it can be improved.
 Solution
Solution Discuss
Discuss Report
Report