IIFT 2015 QA | Previous Year IIFT Paper
The internal evaluation for Economics course in an Engineering programme is based on the score of four quizzes. Rahul has secured 70, 90 and 80 in the first three quizzes. The fourth quiz has ten True-False type questions, each carrying 10 marks. What is the probability that Rahul’s average internal marks for the Economics course is more than 80, given that he decides to guess randomly on the final quiz?
- A.
12/1024
- B.
11/1024
- C.
11/256
- D.
12/256
Answer: Option B
Explanation :
Average marks of the first three quizzes = 80
So, for Rahul to have average internal marks more than 80, he has to score more than 80 marks in the last quiz.
This is possible if he attempts 10 questions or 9 questions correctly.
Number of ways this can be done = 1 + 10C9 = 1 + 10 = 11
Total number of ways the quiz can be solved = 210 = 1024
∴ The required probability = 11/1024
Hence, option (b).
Workspace:
In 2004, Rohini was thrice as old as her brother Arvind. In 2014, Rohini was only six years older than her brother. In which year was Rohini born?
- A.
1984
- B.
1986
- C.
1995
- D.
2000
Answer: Option C
Explanation :
Let Rohini’s age in the year 2014 is R and Arvind’s age is A.
∴ R – A = 6 … (i)
∴ Rohini’s age in 2004 = R – 10 and Arvind’s age in 2004 = A – 10
∴ (R – 10) = 3(A – 10)
∴ 3A – R = 20 … (ii)
Solving (i) and (ii), we get
R = 19
∴ Rohini was born in year (2014 – 19 =) 1995.
Hence, option (c).
Alternatively,
Rohini’s age in 2004 for the given options:
1. 1984 : 20
2. 1986 : 22
3. 1995 : 9
4. 2000 : 4
As Rohini’s age was thrice as old as her brother, the correct answer should be option 3.
Hence, option (c).
Workspace:
If p, q and r are three unequal numbers such that p, q and r are in A.P., and p, r-q and q-p are in G.P., then p : q : r is equal to:
- A.
1 : 2 : 3
- B.
2 : 3 : 4
- C.
3 : 2 : 1
- D.
1 : 3 : 4
Answer: Option A
Explanation :
Without loss of generality, p < q < r
∴ r – q = q – p = d
p, r – q and q – p are in G.P.
i.e., p, d, d are in G. P.
So, p = d
∴ q = p + d = 2d and r = 3d
∴ p : q : r = 1 : 2 : 3
Hence, option (d).
Workspace:
If log255 = a and log2515 = b, then the value of log2527 is:
- A.
3(b + a)
- B.
3(1 - b - a)
- C.
3(a + b - 1)
- D.
3(1 - b + a)
Answer: Option C
Explanation :
a + b = log255 + log2515 = log2575 = log2525 + log253
a + b = 1 + log253
a + b – 1 = log253
∴ log2527 = 3log253 = 3(a + b – 1)
Hence, option (c).
Workspace:
During the essay writing stage of MBA admission process in a reputed B-School, each group consists of 10 students. In one such group, two students are batchmates from the same IIT department. Assuming that the students are sitting in a row, the number of ways in which the students can sit so that the two batchmates are not sitting next to each other, is:
- A.
3540340
- B.
2874590
- C.
2903040
- D.
None of the above
Answer: Option C
Explanation :
Number of ways in which 10 students can sit = 10!
The number of ways in which two students (batchmates) sit together = 9! × 2
∴ The number of ways in which the student can sit so that the two batchmates are not sitting next to each other = 10! – 9! × 2 = 9! × 8 = 2903040
Hence, option (c).
Workspace:
The pre-paid recharge of Airtel gives 21% less talktime than the same price pre-paid recharge of Vodafone. The post-paid talktime of Airtel is 12% more than its pre-paid recharge, having the same price. Further, the post-paid talktime of same price of Vodafone is 15% less than its pre-paid recharge. How much percent less / more talktime can one get from the Airtel post-paid service compared to the post-paid service of Vodafone?
- A.
3.9% more
- B.
4.7% less
- C.
4.7% more
- D.
2.8% less
Answer: Option A
Explanation :
Assume that for price P, assume that Vodafone gives talk time of 100 seconds.
So, for the same price P, Airtel gives talk time of 79 (= 21% less than 100) seconds.
The post-paid talk time for the same price by Airtel and Vodafone is 1.12 × 79 and 100 × 0.85, i.e., 88.48 seconds and 85 seconds respectively.
One can get 88.48 – 85 = 3.48 seconds more from Airtel post-paid service compared to the Vodafone post-paid service.
Required percentage = 3.48/85 = 4.07
The closest option is option 1.
Hence, option (a).
Workspace:
As a strategy towards retention of customers, the service centre of a split AC machine manufacturer offers discount as per the following rule: for the second service in a year, the customer can avail of a 10% discount; for the third and fourth servicing within a year, the customer can avail of 11% and 12% discounts respectively of the previous amount paid, Finally, if a customer gets more than four services within a year, he has to pay just 55% of the original servicing charges. If Rohan has availed 5 services from the same service centre in a given year, the total percentage discount availed by him is approximately:
- A.
16.52
- B.
20.88
- C.
22.33
- D.
24.08
Answer: Option B
Explanation :
Let original service charges be Rs. x.
Rohan has paid x, 0.9x, (0.9 × 0.89x =) 0.801x, (0.88 × 0.89 × 0.9x ≈ ) 0.705x, 0.55x for the five services.
Total payment done by Rohan ≈ 3.956x
Discount availed by Rohan ≈ 1.044x
Percentage discount ≈ (1.044 × 100)/5 = 20.88
Hence, option (b).
Workspace:
A tank is connected with both inlet pipes and outlet pipes. Individually, an inlet pipe can fill the tank in 7 hours and an outlet pipe can empty it in 5 hours. If all the pipes are kept open, it takes exactly 7 hours for a completely filled-in tank to empty. If the total number of pipes connected to the tank is 11, how many of these are inlet pipes?
- A.
2
- B.
4
- C.
5
- D.
6
Answer: Option D
Explanation :
Let there be x and y inlet and outlet pipes respectively.
∴ x + y = 11 … (i)
Assume that the capacity of the tank is 35 units.
So, inlet pipe fills 5 units and the outlet pipes empties 7 units of the tank in one hour.
The completely filled tank empties in 7 hours.
∴ 7y – 5x = 5 … (ii)
Solving the two equations, we get y = 5 and x = 6
Hence, option (d).
Workspace:
In a certain village, 22% of the families own agricultural land, 18% own a mobile phone and 1600 families own both agricultural land and a mobile phone. If 68% of the families neither own agricultural land nor a mobile phone, then the total number of families living in the village is:
- A.
20000
- B.
10000
- C.
8000
- D.
5000
Answer: Option A
Explanation :
Let total number of families in the village be T
Number of families own agricultural land, n(A) = 0.22T
Number of families own mobile phone, n(M) = 0.18T
Number of families own both agricultural land and mobile phone, n(A ⋂ M) = 1600
Number of families own agricultural land or mobile phone, n(A ⋃ M) = T – 0.68T = 0.32T
∴ n(A ⋃ M) = n(A) + n(M) – n(A ⋂ M)
∴ n(A ⋂ M) = 0.08T
0.08T = 1600 ⇒ T = 20000
Hence, option (a).
Workspace:
In the board meeting of a FMCG Company, everybody present in the meeting shakes hand with everybody else. If the total number of handshakes is 78, the number of members who attended the board meeting is:
- A.
7
- B.
9
- C.
11
- D.
13
Answer: Option D
Explanation :
Let n members attended the board meeting.
Number of handshakes = n×(n – 1)/2 = 78
Solving this, n = 13
Hence, option (d).
Workspace:
A firm is thinking of buying a printer for its office use for the next one year. The criterion for choosing is based on the least per-page printing cost. It can choose between an inkjet printer which costs Rs. 5000 and a laser printer which costs Rs. 8000. The per-page printing cost for an inkjet is Rs. 1.80 and that for a laser printer is Rs. 1.50. The firm should purchase the laser printer, if the minimum number of a pages to be printed in the year exceeds
- A.
5000
- B.
10000
- C.
15000
- D.
18000
Answer: Option B
Explanation :
Let minimum number of pages to be printed be x.
∴ 5000 + 1.8x = 8000 + 1.5x
Solving, we get x = 10000
Hence, option (b).
Workspace:
If in the figure below, angle XYZ=90° and the length of the arc XZ=10π, then the area of the sector XYZ is:

- A.
10π
- B.
25π
- C.
100π
- D.
None of the above
Answer: Option C
Explanation :
Y is the centre of the circle.
Let r be the radius of the circle.
∴ Length of arc XZ =
∴ r = 20
Arc of sector XYZ =
Hence, option (c).
Workspace:
A chartered bus carrying office employees travels everyday in two shifts- morning and evening. In the evening, the bus travels at an average speed which is 50% greater than the morning average speed; but takes 50% more time than the amount of time it takes in the morning. The average speed of the chartered bus for the entire journey is greater/less than its average speed in the morning by:
- A.
18% less
- B.
30% greater
- C.
37.5% greater
- D.
50% less
Answer: Option B
Explanation :
Let the bus travels at speed ‘V’km/hr for time ‘T’ hours in the morning.
∴ Distance travelled in the morning = VT km
∴ In the evening, it travels at speed ‘1.5V’km/hr for ‘1.5T’ hours respectively.
∴ Distance travelled in the evening = 1.5V × 1.5T = 2.25VT
∴ Average speed =
∴ The average speed of the chartered bus for the entire journey is greater than its average speed in the morning by 30%.
Hence, option (b).
Workspace:
If a right circular cylinder of height 14 is inscribed in a sphere of radius 8, then the volume of the cylinder is:
- A.
110
- B.
220
- C.
440
- D.
660
Answer: Option D
Explanation :
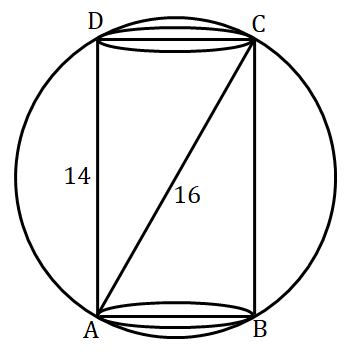
DC is diameter of the cylinder.
m∠ADC = 90°
∴ AC is the diameter of the sphere.
Thus, AC = 16 and AD = height of the cylinder = 14
DC2 = 162 – 142 = 60
∴ Radius2 = 60/4 = 15
∴ Volume of cylinder = πr2h = π × 15 × 14 = 660
Hence, option (d).
Workspace:
Seema has joined a new Company after the completion of her B.Tech from a reputed engineering college in Chennai. She saves 10% of her income in each of the first three months of her service and for every subsequent month, her savings are Rs. 50 more than the savings of the immediate previous month. If her joining income was Rs. 3000, her total savings from the start of the service will be Rs.11400 in:
- A.
6 months
- B.
12 months
- C.
18 months
- D.
24 months
Answer: Option C
Explanation :
Seema saves Rs. 900 in first three months.
Let she reach the given amount in X more months.
She would save 300X + 50 + 50 × 2 + 50 × 3 +......... + 50 × X = 11400 − 900
∴ 300X + 50(1 + 2 + 3 +......+ X) = 10500
∴ X2 + 13X - 420 = 0
On solving, we get X = 15.
Thus, in 15 + 3 = 18 months her savings will be Rs. 11,400.
Hence, option (c).
Workspace:
Sailesh is working as a sales executive with a reputed FMCG Company in Hyderabad. As per the Company’s policy, Sailesh gets a commission of 6% on all sales upto Rs.1,00,000 and 5% on all sales in excess of this amount. If Sailesh remits Rs. 2,65,000 to the FMCG company after deducting his commission, his total slaes were worth:
- A.
Rs. 1,20,000
- B.
Rs. 2,90,526
- C.
Rs. 2,21,054
- D.
Rs. 2,80,000
Answer: Option D
Explanation :
Sailesh earns Rs. 6,000 as commission from first Rs. 1,00,000.
Let his total sales = X
∴ Total commission = 6000 + 0.05 (X – 100000) = 0.05X + 1000
∴ X – 0.05X + 1000 = 265000
Solving, we get X = 280000
Hence, option (d).
Workspace:
Three carpenters P, Q and R are entrusted with office furniture work. P can do a job in 42 days. If Q is 26% more efficient than P and R is 50% more efficient than Q, then Q and R together can finish the job in approximately:
- A.
11 days
- B.
13 days
- C.
15 days
- D.
17 days
Answer: Option B
Explanation :
Assume that P does p units of work in one day.
∴ Total work = 42p
So, Q and R do 1.26p and (1.26 × 1.5 =)1.89p units of work in one day respectively.
∴ Total work done by Q and R in one day = 1.26p + 1.89p = 3.15p
So, Q and R can finish the work in (42p/3.15p ≈) 13 days.
Hence, option (b).
Workspace:
There are two alloys P and Q made up of silver, copper and aluminium. Alloy P contains 45% silver and rest aluminum. Alloy Q contains 30% silver, 35% copper and rest aluminium. Alloys P and Q are mixed in the ratio of 1:4.5. The approximate percentages of silver and copper in the newly formed alloy is:
- A.
33% and 29%
- B.
29% and 26%
- C.
35% and 30%
- D.
None of the above
Answer: Option A
Explanation :
P and Q are mixed in the ratio 1 : 4.5 i.e., 2 : 9.
Assume that 1 kg of P is mixed with 4.5 kg of Q.
1 kg of P has 0.45 kg of silver.
4.5 kg of Q has (0.3 × 4.5 =) 1.35 kg of silver and (0.35 × 4.5 =) 1.575 kg of copper.
Thus, the newly formed alloy of 5.5 kg has 1.8 kg of silver and 1.575 kg of copper.
∴ % of silver ≈ 33 and % of copper = 29
Hence, option (a).
Workspace:
A ladder of 7.6 m long is standing against a wall and the difference between the wall and the base of the ladder is 6.4 m. If the top of the aldder now slips by 1.2m, then the foot of the ladder shifts by approximately:
- A.
0.4 m
- B.
0.6 m
- C.
0.8 m
- D.
1.2 m
Answer: Option B
Explanation :
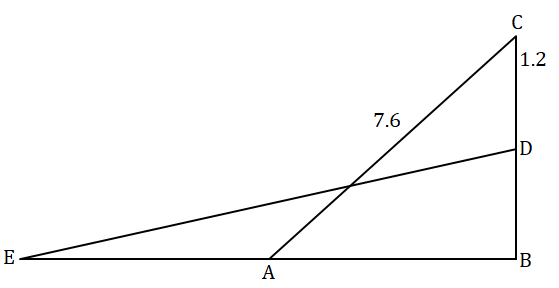
AC = ED = 7.6 and AB = 6.4
From the given diagram ; BC2 = 7.62 – 6.42 = 16.8
∴ BC ≈ 4.1 m
When the ladder slips 1.2 m, its top edge would be at D at a height of 4.1 – 1.2 ≈ 2.9 m
∴ BE2 = 7.62 – 2.92 ≈ 49
∴ BE ≈ 7
∴ Ladder shifts approximately 7 – 6.4 = 0.6 m.
Hence, option (b).
Workspace:
The value of x for which the equation will be satisfied is:
- A.
1
- B.
2
- C.
3
- D.
4
Answer: Option D
Explanation :
Substituting values given in options, the equation is satisfied for x = 4.
Hence, option (d).
Workspace:
The simplest value of the expression is:
- A.
4
- B.
8
- C.
4p
- D.
8p
Answer: Option B
Explanation :
Hence, option (b).
Workspace:
In a reputed engineering college in Delhi, students are evaluated based on trimesters. The probability that an Engineering student fails in the first trimester is 0.08. If he does not fail in the first trimester, the probability that he is promoted to the second year is 0.87. The probability that the student will complete the first year in the Engineering College is approximately:
- A.
0.8
- B.
0.6
- C.
0.4
- D.
0.7
Answer: Option A
Explanation :
We consider that the student fails in the first year if he fails in the first trimester.
Therefore, the probability that the student will complete the first year the first year in the Engineering College is approximately = Probability that he passes 1st trimester × Probability that he passes 1st semester and is promoted to the second year) = 0.92 × 0.87 ≈ 0.8
Hence, option (a).
Workspace:
Feedback
Help us build a Free and Comprehensive Preparation portal for various competitive exams by providing us your valuable feedback about Apti4All and how it can be improved.
 Solution
Solution Discuss
Discuss Report
Report