CRE 2 - Relative Speed | Time, Speed & Distance

1. CRE 2 - Relative Speed | Time, Speed & Distance

Two cyclists start from the same place in the opposite directions. One goes towards north at 36 km/h. and the other goes towards south at the speed of 40 km/h. What time will they take to be 190 km. apart?

(a)
$4 \frac{1}{2} h r s$
(b)
4 hrs. 45 min
(c)
5 hrs. 16 min
(d)
2 hrs. 30 min.

Answer: Option D

Explanation :

Since they are going in opposite directions, their relative speed = 36 + 40 = 76 kmph.

They are 76 km apart in 1 hour. They will be 190 km apart in = $(\frac{1}{76} \times 190)$ = 2 hrs. 30 mins.

Hence, option (d).

2. CRE 2 - Relative Speed | Time, Speed & Distance

X and Y are two stations 250 km apart. A train starts from X and moves towards Y at the rate of 10 km/h. Another train starts from Y at the same time and moves towards X at the rate of 15 km/h. How far from X will they cross each other?

(a)
200 km
(b)
300 km
(c)
120 km
(d)
40 km

Answer: Option A

Explanation :

Suppose they meet at a distance of x km. from x. Then,

$\frac{x}{10} = \frac{500 - x}{15}$

⇒x=200 kms.

Hence, option (a)

3. CRE 2 - Relative Speed | Time, Speed & Distance

A thief steals a car at 2:30 p.m. and drives it at 30 km/h. The theft is discovered at 3 p.m. and the owner sets off in another car at 50 km/h. when will he overtake the thief

(a)
3:30 p.m.
(b)
3:45 p.m.
(c)
4 p.m.
(d)
4:15 p.m.

Answer: Option B

Explanation :

Here, distance to be covered by the thief and by the owner is same.

Let after 2:30 p.m., owner catches the thief in t hrs.

Then, 30×t=50 $(t - \frac{1}{2})$

⇒t = $\frac{5}{4}$ hrs

So, the thief is overtaken at 3:45 p.m.

Hence, option (b).

4. CRE 2 - Relative Speed | Time, Speed & Distance

Points A and B are 35 km apart on a highway. One car starts from A and the another one from B at the same time. If they travel in the same direction, they meet in 7 hours. But if they travel towards each other, they meet in one hour. The speed of the two cars are, respectively.

(a)
25 and 20 km/h
(b)
35 and 5 km/h
(c)
20 and 15 km/h
(d)
30 and 20 km/h

Answer: Option C

Explanation :

Let the speed of the car be x km/h and y km/h, respectively.

Their relative speeds when they are moving in same direction = (x – y) km/h.

Their relative speeds when they are in opposite directions = (x + y) km/h.

Now, $\frac{35}{x + y} = 1$ or x + y = 35 …(1)

and $\frac{35}{x - y} = 7$ or x – y = 5 …(2)

Solving (1) and (2), we have

x = 20 km/h and y = 15 km/h

Hence, option (c).

5. CRE 2 - Relative Speed | Time, Speed & Distance

distance between two cities A and B is 330 km. A train starts from A at 8 a.m. and travels towards B at 60 km/h. Another train starts from B at 9 a.m. and travels towards A at 75 km/h. At what time do they meet?

(a)
10 a.m.
(b)
10:30 a.m.
(c)
11 a.m.
(d)
11:30 a.m.

6. CRE 2 - Relative Speed | Time, Speed & Distance

A Person X started at 3 hours earlier at 40km/h from a place P, then another person Y followed him at 60km/h started his journey at 3 O'clock, afternoon. What is the difference in time when X was 30 km ahead of Y and when Y was 30 km ahead of X?

(a)
2 h
(b)
3 h
(c)
3.5 h
(d)
4.25 h

7. CRE 2 - Relative Speed | Time, Speed & Distance

A thief is noticed by a policeman from a distance of 200m. The thief starts running and the policeman chases him. The thief and the policeman run at the rate of 10 km and 11 km per hour respectively. What is the distance between them after 6 minutes?

(a)
100 m
(b)
120 m
(c)
110 m
(d)
130 m

8. CRE 2 - Relative Speed | Time, Speed & Distance

A car driver driving in fog, passes a pedestrian who was walking at the rate of 2km/h in the same direction. The pedestrian could see the car for 6 mins and it was visible to him up to a distance of 0.5 km. The speed of the car would be:

(a)
7 km/h
(b)
8 km/h
(c)
6 km/h
(d)
15 km/h

9. CRE 2 - Relative Speed | Time, Speed & Distance

2 trains start from the opposite station and at the same time a bird with the constant speed of 25 kmph starts from one train and go to another train and then return to the first train again and vice versa till both the train meet each other in 1 hour. Find out the total distance travelled by the bird.

(a)
24 km
(b)
50 km
(c)
25 km
(d)
Can’t be determined

10. CRE 2 - Relative Speed | Time, Speed & Distance

Two guns were fired from the same place at an interval of 10 minutes and 30 seconds, but a person in the train approaching the place hears the second shot 10 minutes after the first. The speed of the train (in km/hr), supposing that speed travels at 330 metres per second, is:

(a)
50.4
(b)
11.8
(c)
19.88
(d)
59.4

A and B are running between two cities P and Q. A starts from P towards Q, reaches Q and then turns back. Similarly,B starts from Q towards P, reaches P and then turns back. They keep running to and fro in this manner.P and Q are 100 kms apart and A and B are running with respective speeds of 10 and 15 kmph.

11. CRE 2 - Relative Speed | Time, Speed & Distance

If both A and B start simultaneously from P and Q respectively, how long will they take to meet for the first time (hours)?

Backspace

7 8 9 4 5 6 1 2 3 0 . -

Clear All Submit

Answer: 4

Explanation :

Distance between A and B initially = 100 kms.

Relative speed = 10 + 15 = 25 kmph.

∴ Time taken for them to meet for the first time = $\frac{100}{25}$ = 4 hours.

Hence, 4.

12. CRE 2 - Relative Speed | Time, Speed & Distance

If both A and B start simultaneously from P and Q respectively, how long will they take to meet for the second time (hours)?

(a)
10
(b)
12
(c)
8
(d)
None of these

Answer: Option B

Explanation :

Since the ratio of speeds of faster person to slower person is less than 2, both of them will reach their respective ends and turn back before meeting for the second time.

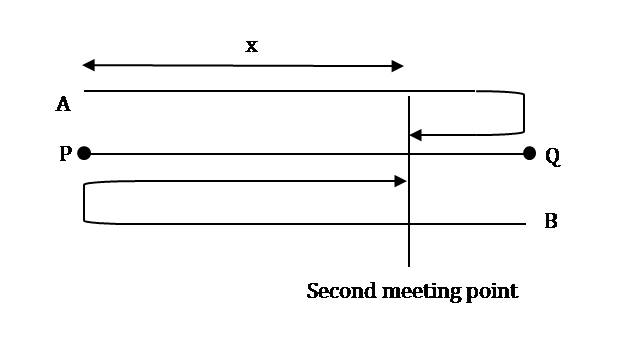
Suppose they meet at a distance of x kms from P.

⇒ Distance covered by A = 100 + (100 - x) = 200 – x kms.

⇒ Distance covered by B = 100 + x kms.

Since both of them cover these distance in same time, ratio of speeds will be same as ratio of distances covered.

$\frac{200 - x}{100 + x} = \frac{10}{15}$

⇒ x = 80 kms.

⇒ A covers a total distance of 200 – 80 = 120 kms at the speed of 10 kmph.

∴ Time taken by A = 120/10 = 12 hours.

Hence, they meet for the second time after 12 hours.

Hence, option (b).

13. CRE 2 - Relative Speed | Time, Speed & Distance

If both A and B start simultaneously from P and Q respectively, how long will they take to meet for the third time (hours)?

Backspace

7 8 9 4 5 6 1 2 3 0 . -

Clear All Submit

Answer: 20

Explanation :

Since the ratio of speeds of faster person to slower person is less than 2,

Time taken to meet nth time = $\frac{(2 n - 1) D}{R_{s}}$

where,
D = distance between the end points.
Rs = Relative speed of the people running.

Here, time taken to meet for the third time = $\frac{(2 \times 3 - 1) \times 100}{10 + 15} = 20$ hours.

Hence, 20.

Direction:

A and B are running between two cities P and Q. A starts from P towards Q, reaches Q and then turns back. Similarly, B starts from Q towards P, reaches P and then turns back. They keep running to and fro in this manner.P and Q are 100 kms apart and A and B are running with respective speeds of 5 and 20 kmph.

14. CRE 2 - Relative Speed | Time, Speed & Distance

If both A and B start simultaneously from P and Q respectively, how long will they take to meet for the first time (hours)?

Backspace

7 8 9 4 5 6 1 2 3 0 . -

Clear All Submit

CRE 2 - Relative Speed | Time, Speed & Distance

Subscribe to our Newsletter