IPMAT (I) 2020 QA MCQ | Previous Year IPMAT - Indore Paper
The probability that a randomly chosen factor of 1019 is a multiple of 1015 is
- A.
1/25
- B.
1/12
- C.
1/20
- D.
1/16
Answer: Option D
Explanation :
Total number of factors of 1019 = 219 × 519 are (19 + 1) × (19 + 1) = 400
Factors of 219 × 519 which are also a multiple of 1015 will have power of both 2 and 5 greater than or equal to 15.
Possible powers of 2 or 5 are 15 or 16 or 17 or 18 or 19 i.e., 5 possibilities.
∴ Number of such factors are 5 × 5 = 25
⇒ Required probability = 25/400 = 1/16
Hence, option (d).
Workspace:
The number of acute angled triangles whose sides are three consecutive positive integers and whose perimeter is at most 100 is
- A.
28
- B.
29
- C.
31
- D.
33
Answer: Option B
Explanation :
Let the sides of the triangle be x, x + 1 and x + 2.
Now, x + x + 1 + x + 2 ≤ 100
⇒ 3x ≤ 97
⇒ x ≤ 32.33 ...(1)
Also, the given triangle is an acute triangle, hence
(x + 2)2 < (x + 1)2 + x2
⇒ x2 + 4 + 4x < x2 + 2x + 1 + x2
⇒ x2 - 2x - 3 > 0
⇒ (x - 3)(x + 1) > 0
⇒ x < -1 or x > 3 ...(2)
From (1) and (2), we get
x can take any value out of 4, 5, 6, ..., 31 and 32 i.e., 29 values.
Hence, option (b).
Workspace:
The equation of the straight line passing through the point M (-5, 4), such that the portion of it between the axes is divided by the point M in to two equal halves, is
- A.
10y - 8x = 80
- B.
8y + 10x = 80
- C.
10y + 8x = 80
- D.
8y + 10x + 80 = 0
Answer: Option A
Explanation :
Let the x-intercept and y-intercept of the line are B (a, 0) and A (0, b)
 2020 point M.png)
Since M (-5, 4) is the midpoint of these two intercepts, we have
-5 = (a + 0)/2 and 4 = (0 + b)/2
⇒ a = -10 and b = 8
∴ Equation of the line is: x/-10 + y/8 = 1
⇒ -8x + 10y = 80
⇒ 10y - 8x = 80
Hence, option (a).
Workspace:
The value of + + +
- A.
1
- B.
3/2
- C.
2
- D.
9/4
Answer: Option C
Explanation :
Given, + + +
= + + +
= + + +
= + + + +
= 1 + 1 = 2
Hence, option (c).
Workspace:
If + + + ... upto ∞ = , then the value of + + + ... upto ∞ is
- A.
- B.
- C.
- D.
Answer: Option A
Explanation :
If + + + ... upto ∞ = , then the value of
Let X = + + + ... upto ∞
Adding and subtracting + + + ... upto ∞, we have
X = + + + ... upto ∞ - ( + + + ... upto ∞)
⇒ X = - ( + + + ... upto ∞)
⇒ X = - ()
⇒ X = - ()
⇒ X =
Hence, option (a).
Workspace:
A man is known to speak the truth on an average 4 out of 5 times. He throws a die and reports that it is a five. The probability that it is actually a five is
- A.
4/9
- B.
5/9
- C.
4/15
- D.
2/15
Answer: Option E
Explanation :
The answer should be 4/5.
P(A given B) = P(A and B) ÷ P(B)
P(5 given that he says it is a 5) = P(5 and he says it is 5) ÷ P(he says it is 5)
P(5 and he says it is 5) = P(it is 5) × P(he say truth) = 1/6 × 4/5 = 4/30
P(he says it is 5) = P(it is 5) × P(he says the truth) + P(it is not 5) × P(he lies) × P(he lies that it is 5) = 1/6 × 4/5 + 5/6 × 1/5 × 1/5 = 4/30 + 1/30 = 5/30
∴ P(5 given that he says it is a 5) = 4/30 ÷ 5/30 = 4/5.
Hence, 4/5.
Note:
Let's say the die rolls a 4 and the person lies, the person could lie that it is a 1 or 2 or 3 or 5 or 6.
Now, probability that the person lies that is it 5 = 1/5
∴ P(it is not 5) × P(he lies) × P(he lies that it is 5) = 5/6 × 1/5 × 1/5 = 1/30.
In most study material you would find the answer to this question as 4/9.
This is because they assume that when the die does not roll a 5 and he lies, he will always say that it is 5. But this is not true. He could lie that it is a 4 when a 6 rolls. Hence, the difference in the answer.
Workspace:
If log5log8(x2 - 1) = 0, then a possible value of x is
- A.
2√2
- B.
√2
- C.
2
- D.
3
Answer: Option D
Explanation :
Given, log5log8(x2 - 1) = 0
⇒ log8(x2 - 1) = 50 = 1
⇒ (x2 - 1) = 81
⇒ x2 = 9
⇒ x = +3 or -3.
Hence, option (d).
Workspace:
Consider the following statements:
(i) When 0 < x < 1, then < 1 - x + x2
(ii) When 0 < x < 1, then > 1 - x + x2
(iii) When -1 < x < 0, then < 1 - x + x2
(iv) When -1 < x < 0, then > 1 - x + x2
Then the correct statements are
- A.
(i) and (ii)
- B.
(ii) and (iv)
- C.
(i) and (iv)
- D.
(ii) and (iii)
Answer: Option C
Explanation :
When 0 < x < 1, consider and 1 - x + x2
Let us take x = 0.5
∴ = 0.67 and 1 - x + x2 = 1 - 0.5 + 0.25 = 0.75
⇒ < 1 - x + x2
∴ (i) is correct.
When 0 < x < 1, consider and 1 - x + x2
Let us take x = -0.5
∴ = 2 and 1 - x + x2 = 1 + 0.5 + 0.25 = 1.75
⇒ > 1 - x + x2
∴ (iv) is correct.
Hence, option (d).
Workspace:
Fifty litres of a mixture of milk and water contains 30 percent of water. This mixture is added to eighty litres of another mixture of milk and water that contains 20 percent of water. Then, how many litres of water should be added to the resulting mixture to obtain a final mixture that contains 25 percent of water?
- A.
1
- B.
2
- C.
3
- D.
4
Answer: Option B
Explanation :
Fifty litres of a mixture of milk and water contains 30 percent of water.
⇒ Quantity of milk = 70% of 50 = 35 liters
⇒ Quantity of water = 30% of 50 = 15 liters
Eighty litres of another mixture of milk and water that contains 20 percent of water
⇒ Quantity of milk = 80% of 80 = 64 liters
⇒ Quantity of water = 20% of 80 = 16 liters
Let x liters of water is added along with these two solutions to make water 25% of the whole mixture.
⇒ 15 + 16 + x = 25% of (50 + 80 + x)
⇒ 31 + x = 25% of (130 + x)
⇒ 31 + x = 32.5 + 0.25x
⇒ 0.75x = 1.5
⇒ x = 2 liters.
Hence, option (b).
Workspace:
Three workers working together need 1 hour to construct a wall. The first worker, working alone, can construct the wall twice as fast at the third worker, and can complete the task an hour sooner than the second worker. Then, the average time in hours taken by the three workers, when working alone, to construct the wall is
- A.
(√33 + 4)/3
- B.
(√33 + 3)/3
- C.
(√33 + 6)/5
- D.
(√33 + 7)/3
Answer: Option A
Explanation :
Let the time taken by the first worker be x hours.
∴ Time taken by the second worker = x + 1 hours.
∴ Time taken by the third worker = 2x hours.
Together they take 1 hour to complete the task.
⇒ =
⇒ =
⇒ 3(x + 1) + 2x = 2x × (x + 1)
⇒ 5x + 3 = 2x2 + 2x
⇒ 2x2 - 3x - 3 = 0
⇒ x = =
We will accept only +ve value.
∴ x =
⇒ Required average = = =
Hence, option (a).
Workspace:
In a class, students are assigned roll numbers from 1 to 140. All students with even roll numbers opted for cricket, all those whose roll numbers are divisible by 5 opted for football, and all those whose roll numbers are divisible by 3 opted for basketball. 'The number of students who did not opt for any of the three sports is
- A.
102
- B.
38
- C.
98
- D.
42
Answer: Option B
Explanation :
Number of students who opted for cricket = Number of number which are divisible by 2 = Quotient of 140/2 = 70.
Number of students who opted for football = Number of number which are divisible by 5 = Quotient of 140/5 = 28.
Number of students who opted for basketball = Number of number which are divisible by 3 = Quotient of 140/3 = 46.
Number of students who opted for cricket and football = Number of number which are divisible by 2 and 5 = Quotient of 140/10 = 14.
Number of students who opted for basketball and football = Number of number which are divisible by 3 and 5 = Quotient of 140/15 = 9.
Number of students who opted for cricket and basketball = Number of number which are divisible by 2 and 3 = Quotient of 140/6 = 23.
Number of students who opted for cricket and football and basketball = Number of number which are divisible by 2, 5 and 3 = Quotient of 140/30 = 4.
∴ C ∪ F ∪ B = C + F + B - C ∩ F - F ∩ B - B ∩ C + C ∩ F ∩ B
⇒ C ∪ F ∪ B = 70 + 28 + 46 - (14 + 9 + 23) + 4
⇒ C ∪ F ∪ B = 144 - 46 + 4 = 102
∴ Number of students who opted for none of the three sports = 140 - 102 = 38.
Hence, option (b).
Workspace:
Given f(x) = x2 + log3x and g(y) = 2y + f(y), then the value of g(3) equals
- A.
16
- B.
15
- C.
25
- D.
26
Answer: Option A
Explanation :
Given, f(x) = x2 + log3x and g(y) = 2y + f(y),
∴ g(3) = 2 × 3 + f(3)
⇒ g(3) = 2 × 3 + 32 + log33
⇒ g(3) = 6 + 9 + 1 = 16
Hence, option (a).
Workspace:
A 2 × 2 matrix is filled with four distinct integers randomly chosen from the set {1,2,3,4,5,6}. Then the probability that the matrix generated in such a way is singular is
- A.
2/45
- B.
1/45
- C.
4/15
- D.
1/15
Answer: Option A
Explanation :
Total number of matrices formed = Number of ways of selecting 4 distinct integer × Arranging these 4 integers in the matrix.
= 6C4 × 4! = 15 × 24 = 360
For a singular matrix , its determinant = 0.
∴ ad - bc = 0
⇒ ad = bc
Now, possible pairs of a and d can be
Case 1: a and b are 2 or 3,
∴ a × d = b × c = 6
⇒ b and c are 1 or 6.
∴ Possible arrangements for a and d = 2! and that for b and c = 2!
∴ Total possible arrangements for a, b, c and d = 2! × 2! = 4.
Case 2: a and b are 1 or 6,
∴ a × d = b × c = 6
⇒ b and c are 2 or 3.
∴ Possible arrangements for a and d = 2! and that for b and c = 2!
∴ Total possible arrangements for a, b, c and d = 2! × 2! = 4.
Case 3: a and b are 3 or 4,
∴ a × d = b × c = 12
⇒ b and c are 2 or 6.
∴ Possible arrangements for a and d = 2! and that for b and c = 2!
∴ Total possible arrangements for a, b, c and d = 2! × 2! = 4.
Case 4: a and b are 2 or 6,
∴ a × d = b × c = 12
⇒ b and c are 3 or 4.
∴ Possible arrangements for a and d = 2! and that for b and c = 2!
∴ Total possible arrangements for a, b, c and d = 2! × 2! = 4.
⇒ Total number of required matrices = 4 + 4 + 4 + 4 = 16
Required probability = 16/360 = 2/45.
Hence, option (a).
Workspace:
Ashok started a business with a certain investment. After few months, Bharat joined him investing half amount of Ashok's initial investment. At the end of the first year, the total profit was divided between them in ratio 3:1 . Bharat joined Ashok after
- A.
2 months
- B.
3 months
- C.
4 months
- D.
6 months
Answer: Option C
Explanation :
Let Ashok's investment be Rs. 2x, hence Bharat's investment is Rs. x.
Ashok invested for 12 months whereas Bharat invested for say m months.
⇒ =
⇒ =
⇒ 3m = 24
⇒ m = 8
∴ If Bharat invested for 8 months it means he invested after 12 - 8 = 4 months of Ashok.
Hence, option (c).
Workspace:
The average marks of 6 students in a test is 64 . All the students got different marks, one of the students obtained 70 marks and all other students scored 40 or above. The maximum possible difference between the second highest and the second lowest marks is
- A.
50
- B.
54
- C.
57
- D.
58
Answer: Option B
Explanation :
Total marks of 6 students = 6 × 64 = 384
Marks of all the students are distinct and greater than 40.
We need to maximise the difference of 2nd highest and 2nd lowest marks
For this we need to minimise the 2nd lowest marks and maximise the 2nd highest marks.
Now, least possible marks of bottom 2 students = 40 and 41.
Marks of the students is 70.
Let the marks of remaining three students be A, B and C
∴ 40 + 41 + 70 + A + B + C = 384
⇒ A + B + C = 233.
Now, if C is higest and B is second highest, we can maximise B by minimising A.
Least possible marks of A now is 42.
⇒ B + C = 191
For B to be maximum possible, B and C should be as close as possible.
∴ C = B + 1
⇒ B + B + 1 = 191
⇒ B = 95
∴ The 6 numbers are: 40, 41, 42, 70, 95, 96
⇒ Differnce between 2nd highest and 2nd lowest marks = 95 - 41 = 54.
Hence, option (b).
Workspace:
Answer the following questions based on the information given below:
The table below represents the buy and sell prices of five stocks during the five trading days of a given week. The quoted sell price is the price at which an investor can sell a stock in the market. The quoted buy price is the price at which an investor can buy a stock from the market. All the quoted numbers are in Indian Rupees.
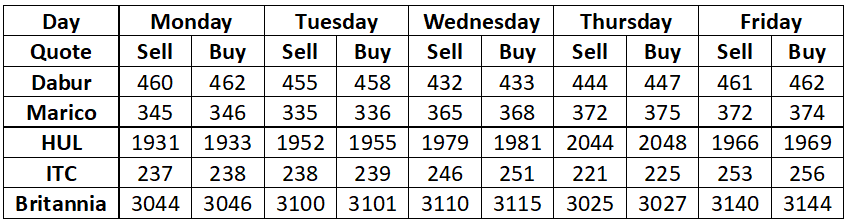
If an investor had Rs 36,00,000 to invest in any particular single stock, and she could buy the stock only on Monday and sell it off only on Fridav. then the stock she should buy on Monday to earn the maximum possible profit during the week is
- A.
Marico
- B.
HUL
- C.
ITC
- D.
Britannia
Answer: Option A
Explanation :
To earn maximum profit, the investor should invest in the stock which gives the maximum profit % over the 5 days.
Profit % of
Option (a): Marico = (372/346 - 1) × 100% = 7.5%
Option (b): HUL = (1966/1933 - 1) × 100% = 1.7%
Option (c): ITC = (25/238 - 1) × 100% = 6.3%
Option (d): Brittania = (3140/3046 - 1) × 100% = 3.1%
By investing in Marico, the investor will earn maximum profit.
Hence, option (a).
Workspace:
If an investor planned to invest Rs 36,00,000 in purchasing the stocks of HUL on Monday, sell them off on Wednesday and use the entire proceeds to purchase the stocks of Britannia on the same day and sell them off again on Friday, then the total investment return during the week would be
- A.
2.8%
- B.
3%
- C.
3.2%
- D.
3.4%
Answer: Option C
Explanation :
Multiplication for the whole process = 1979/1933 × 3140/3115 = 1.032
∴ Overall % change = (1.032 - 1) × 100% = 3.2%
Hence, option (c).
Workspace:
The difference between the quoted buy and sell price of a stock is referred to as the spread of the stock. The average spread of the stocks is lowest on
- A.
Monday
- B.
Tuesday
- C.
Thursday
- D.
Friday
Answer: Option A
Explanation :
Average spread on
Monday = (2 + 1 + 2 + 1 + 2)/5 = 1.6
Tuesday = (3 + 1 + 3 + 1 + 1)/5 = 1.8
Wednesday = (1 + 3 + 2 + 5 + 5)/5 = 3.2
Thursday = (3 + 3 + 4 + 4 + 2)/5 = 3.2
Friday = (1 + 2 + 3 + 3 + 4)/5 = 2.6
Hence, option (a).
Workspace:
A brokerage firm charges 0.1 percent trading commission on the value of shares bought or sold through its trading platform. If an investor bought 1000 shares of Britannia on Tuesday, and sold all of them on Thursday, then the total brokerage fee that will be charged from the investor is
- A.
6,125
- B.
6,126
- C.
6,127
- D.
6,128
Answer: Option B
Explanation :
Total transation value = 1000 × 3101 + 1000 × 3025 = 1000 × 6126 = 6126000
∴ Commission = 0.1% of 6126000 = 0.1/100 × 6126000 = 6126.
Hence, option (b).
Workspace:
If you had decided to invest Rs.36,00,000 worth of ITC stocks on Monday, then the day of the week you should choose to sell the stocks to earn the maximum possible profit would be
- A.
Tuesday
- B.
Wednesday
- C.
Thursday
- D.
Friday
Answer: Option D
Explanation :
To earn maximum possible profit we should sell the stocks when the sellling price is highest.
Of all the 5 days, selling price is highest on Friday i.e., 253.
Hence, option (d).
Workspace:
 Solution
Solution Discuss
Discuss Report
Report