IPMAT (I) 2021 QA MCQ | Previous Year IPMAT - Indore Paper
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Suppose that a real-valued function f(x) of real numbers satisfies f(x + xy) = f(x) + f(xy) for all real x, y, and that f(2020) = 1. Compute f(2021).
- A.
2021/2020
- B.
2020/2019
- C.
1
- D.
2020/2021
Answer: Option A
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
f(x + xy) = f(x) + f(xy)
Substituting y = 1
⇒ f(x + x) = f(x) + f(x)
⇒ f(2x) = 2f(x)
Substituting y = 2
⇒ f(x + 2x) = f(x) + f(2x)
⇒ f(3x) = f(x) + 2f(x)
⇒ f(3x) = 3f(x)
Hence, we can say, f(ax) = a × f(x) ...(1)
Substituting a = 2020 and x = 1
⇒ f(2020) = 2020 × f(1)
⇒ 1 = 2020 × f(1)
⇒ f(1) = 1/2020
Now, putting x = 1 and a = 2021 in (1) we get,
f(2021) = 2021 × f(1) = 2021/2020
Hence, option (a).
Workspace:
Suppose that log2[log3(log4a)] = log3[log4(log2b)] = log4[log2(log3c)] = 0 then the value of a + b + c is
- A.
105
- B.
71
- C.
89
- D.
37
Answer: Option C
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
Given, log2[log3(log4a)] = log3[log4(log2b)] = log4[log2(log3c)] = 0
⇒ log2[log3(log4a)] = 0
⇒ log3(log4a) = 20 = 1
⇒ log4a = 31 = 3
⇒ a = 43 = 64
Also, log3[log4(log2b)] = 0
⇒ log4(log2b) = 30 = 1
⇒ log2b = 41 = 4
⇒ b = 24 = 16
Also, log4[log2(log3c)] = 0
⇒ log2(log3c) = 40 = 1
⇒ log3c = 21 = 2
⇒ c = 32 = 9
∴ a + b + c = 64 + 16 + 9 = 89.
Hence, option (c).
Workspace:
Let Sn be sum of the first n terms of an A.P. {an}. If S5 = S9 , what is the ratio of a3 : a5
- A.
9 : 5
- B.
5 : 9
- C.
3 : 5
- D.
5 : 3
Answer: Option A
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
In an AP S of odd number of terms = n × (Middle term)
Given, S5 = S9
⇒ 5 × a3 = 9 × a9
⇒ a3 : a9 = 9 : 5
Hence, option (a).
Workspace:
If A, B and A + B are non singular matrices and AB = BA then 2A - B - A(A + B)-1A + B(A + B)-1B equals
- A.
A
- B.
B
- C.
A + B
- D.
I
Answer: Option A
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
Given, 2A - B - A(A + B)-1A + B(A + B)-1B
= 2A - B - (A + B)-1[A2 - B2]
= 2A - B - (A + B)-1(A - B)(A + B)
= 2A - B - (A + B)-1(A + B)(A - B)
= 2A - B - I(A - B)
= 2A - B - (A - B)
= 2A - B - A + B
= A
Hence, option (a).
Workspace:
If the angles A, B, C of a triangle are in arithmetic progression such that sin(2A + B) = 1/2 then sin(B + 2C) is equal to
- A.
-1/2
- B.
1/2
- C.
-1/√2
- D.
3/√2
Answer: Option A
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
Let the three angle of the triangle be A = a - d, B = a and C = a + d degrees.
⇒ A + B + C = (a - d) + a + (a + d) = 180°
⇒ a = 60°
∴ B = 60°
Now, Sin(2A + B) = 1/2
Since, B = 60° (2A + B) > 60°
Sin30° = 1/2 or Sin(180 - 30) = 1/2
⇒ 2A + B = 180 - 30
⇒ 2A + 60° = 150°
⇒ A = 45°
∴ C = 180 - 45 - 60 = 75°
∴ Sin(B + 2C) = Sin(210°)
= Sin(180° + 30°)
= - Sin(30°)
= - 1/2
Hence, option (a).
Workspace:
The unit digit in (743)85 – (525)37 + (987)96 is ________
- A.
9
- B.
3
- C.
1
- D.
5
Answer: Option A
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
Unit's digit of (743)85 = Unit's digit of 385
= Unit's digit of (34)21 × 3 = 1 × 3 = 3 [Unit's digit of 34 = 1]
Units's digit of (525)37 = Units's digit of 537 = 5
Units's digit of (987)96 = Units's digit of 796
= Units's digit of (74)24 = 1 [Unit's digit of 74 = 1]
∴ Unit's digit of (743)85 – (525)37 + (987)96 = 3 - 5 + 1 = -1 = 9
Hence, option (a).
Workspace:
The set of all real value of p for which the equation 3sin2x + 12cosx – 3 = p has at least one solution is
- A.
[-12, 12]
- B.
[-12, 9]
- C.
[-15, 9]
- D.
[-15, 12]
Answer: Option C
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
The set of all real value of p for which the equation 3sin2x + 12cosx – 3 = p has one solution is
Given, 3sin2x + 12cosx – 3 = p
⇒ 3(1 - cos2x) + 12cosx – 3 = p
⇒ -3cos2x + 12cosx – p = 0
⇒ -3[cos2x - 4cosx] – p = 0
⇒ -3[cos2x - 4cosx + 4] + 12 – p = 0 [Adding and subtracting 12]
⇒ -3[cosx - 2]2 = p - 12
⇒ [cosx - 2]2 = -p/3 + 4
Now, 1 ≥ cosx ≥ -1
∴ 9 ≥ (cosx - 2)2 ≥ 1
⇒ 9 ≥ -p/3 + 4 ≥ 1
⇒ 5 ≥ -p/3 ≥ -3
⇒ -15 ≤ p ≤ 9
Hence, option (c).
Workspace:
ABCD is a quadrilateral whose diagonals AC and BD intersect at O. If triangles AOB and COD have areas 4 and 9 respectively, then the minimum area that ABCD can have is
- A.
26
- B.
25
- C.
21
- D.
16
Answer: Option B
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
Area of triangle AOB = 4, DOC = 9, AOD = x and BOC = y
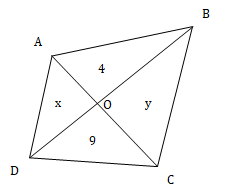
In a quadrilateral, product of area of diagonally opposite triangles is same.
∴ 4 × 9 = x × y
⇒ xy = 36
Area of quadrilateral = 4 + 9 + x + y
Now we need to minimise 4 + 9 + x + y
This is possible when x + y is least possible.
We know, AM ≥ GM
⇒ ≥
⇒ (x + y) ≥ 12
∴ Least possible value of x + y = 12
⇒ Least possible area of the quadrilateral = 4 + 9 + x + y = 25
Hence, option (b).
Workspace:
The highest possible value of the ratio of a four-digit number and the sum of its four digits is
- A.
1000
- B.
277.75
- C.
900.1
- D.
999
Answer: Option A
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
The highest possible value of the ratio of a four-digit number and the sum of its four digits is
Let the number be 'abcd' = 1000a + 100b + 10c + d
We have to find the highest possible value of
(1000a + 100b + 10c + d) : (a + b + c + d)
This is possible when a is highest and b, c and d are lowest
i.e., a = 9 and b = c = d = 0
∴ Highest possible ratio = 9000 : 9 = 1000
Hence, option (a).
Workspace:
Consider the polynomials f(x) = ax2 + bx + c, where a > 0, b, c are real, g(x) = -2x. If f(x) cuts the x-axis at (-2, 0) and g(x) passes through (a, b), then the minimum value of f(x) + 9a + 1 is
- A.
0
- B.
1
- C.
2
- D.
3
Answer: Option B
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
g(x) = -2x passes through (a, b)
⇒ b = -2a ...(1)
Now, f(x) = ax2 + bx + c
⇒ f(x) = ax2 - 2ax + c [from (1)]
f(x) passes through (2, 0)
⇒ a(-2)2 - 2a(-2) + c = 0
⇒ 8a + c = 0
⇒ c = -8a
∴ f(x) = ax2 - 2ax - 8a
Now, we have to find the least value of f(x) + 9a + 1
= ax2 - 2ax - 8a + 9a + 1
= ax2 - 2ax + a + 1
= a(x2 - 2x + 1) + 1
= a(x - 1)2 + 1
Least value of this expression will be 1, when x = 1.
Hence, option (b).
Workspace:
In a city, 50% of the population can speak in exactly one language among Hindi, English and Tamil, while 40% of the population can speak in at least two of these three languages. Moreover, the number of people who cannot speak in any of these three languages is twice the number of people who can speak in all these three languages. If 52% of the population can speak in Hindi and 25% of the population can speak exactly in one language among English and Tamil, then the percentage of the population who can speak in Hindi and in exactly one more language among English and Tamil is
- A.
22%
- B.
25%
- C.
30%
- D.
38%
Answer: Option A
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
Let the number of people who speak
Exactly 3 languages = a
Exactly 2 languages = b
Exactly 1 language = c
No language = d
⇒ a + b + c + d = 100 ...(1)
Given, c = 50 and a + b = 40 ...(2)
From (1) and (2) we get, d = 10 ...(3)
The number of people who cannot speak in any of these three languages is twice the number of people who can speak in all these three languages
⇒ d = 2a
∴ a = 5 [d = 10 from (3)]
Now, 52% of the population can speak in Hindi and 25% of the population can speak exactly in one language among English and Tamil. We can make the following Venn Diagram with the information so far.
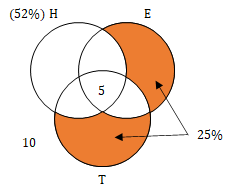
Total orange region = 25
Now, Number of people speaking exactly one language (c) = 50 = (Only Hindi speaking people) + (Only English speaking people) + (Only Tamil speaking people)
⇒ 50 = (Only Hindi speaking people) + 25
⇒ Only Hindi speaking people = 25
Also, Total Hindi speaking people = Only Hindi + (Hindi and exactly one other language) + (All three languages)
⇒ 52 = 25 + (Hindi and exactly one other language) + 5
⇒ Hindi and exactly one other language = 22
Hence, option (a).
Workspace:
A train left point A at 12 noon. Two hours later, another train started from point A in the same direction. It overtook the first train at 8 PM. It is known that the sum of the speeds of the two trains is 140 km/hr. Then, at what time would the second train overtake the first train, if instead the second train had started from point A in the same direction 5 hours after the first train? Assume that both the trains travel at constant speeds.
- A.
3 am the next day
- B.
4 am the next day
- C.
8 am the next day
- D.
11 pm the same day
Answer: Option C
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
Case 1: Train 2 starts 2 hours after Train 1.
Train 1 travels from 12 pm till 8 pm i.e., for 8 hours.
Same distance is travelled by Train 2 from 2 pm till 8 pm i.e., in 6 hours.
∴ Ratio of speeds of Train 1 and Train 2 = 6 : 8 = 3 : 4
⇒ Let speed of Train 1 = 3x and Train 2 = 4x
⇒ 3x + 4x = 140
⇒ x = 20
⇒ Speed of Train 1 = 60 kmph, Speed of Train 2 = 80 kmph.
Case 2: Train 2 starts 5 hours after Train 1.
Train 1 would have travelled 5 × 60 = 300 kms when Train 2 starts i.e., at 5 pm.
Relative speed of the two trains = 80 - 60 = 20 kmph.
∴ Time for Train 2 after it starts to meet Train 1 = 300/20 = 15 hours.
⇒ 15 hours after 5 pm = 8 am the next day.
Hence, option (c).
Workspace:
The number of 5-digit numbers consisting of distinct digits that can be formed such that only odd digits occur at odd places is
- A.
5250
- B.
6240
- C.
2520
- D.
3360
Answer: Option C
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
Let the five digit number be 'abcde'
All digits are distinct and digits a, c and e must be odd
There are 5 odd digits i.e., 1, 3, 5, 7 and 9.
a can take any of these 5 digits in 5 ways.
c can take any of the remaining 4 digits in 4 ways.
e can take any of the remaining 3 digits in 3 ways.
∴ Number of ways of choosing values for a, c and e = 5 × 4 × 3 = 60 ways.
b and d can take distinct values out of 0, 2, 4, 6, 8 and remaining 2 odd digits in 7 × 6 = 42 ways.
∴ Total number of ways of forming the requried 5 digit number = 60 × 42 = 2520 ways.
Hence, option (c).
Workspace:
There are 10 points in the plane, of which 5 points are collinear and no three among the remaining are collinear. Then the number of distinct straight lines that can be formed out of these 10 points is
- A.
10
- B.
25
- C.
35
- D.
36
Answer: Option D
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
Total number of lines that can be formed using 10 points = 10C2 = 45 lines.
But out of these 10, 5 points are collinear and hence will give only 1 line instead of 10C2 = 10.
∴ Number of unique lines = 45 - 10 + 1 = 36.
Hence, option (d).
Workspace:
The x-intercept of the line that passes through the intersection of the lines x + 2y = 4 and 2x + 3y = 6, and is perpendicular to the line 3x – y = 2 is
- A.
2
- B.
0.5
- C.
4
- D.
6
Answer: Option D
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
The intersection of the lines x + 2y = 4 and 2x + 3y = 6 is (0, 2)
Slope of line 3x – y = 2 is 3. Slope of line perpendicular to this line is -1/3 [Product of slopes of two perpendicular lines = -1]
Now, we need to find the x-intercept of a line passing through (0, 2) whose slope is -1/3
Let the x-intercept be (a, 0)
⇒ Slope of the line passing through (a, 0) and (0, 2) = - 1/3
⇒ =
⇒ a = 6
Hence, option (d).
Workspace:
Answer the following questions based on the information given below:
In a football tournament six teams A, B, C, D, E, and F participated. Every pair of teams had exactly one match among them. For any team, a win fetches 2 points, a draw fetches 1 point, and a loss fetches no points. Both teams E and F ended with less than 5 points. At the end of the tournament points table is as follows (some of the entries are not shown):

It is known that:
(1) team B defeated team C, and
(2) team C defeated team D
Total number of matches ending in draw is
- A.
12
- B.
4
- C.
5
- D.
6
Answer: Option D
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
Total number of matches played = 6C2 = 15
Each team plays 5 matches, one against each of the remaining 5 teams.
Points distributed in a match = 2 (2 + 0 or 1 + 1)
Total points to be distributed in the tournament = 2 × 15 = 30 points.
So far points scored by A, B, C and D = 8 + 6 + 5 + 5 = 24
∴ Points scored by E and F together = 30 – 24 = 6.
E: E lost only 1 match. The minimum number of points E can score is when it draws its remaining matches i.e., 4 points. Since E and F scored less than 5 points hence, E’s score is 4 point when it draws its remaining 4 matches.
A: A did not lose any game, hence it can score 8 points only by winning 3 matches and drawing 2 matches.
B: B lost 2 matches, hence it can score 6 points only by winning the remaining 3 matches.
C: C lost 2 games, hence it can score 5 points only by winning 2 matches and drawing 1 match.
D: D lost only 1 game, hence it can score 5 points by drawing 3 matches and winning 1 match.
So far A, B, C, D and E together have won 9 matches and lost only 6 matches. But the number of wins should be equal to number of loses.
∴ F must have lost 3 matches more than it wins.
F: Since E’s score is 4, F’s score = 6 – 4 = 2 points. F lost 3 matches more than it won. This is possible in 2 cases.
Case (a): F won 1 match and lost 4 matches and 0 draws.
Since E has to play 4 draws, E will play a draw match against F. [E cannot play drawn against B. B has 0 draws.]
Hence, F cannot have 0 draws.
∴ This case is rejected.
Case (b): F won 0 match and lost 3 matches and drew 2 matches.
This is the only possible case.
We can make the following table with the information available.

Sum of the draws column = 12 hence there are total 6 drawn matches. [Each draw results in entry of draw for 2 teams.]
Hence, option (d).
Workspace:
Which team has the highest number of draws
- A.
A
- B.
C
- C.
D
- D.
E
Answer: Option D
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
Consider the solution to first question of this set.
E plays the highest number of draws i.e., 4 draws.
Hence, option (d).
Workspace:
Total points Team F scored was
- A.
0
- B.
1
- C.
2
- D.
3
Answer: Option C
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
Consider the solution to first question of this set.
F scored 2 points.
Hence, option (c).
Workspace:
Which team was not defeated by team A
- A.
B
- B.
C
- C.
D
- D.
F
Answer: Option C
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
Consider the solution to first question of this set.
D lost only 1 match to C, hence A cannot defeat D.
Hence, option (c).
Workspace:
Team E was defeated by
- A.
Teams A and B only
- B.
Only Team A
- C.
Only Team B
- D.
Teams A, B and D only
Answer: Option C
Get your concepts cleared through our Self-Study Courses.
Join our Telegram Channel for CAT/MBA Preparation.
Explanation :
Consider the solution to first question of this set.
E played 4 draws and lost 1 match. Since B didn't play any draw, the match between B and E must end in a result. Hence, B must have defeated E.
Hence, option (c).
Workspace:
Feedback
Help us build a Free and Comprehensive Preparation portal for various competitive exams by providing us your valuable feedback about Apti4All and how it can be improved.
 Solution
Solution Discuss
Discuss Report
Report