XAT 2024 QADI | Previous Year XAT Paper
In a small college, students are allowed to take only one specialization. Traditionally, only two specializations are offered: Science and Arts. Students enrolled to specialize in Science must take Physics and Mathematics subjects, while students enrolled to specialize in Arts must take Economics and Political Science subjects. Students enrolled in Science are not allowed to take either Economics or Political Science, while students enrolled in Arts are not allowed to take either Physics or Mathematics.
Recently, the college has started a third specialization called MatEco that requires students to take Economics and Mathematics. However, MatEco students would not be allowed to take either Physics or Political Science. When the college opens this new specialization for enrolment, it allows students, originally enrolled in Science or Arts, to switch to MatEco. From among the students originally enrolled in Arts, 20 students switch to MatEco. This makes the number of Science students twice the number of Arts students. After this, from among the students who originally enrolled in Science, 45 students switch to MatEco. This makes the number of Arts students twice the number of Science students.
In total, how many students, from among those originally enrolled in Science or Arts, are now taking Economics?
- A.
45
- B.
65
- C.
80
- D.
95
- E.
None of the remaining options is correct.
Answer: Option D
Explanation :
Workspace:
In a school, the number of students in each class, from Class I to X, in that order, are in an arithmetic progression. The total number of students from Class I to V is twice the total number of students from Class VI to X. If the total number of students from Class I to IV is 462, how many students are there in Class VI?
- A.
93
- B.
88
- C.
83
- D.
77
- E.
None of the remaining options is correct
Answer: Option D
Explanation :
Workspace:
A flight, traveling to a destination 11,200 kms away, was supposed to take off at 6:30 AM. Due to bad weather, the departure of the flight got delayed by three hours. The pilot increased the average speed of the airplane by 100 km/hr from the initially planned average speed, to reduce the overall delay to one hour. Had the pilot increased the average speed by 350 km/hr from the initially planned average speed, when would have the flight reached its destination?
- A.
11:30 PM
- B.
7:50 PM
- C.
5:10 PM
- D.
8:10 PM
- E.
10:36 PM
Answer: Option D
Explanation :
Workspace:
Consider the equation , where x is a real number log5(x - 2) = 2log25(2x - 4).
For how many different values of x does the given equation hold?
- A.
0
- B.
1
- C.
2
- D.
4
- E.
Infinitely many
Answer: Option A
Explanation :
Given, log5(x - 2) = 2log25(2x - 4).
⇒ log5(x - 2) = 2log52(2x - 4).
⇒ log5(x - 2) = 2/2 × log5(2x - 4).
⇒ log5(x - 2) = log5(2x - 4).
⇒ x - 2 = 2x - 4
⇒ x = 2
Now, for x = 2, log5(x - 2) will not be defined. Hence, x = 2 cannot be the solution
∴ We get no solution for the given equation.
Hence, option (a).
Workspace:
The cost of running a movie theatre is Rs. 10,000 per day, plus additional Rs. 5000 per show. The theatre has 200 seats. A new movie released on Friday. There were three shows, where the ticket price was Rs. 250 each for the first two shows and Rs. 200 for the late-night show. For all shows together, total occupancy was 80%. What was the maximum amount of profit possible?
- A.
Rs. 1,20,000
- B.
Rs. 87,000
- C.
Rs. 95,000
- D.
Rs. 91,000
- E.
Rs. 1,16,000
Answer: Option D
Explanation :
There are 3 shows of 200 seats with 80% occupancy, hence 80% of 600 = 480 tickets were sold.
Profit will be maximum when maximum tickets are sold for first two shows (which have higher ticket price) and least tickets are sold for the night show (which has lower ticket price).
To maximise profit, we will assume first two shows sold 200 + 200 = 400 tickets while the late night show sold only 80 tickets
∴ Total revenue = 400 × 250 + 80 × 200 = 1,00,000 + 16,000 = 1,16,000
Also, total cost = 10000 + 3 × 5000 = 25,000
⇒ Maximum profit = 1,16,000 - 25,000 = 91,000
Hence, option (d).
Workspace:
FS food stall sells only chicken biryani. If FS fixes a selling price of Rs. 160 per plate, 300 plates of biriyani are sold. For each increase in the selling price by Rs. 10 per plate, 10 fewer plates are sold. Similarly, for each decrease in the selling price by Rs. 10 per plate, 10 more plates are sold. FS incurs a cost of Rs. 120 per plate of biriyani, and has decided that the selling price will never be less than the cost price. Moreover, due to capacity constraints, more than 400 plates cannot be produced in a day. If the selling price on any given day is the same for all the plates and can only be a multiple of Rs. 10, then what is the maximum profit that FS can achieve in a day?
- A.
Rs. 25,300
- B.
Rs. 28,900
- C.
Rs. 41,400
- D.
Rs. 52,900
- E.
None of the remaining options is correct.
Answer: Option B
Explanation :
The selling price of each plate will always be a multiple of 10. So, let the number of selling price of a plate be 160 + 10x.
∴ Number of plates sold will be 300 - 10x.
Total revenue = (300 - 10x) × (160 + 10x) = -100x2 + 1400x + 48000
Total cost = 120 × (300 - 10x)
⇒ Net profit = Total revenue - Total cost
= -100x2 + 1400x + 48000 - 120 × (300 - 10x)
= -100x2 + 1400x + 48000 - 36000 + 1200x
= -100x2 + 2600x + 12000
Now, net profit is a quadratic expression -100x2 + 2600x + 12000.
This will be maximum when x = - (2600/2 × -100) = 13
∴ Maximum profit = -100 × (13)2 + 2600 × (13) + 12000
= - 16900 + 33800 + 12000
= 28,900
Hence, option (b).
Workspace:
A farmer has a triangular plot of land. One side of the plot, henceforth called the base, is 300 feet long and the other two sides are equal. The perpendicular distance, from the corner of the plot, where the two equal sides meet, to the base, is 200 feet. To counter the adverse effect of climate change, the farmer wants to dig a circular pond. He plans that half of the circular area will be inside the triangular plot and the other half will be outside, which he will purchase at the market rate from his neighbour. The diameter of the circular plot is entirely contained in the base and the circumference of the pond touches the two equal sides of the triangle from inside.
If the market rate per square feet of land is Rs. 1400, how much does the farmer must pay to buy the land from his neighbour for the pond? (Choose the closest option.)
- A.
Rs. 3,16,80,000
- B.
Rs. 4,25,60,000
- C.
Rs. 6,33,60,000
- D.
Rs. 7,42,80,000
- E.
Rs. 2,98,20,000
Answer: Option A
Explanation :
Workspace:
A group of boys is practising football in a rectangular ground. Raju and Ratan are standing at the two opposite mid-points of the two shorter sides. Raju has the ball, who passes it to Rivu, who is standing somewhere on one of the longer sides. Rivu holds the ball for 3 seconds and passes it to Ratan. Ratan holds the ball for 2 seconds and passes it back to Raju. The path of the ball from Raju to Rivu makes a right angle with the path of the ball from Rivu to Ratan. The speed of the ball, whenever passed, is always 10 metre per second, and the ball always moves on straight lines along the ground.
Consider the following two additional pieces of information:
I. The dimension of the ground is 80 metres × 50 metres.
II. The area of the triangle formed by Raju, Rivu and Ratan is 1000 square metres.
Consider the problem of computing the following: how many seconds does it take for Raju to get the ball back since he passed it to Rivu?
- A.
I alone is sufficient to solve the problem.
- B.
II alone is sufficient to solve the problem.
- C.
Either of I or II, by itself, is sufficient to solve the problem.
- D.
I and II both are required to solve the problem.
- E.
The problem cannot be solved even with both I and II.
Answer: Option A
Explanation :
Workspace:
The least common multiple of a number and 990 is 6930. The greatest common divisor of that number and 550 is 110. What is the sum of the digits of the least possible value of that number?
- A.
6
- B.
9
- C.
14
- D.
18
- E.
None of the remaining options is correct
Answer: Option C
Explanation :
Workspace:
The roots of the polynomial P(x) = 2x3 - 11x2 + 17x + 6 are the radii of three concentric circles. The ratio of their area, when arranged from the largest to the smallest, is:
- A.
6 : 2 : 1
- B.
9 : 4 : 1
- C.
16 : 6 : 3
- D.
36 : 16 : 1
- E.
None of the remaining options is correct.
Answer: Option D
Explanation :
Workspace:
A local restaurant has 16 vegetarian items and 9 non-vegetarian items in their menu. Some items contain gluten, while the rest are gluten-free.
One evening, Rohit and his friends went to the restaurant. They planned to choose two different vegetarian items and three different non-vegetarian items from the entire menu. Later, Bela and her friends also went to the same restaurant: they planned to choose two different vegetarian items and one non-vegetarian item only from the gluten-free options. The number of item combinations that Rohit and his friends could choose from, given their plan, was 12 times the number of item combinations that Bela and her friends could choose from, given their plan.
How many menu items contain gluten?
- A.
1
- B.
2
- C.
3
- D.
4
- E.
5
Answer: Option B
Explanation :
Let the number of gluten free - vegetarian items be x and number of gluten free - non-vegetarian items be y.
∴ We have the following
Vegetarian items (16)
- Gluten free = x
- Gluten free = 16 - x
Non-Vegetarian items (9)
- Gluten free = y
- Gluten free = 9 - x
Rohit & his friends planned to choose two different vegetarian items and three different non-vegetarian
Number of ways of doing this = 16C2 × 9C3 = 120 × 84
Bela & his friends planned to choose two different vegetarian items and one non-vegetarian only from gluten-free options
Number of ways of doing this = xC2 × yC1 = x(x - 1)/2 × y
According to the question:
120 × 84 = 12 × x(x - 1)/2 × y
⇒ 120 × 84 = 6 × x(x - 1) × y
⇒ 20 × 84 = x(x - 1) × y
⇒ 20 × 84 = x(x - 1) × y
⇒ 1680 = x(x - 1) × y
Here, x ≤ 16 & y ≤ 9
Case 1: x = 16
⇒ 1680 = 16(16 - 1) × y
⇒ y = 7
Case 2: x = 15
⇒ 1680 = 15(15 - 1) × y
⇒ y = 8
Case 3: x = 14
⇒ 1680 = 14(13 - 1) × y
⇒ y = 9.23 (rejected)
We will note consider further cases for x, since y will be greater than 9, which is not possible.
∴ We have two cases for (x, y) = (16, 7) & (15, 8)
In both these cases, number of gluten free items = 16 + 7 or 15 + 8 = 23
∴ Out of total 16 + 9 = 25 items, 23 are definitely gluten free, hence 2 items will contain gluten.
Hence, option (b).
Workspace:
Consider the system of two linear equations as follows: 3x + 21y + p = 0; and qx + ry – 7 = 0, where p, q, and r are real numbers.
Which of the following statements DEFINITELY CONTRADICTS the fact that the lines represented by the two equations are coinciding?
- A.
p and q must have opposite signs
- B.
The smallest among p, q, and r is r
- C.
The largest among p, q, and r is q
- D.
r and q must have same signs
- E.
p cannot be 0
Answer: Option C
Explanation :
Workspace:
Consider a 4-digit number of the form abbb, i.e., the first digit is a (a > 0) and the last three digits are all b. Which of the following conditions is both NECESSARY and SUFFICIENT to ensure that the 4-digit number is divisible by a?
- A.
b is divisible by a
- B.
b is equal to 0
- C.
21b is divisible by a
- D.
9b is divisible by a
- E.
3b is divisible by a
Answer: Option E
Explanation :
Workspace:
Consider a right-angled triangle ABC, right angled at B. Two circles, each of radius r, are drawn inside the triangle in such a way that one of them touches AB and BC, while the other one touches AC and BC. The two circles also touch each other (see the image below).
If AB = 18 cm and BC = 24 cm, then find the value of r.

- A.
3 cm
- B.
4 cm
- C.
3.5 cm
- D.
4.5 cm
- E.
None of the remaining options is correct.
Answer: Option B
Explanation :
Workspace:
A king has distributed all his rare jewels in three boxes. The first box contains 1/3 of the rare jewels, while the second box contains k/5 of the rare jewels, for some positive integer value of k. The third box contains 66 rare jewels. How many rare jewels does the king have?
- A.
990
- B.
660
- C.
240
- D.
1080
- E.
Cannot be determined uniquely from the given information.
Answer: Option A
Explanation :
Workspace:
Read the following scenario and answer the TWO questions that follow.
Aman has come to the market with Rs. 100. If he buys 5 kilograms of cabbage and 4 kilograms of potato, he will have Rs. 20 left; or else, if he buys 4 kilograms of cabbage and 5 kilograms of onion, he will have Rs. 7 left. The per kilogram prices of cabbage, onion and potato are positive integers (in rupees), and any type of these vegetables can only be purchased in positive integer kilogram, or none at all.
Aman decides to buy only onion, in whatever maximum quantity possible (in positive integer kilogram), with the money he has come to the market with.
How much money will he be left with after the purchase?
- A.
Rs. 12
- B.
Rs. 9
- C.
Rs. 7
- D.
Rs. 5
- E.
Re. 1
Answer: Option E
Explanation :
Workspace:
Aman decides to buy only onion and potato, both in positive integer kilogram, in such a way that the money left with him after the purchase will be insufficient to buy a full kilogram of either of the two vegetables.
If all such permissible combinations of purchases are equally likely, what is the probability that Aman buys more onion than potato?
- A.
3/10
- B.
5/6
- C.
2/9
- D.
7/20
- E.
4/10
Answer: Option A
Explanation :
Workspace:
Read the following scenario and answer the TWO questions that follow.
41 applicants have been shortlisted for interviews for some data analyst positions. Some of the applicants have advanced expertise in one or more fields among the following: data analysis, database handling and coding. The numbers of applicants with different advanced expertise are given in the 2 × 8 table below.
The number of applicants with advanced expertise in all three fields is given as x in the table, where x is a non-negative integer.
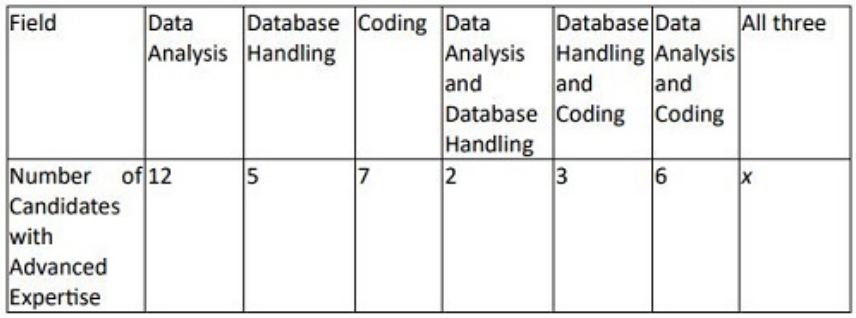
What BEST can be concluded about the value of x?
- A.
0, 1 and 2
- B.
2 only
- C.
1 only
- D.
0 or 1 only
- E.
1 or 2 only
Answer: Option B
Explanation :
Workspace:
How many applicants DID NOT have advanced expertise in any of the three given fields?
- A.
Cannot be determined uniquely from the given information.
- B.
25
- C.
26
- D.
27
- E.
28
Answer: Option C
Explanation :
Workspace:
Read the following scenario and answer the THREE questions that follow.
The upper hinge of a dataset is the median of all the values to the right of the median of the dataset in an ascending arrangement, while the lower hinge of the dataset is the median of all the values to the left of the median of the dataset in the same arrangement. For example, consider the dataset 4, 3, 2, 6, 4, 2, 7. When arranged in the ascending order, it becomes 2, 2, 3, 4, 4, 6, 7. The median is 4 (the bold value), and hence the upper hinge is the median of 4, 6, 7, i.e., 6. Similarly, the lower hinge is 2.
A student has surveyed thirteen of her teachers, and recorded their work experience (in integer years). Two of the values recorded by the student got smudged, and she cannot recall those values. All she remembers is that those two values were unequal, so let us write them as A and B, where A < B. The remaining eleven values, as recorded, are: 5, 6, 7, 8, 12, 16, 19, 21, 21, 27, 29. Moreover, the student also remembers the following summary measures, calculated based on all the thirteen values:
Minimum: 2
Lower Hinge: 6.5
Median: 12
Upper Hinge: 21
Maximum: 29
Which of the following is a possible value of B?
- A.
2
- B.
6
- C.
8
- D.
13
- E.
29
Answer: Option C
Explanation :
The known observations are: 5, 6, 7, 8, 12, 16, 19, 21, 21, 27, 29
Median of all 13 observations is 12, hence there must be 6 values greather than or equal to 12 and 6 values less than or equal to 12.
We see that for known observations, we already have 6 values (16, 19, 21, 21, 27 and 29) which are greather than 12. Hence, both the unknown values i.e., A and B must be less than 12.
Also, the smallest of these observations is 2 (given) and we know A < B, hence A = 2.
The lowest 6 observations are 2, 5, 6, 7, 8 and B.
The lower hinge is 6.5. Hence, B should take a value such than 6 and 7 are the middle two numbers when arranged in ascending order.
This is possible when B is greater than or equal to 7 and less than or equal to 12.
∴ Possible values of B are 7, 8, 9, 10, 11 or 12.
Hence, option (c).
Workspace:
Based on the information recorded, which of the following can be the average work experience of the thirteen teachers?
- A.
12
- B.
12.5
- C.
13
- D.
13.5
- E.
14
Answer: Option E
Explanation :
Workspace:
While rechecking her original notes to re-enter the smudged values of A and B in the records, the student found that one of the eleven recorded work experience values that did not get smudged was recorded wrongly as half of its correct value. After re-entering the values of A and B, and correcting the wrongly recorded value, she recalculated all the summary measures. The recalculated average value was 15.
- A.
7
- B.
9
- C.
10
- D.
12
- E.
Cannot be determined from the given information.
Answer: Option C
Explanation :
Workspace:
Read the following scenario and answer the THREE questions that follow.
A T20 cricket match consists of two teams playing twenty overs each, numbered 1 to 20. The runs scored in any over is a non-negative integer. The run rate at the end of any over is the average runs scored up to and including that over, i.e., the run rate at the end of the k-th over is the average number of runs scored in overs numbered 1, 2, …, k, where 1 ≤ k ≤ 20, k a positive integer. The following table indicates the run rate of a team at the end of some of the overs during a T20 cricket match (correct up to 2 decimal places), where 1 ≤ N – 2 < N + 6 ≤ 20, N a positive integer. It is also known that the team did not score less than 6 runs and more than 15 runs in any over.
| Over Number | Run Rate |
| N - 2 | 8.00 |
| N | 7.43 |
| N + 2 | 8.11 |
| N + 4 | 8.45 |
| N + 6 | 8.08 |
What is the value of N?
- A.
7
- B.
13
- C.
14
- D.
9
- E.
12
Answer: Option A
Explanation :
Average runs scored till Nth over is 7.43.
∴ Total runs scored in N overs = N × 7.43.
It would be best to check the options for the value of N such that N × 7.43 should be an integer.
This is possible for option (a) i.e., 7 and option (c) i.e., 14.
Now, the runrate at the end of N + 6 overs is 8.08. Checking for N = 7 or 14 such that (N + 6) × 8.08 should be an integer.
N = 7 is the only option satisfying.
⇒ N = 7
Runs scored after:
5th over: 5 × 8 = 40
7th over: 7 × 7.43 = 52
9th over: 9 × 8.11 = 73
11th over: 11 × 8.45 = 93
13th over: 13 × 8.08 = 105
Hence, option (a).
Workspace:
In which of these pairs of over numbers, the team could have scored 22 runs in total?
- A.
6 and 7
- B.
7 and 8
- C.
8 and 9
- D.
9 and 10
- E.
10 and 11
Answer: Option D
Explanation :
Consider the solution for first question of this set.
Runs scored after:
5th over: 5 × 8 = 40
7th over: 7 × 7.43 = 52
9th over: 9 × 8.11 = 73
11th over: 11 × 8.45 = 93
13th over: 13 × 8.08 = 105
Option (a): In 6th and 7th overs total 52 - 40 = 12 runs are scored. Hence, option (a) is rejected.
Option (b): Minimum runs scored till 6th over 40 + 6 = 46
Maximum runs scored before 9th over 73 - 6 = 67
∴ Maximum runs scored in 7th and 8th over = 67 - 46 = 21
Hence, option (b) is rejected.
Option (c): In 8th and 9th overs total 73 - 52 = 21 runs are scored. Hence, option (c) is rejected.
Option (d): Minimum runs scored till 8th over 52 + 6 = 58
Maximum runs scored before 11th over 93 - 6 = 87
∴ Maximum runs scored in 9th and 10th over = 87 - 58 = 29
Hence, option (d) is the right answer.
Option (e): In 10th and 11th overs total 93 - 73 = 20 runs are scored. Hence, option (e) is rejected.
Hence, option (d).
Workspace:
In which of the following over numbers, the team MUST have scored the least number of runs?
- A.
7
- B.
8
- C.
9
- D.
10
- E.
11
Answer: Option A
Explanation :
Consider the solution for first question of this set.
Runs scored after:
5th over: 5 × 8 = 40
7th over: 7 × 7.43 = 52
9th over: 9 × 8.11 = 73
11th over: 11 × 8.45 = 93
13th over: 13 × 8.08 = 105
In 6th and 7th overs total 52 - 40 = 12 runs are scored.
Since minimum runs scored in any over is 6, hence in both 6th and 7th overs, 6 runs MUST have been scored.
Hence, option (a).
Workspace:
Read the following scenario and answer the THREE questions that follow.
A store offers a choice of five different discount coupons to its customers, described as follows:
Coupon A: A flat discount of Rs. 250 on a minimum spend of Rs. 1200 in one transaction.
Coupon B: A 15% discount on a minimum spend of Rs. 500 in one transaction, up to a maximum discount of Rs. 300.
Coupon C: A flat discount of Rs. 100 on a minimum spend of Rs. 600 in one transaction.
Coupon D: A 10% discount on a minimum spend of Rs. 250 in one transaction, up to a maximum discount of Rs. 100.
Coupon E: A flat discount of Rs. 50 on a minimum spend of Rs. 200 in one transaction.
The customers are allowed to use at most one coupon in one transaction, i.e., two or more coupons cannot be combined for the same transaction.
Four customers used four different discount coupons for their respective transactions in such a way that they obtained a total discount of Rs. 710.
- A.
Coupon A
- B.
Coupon B
- C.
Coupon C
- D.
Coupon D
- E.
Coupon E
Answer: Option E
Explanation :
Workspace:
Four customers used four different discount coupons for their respective transactions in such a way that nobody used any discount coupon sub-optimally. (A discount coupon is used suboptimally if using another discount coupon could have resulted in a higher discount for the same transaction.)
- A.
Rs. 2250
- B.
Rs. 2500
- C.
Rs. 2350
- D.
Rs. 2300
- E.
Rs. 1550
Answer: Option B
Explanation :
Workspace:
A family wanted to purchase four products worth Rs. 1000 each, and another product worth Rs. 300.
They were told that they could:
I) pay for the five products through one or more transactions in any way they wanted, as long as the purchase amount of any one product would not get split into different transactions, and
II) use the same discount coupon repeatedly for separate transactions, if they opt for more than one transaction.
What was the maximum discount that they could obtain for their purchase?
- A.
Rs. 600
- B.
Rs. 645
- C.
Rs. 650
- D.
Rs. 700
- E.
None of the remaining option is correct.
Answer: Option D
Explanation :
Workspace:
Feedback
Help us build a Free and Comprehensive Preparation portal for various competitive exams by providing us your valuable feedback about Apti4All and how it can be improved.
 Solution
Solution Discuss
Discuss Report
Report