IPMAT (I) 2022 QASA | Previous Year IPMAT - Indore Paper

Answer: 72

Explanation :

Given, ${\log }_{x^2}\left(y\right)$ + ${\log }_{y^2}\left(x\right)$ = 1

⇒ $\frac{{\log }_x\left(y\right)}{2}$ + $\frac{{\log }_y\left(x\right)}{2}$ = 1

⇒ log_xy + log_yx = 2

Now, log_xy and log_yx are reciprocal of each other and their sum is 2. Sum of a number and its reciprocal is always greater than or equal to 2. Equality is true when the number and reciprocal are both equal to 1.

∴ log_xy = 1
⇒ x = y

Now, y = x² - 30 [y = x]
⇒ x² - x - 30 = 0
⇒ (x - 6)(x + 5) = 0
⇒ x = 6 [x = -5 is rejected as log is not defined for negative numbers.]
∴ y = 6

∴ x² + y² = 6² + 6² = 72

Hence, 72.

Answer: 12

Explanation :

Consider, 2|x| + 3|y| = 6

Case 1: x > 0 and y > 0 [Quandrant I]
⇒ 2x + 3y = 6

Case 2: x < 0 and y > 0 [Quandrant II]
⇒ - 2x + 3y = 6

Case 3: x < 0 and y < 0 [Quandrant III]
⇒ - 2x - 3y = 6

Case 4: x > 0 and y < 0 [Quandrant IV]
⇒ 2x - 3y = 6

Each of these 4 lines can be drawn in their respective quadrants as shown in the figure.

​​​​​​​

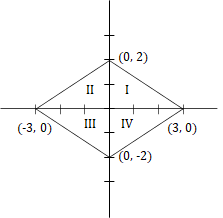
We need the area of region bounded by these 4 lines.

Area of region I = 1/2 × 3 × 2 = 3 sq. units

Similarly area of region II, III and IV = 3 sq. units each.

∴ Area of all the 4 regions combined = 3 + 3 + 3 + 3 = 12 sq. units

Hence, 12..

Answer: 30

Explanation :

Let the times taken at 12 kmph is t hours, hence the time taken at 20 kmph is (t - 1) hours.

⇒ Distance = 12 × t = 20 × (t - 1)
⇒ 12t = 20t - 20
⇒ t = 2.5 hours.

∴ Distance = 12 × 2.5 = 30 kms.

Hence, 30.

Answer: 175

Explanation :

7 points lie on a line and 5 points on another parallel line.

A triangle can be formed by 2 points on one of these lines and third point on the other line.

Case 1: 2 points are chose on line with 7 points and 3rd point is chosen on line with 5 points.
⇒ Number of such triangles = ⁷C₂ × ⁵C₁ = 21 × 5 = 105

Case 2: 1 point is chose on line with 7 points and 2 points are chosen on line with 5 points.
⇒ Number of such triangles = ⁷C₁ × ⁵C₂ = 7 × 10 = 70

∴ Total number of traingles = 105 + 70 = 175.

Hence, 175.

Answer: 50

Explanation :

Let Aruna purchase x apples at 20 each and y mangoes at 25 each.
Total cost = 20x + 25y

Case 1:
Total selling price of apples = x × 20 × 1.1 = 22x
Total selling price of mangoes = y × 25 × 0.8 = 20y
Since Aruna had no profit or loss, total cost price = total selling price
∴ 20x + 25y = 22x + 20y
⇒ 5y = 2x
⇒ x : y = 5 : 2
∴ Let x = 5q and y = 2q   ...(1)

Case 2: 
Total selling price of apples = x × 20 × 0.8 = 16x
Total selling price of mangoes = y × 25 × 1.1 = 27.5y
Now Aruna incurred a loss of Rs. 150
∴ 20x + 25y - 150 = 16x + 27.5y
⇒ 4x - 150 = 2.5y
⇒ 20q - 150 = 5q
⇒ q = 10

∴ Number of apples bought by Aruna = x = 5q = 50.

Hence, 50.

Answer: 26

Explanation :

Given, f(x) = |x| + 2|x−1| + |x−2| + |x−4| + |x−6| + 2|x−10|

f(x) can be written as sum of h(x) and g(x), where
h(x) = |x| + |x−1| + |x−2| + |x−4| + |x−6| + |x−10| and
g(x) = |x−1| + |x−10|

For h(x) critical points are 0, 1, 2, 4, 6 and 10.
∴ h(x) will be least when x is between 2 and 4.

For g(x) critical points are 1 and 10.
∴ g(x) will be least when x is between 1 and 10.

∴ Both h(x) and g(x) will be least when x is between 2 and 4.

Let us take x = 3.

Least value of h(x) = 3 + 2 + 1 + 1 + 3 + 7 = 17
Least value of g(x) = 2 + 7 = 9

⇒ Least value of f(x) = 17 + 9 = 26.

Hence, 26.

Answer: 32

Explanation :

Given, A = $\left[\begin{array}{ccc}1& 0& 0\\ 0& 0& 1\\ 0& 1& 0\end{array}\right]$ 

Now, A × A = $\left[\begin{array}{ccc}1& 0& 0\\ 0& 0& 1\\ 0& 1& 0\end{array}\right]$ × $\left[\begin{array}{ccc}1& 0& 0\\ 0& 0& 1\\ 0& 1& 0\end{array}\right]$ = $\left[\begin{array}{ccc}1\times 1+0\times 0+0\times 0& 1\times 0+0\times 0+0\times 1& 1\times 0+0\times 1+0\times 0\\ 0\times 1+0\times 0+1\times 0& 0\times 0+0\times 0+1\times 1& 0\times 0+0\times 1+1\times 0\\ 0\times 1+1\times 0+0\times 0& 0\times 0+1\times 0+0\times 1& 0\times 0+1\times 1+0\times 0\end{array}\right]$ = $\left[\begin{array}{ccc}1& 0& 0\\ 0& 1& 0\\ 0& 0& 1\end{array}\right]$ = I

⇒ A³ = A² × A = I × A = A

⇒ A⁶ = A³ × A³ = A × A = I

⇒ A⁹ = A³ × A³ × A³ = A × A × A = A² × A = I × A = A

Now, (A⁹ + A⁶ + A³ + A)

= A + I + A + A + A

= 3A + I

= $\left[\begin{array}{ccc}3& 0& 0\\ 0& 0& 3\\ 0& 3& 0\end{array}\right]$ + $\left[\begin{array}{ccc}1& 0& 0\\ 0& 1& 0\\ 0& 0& 1\end{array}\right]$

= $\left[\begin{array}{ccc}4& 0& 0\\ 0& 1& 3\\ 0& 3& 1\end{array}\right]$

∴ The absolute value of the determinant of (A⁹ + A⁶ + A³ + A) = absolute value of $\left[\begin{array}{ccc}4& 0& 0\\ 0& 1& 3\\ 0& 3& 1\end{array}\right]$

= |4 × (1 × 1 - 3 × 3)| = |4 × (1 - 9)| = 32.

Hence, 32.

Answer: 256

Explanation :

Sum of the coefficients of all the terms in the expansion of (ax − b)ⁿ can be obtained by substituting x = 1.

∴ Sum of the coefficients of all the terms in the expansion of (5x − 9)⁴ = (5 - 9)⁴ = 4⁴ = 256.

Hence, 256.

Answer: 2067

Explanation :

2022^nd number in the sequence (including perfect squares) is 2022.

Now let us calculate number of perfect squares till 2022.

1², 2², 3², 4², 5², ..., 44² i.e., 44 numbers.

∴ 2022^nd number will be 2022 + 44 = 2066.

Here we need to check if there is any perfect square between 2022 and 2066.
2025 i.e., 45² is a perfect square between 2022 and 2066.

⇒ 2022^nd number in the given sequence after removing perfect squares will be 2067.

Hence, 2067.

Answer: 100

Explanation :

Sin α + Sin β = $\frac{\sqrt{2}}{\sqrt{3}}$   ...(1) and
Cos α + Cos β = $\frac{1}{\sqrt{3}}$   ...(2),

Squaring both the equations and adding them we get.

⇒ Sin² α + Sin² β + 2 × Sin α × Sin β + Cos² α + Cos² β + 2 × Cos α × Cos β = $\frac{2}{3}$ + $\frac{1}{3}$

⇒ Sin² α + Cos² α + Sin² β + Cos² β + 2 × Sin α × Sin β + 2 × Cos α × Cos β = 1

⇒ 1 + 1 + 2 × cos (α - β) = 1

⇒ cos (α - β) = - 1/2

⇒ 2 × ${\left(\cos \left(\frac{\alpha -\beta }{2}\right)\right)}^2$ - 1 = - 1/2

⇒ ${\left(\cos \left(\frac{\alpha -\beta }{2}\right)\right)}^2$ = 1/4

∴ 20² × ${\left(\cos \left(\frac{\alpha -\beta }{2}\right)\right)}^2$ = 400

⇒  ${\left(20 \cos \left(\frac{\alpha -\beta }{2}\right)\right)}^2$ = 100.

Hence, 100.

Answer: 344

Explanation :

Let the 3^rd term of AP = T₃ = a [Here, a is not the first term of the AP]

14^th term of AP = T₁₄ = ar and
69^th term of AP = T₆₉ = ar² 

Now, T₁₄ - T₃ = 11d [d is the common difference of the AP]
Also, T₆₉ - T₁₄ = 55d

⇒ 5(T₁₄ - T₃) = T₆₉ - T₁₄ 
⇒ 5(ar - a) = ar² - ar
⇒ r² - 6r + 5 = 0
⇒ (r - 1)(r - 5) = 0
⇒ r = 1 or 5
[r = 1 is rejected as the given terms are distinct.]

∴ T₃ = a
T₁₄ = 5a
T₆₉ = 25a

⇒ d = (5a - a)/11 = 4a/11

Now, next term of geometric progression will be 125a = T₃ + (n - 3)d
⇒ 125a = a + (n - 3) × 4a/11
⇒ 341 = n - 3
⇒ n = 344.

∴ The next term of the geometric progression is the 344^th term of the GP.

Hence, 344.

Answer: 16

Explanation :

P(A) is the power set of A.

Number of elements in power set of A = 2ⁿ where n is the number of elements in A.

Since A is a null set, number of elements in P(A) = 2⁰ = 1.

Power set of P(A) i.e., P(P(A)) will have 2¹ = 2 elements.

Power set of P(P(A)) i.e., P(P(P(A))) will have 2² = 4 elements.

Power set of P(P(P(A))) i.e., P(P(P(P(A)))) will have 2⁴ = 16 elements.

Hence, 16.

Answer: 3

Explanation :

−16, 2^x+3 − 2^2x−1 − 16, 2^2x−1 + 16 are in AP

If a, b and c are in AP ⇒ 2b = a + c

∴ 2 × (2^x+3 − 2^2x−1 − 16) = -16 + 2^2x−1 + 16

⇒ 2^x+4 − 2^2x − 32) = 2^2x−1

⇒ 16 × 2^x − 2^2x − 32 = 2^2x​​/2

⇒ 32 × 2^x − 2 × 2^2x − 64 = 2^2x​​

Take 2^x = a and 2^2x = a² 

⇒ 32a − 2a² − 64 = a²​​

⇒ 3a²​​ - 32a + 64 = 0

⇒ 3a²​​ - 8a - 24a + 64 = 0

⇒ (3a - 8)(a - 8) = 0

⇒ a = 8/3 or 8

⇒ 2^x = 8/3 or 8 

[8/3 is rejected as 2^x cannot be a fraction]

⇒ 2^x = 8 = 2³ 

⇒ x = 3 

Hence, 3.

Answer: 960

Explanation :

Let us form a group X of Mr. and Mr. Ahuja. They can be arranged in 2! = 2 ways.

Now, X and 4 other persons can be seated around a circle in 4! = 24 ways.

Now we have total 5 people (X is counted as 1 only, no one should sit between Ahujaas) and there are 5 places between these 5 people for Mr. and Mrs. Sharma to sit.

We need to select 2 of these 5 places for Mr. and Mrs. Sharma. This can be done in ⁵C₂ = 10 ways.
Mr. and Mrs. Sharam can sit in these 2 places in 2! = 2 ways.

∴ Total number of ways = 2 × 24 × 10 × 2 = 960.

Hence, 960.

Answer: 3150

Explanation :

To calculate the maximum sum of 50 integers, we can assume the largest 25 integers as 100, 99, 98, ..., 76.

Average of these 25 integers = (100 + 76)/2 = 88

The average of remaining 25 integers must be 88 - 50 = 38

∴ Maximum sum of all 50 integers = 25 × 88 + 25 × 38 = 25 × 126 = 3150

Hence, 3150.